TS Inter 2nd Year Physics Study Material Chapter 13 Atoms

Telangana TSBIE TS Inter 2nd Year Physics Study Material 13th Lesson Atoms Textbook Questions and Answers.

TS Inter 2nd Year Physics Study Material 13th Lesson Atoms

Very Short Answer Type Questions

Question 1.
What is the angular momentum of electron in the second orbit of Bohr’s model of hydrogen atom?
Answer:
Angular momentum L = \(\frac{nh}{2 \pi}\)
For 2nd orbit ⇒ n = 2.
∴ Angular momentum of 2nd orbit
L₂ = \(\frac{2h}{2 \pi}=\frac{h}{\pi}\)

Question 2.
What is the expression for fine structure constant and what is its value?
Answer:
Fine structure constant a = \(\frac{2 \pi e^2}{ch}=\frac{1}{137}\)
= 7.30 × 10^-3.

Question 3.
What is the physical meaning of ‘negative energy of an electron’?
Answer:
Energy is always +ve. There is no negative energy. But negative energy of electron means it is the force of attraction with which an electron is bounded to the nucleus.

Question 4.
Sharp lines are present in the spectrum of a gas. What does this indicate?
Answer:
When gases are excited they will absorb the exact quanta of energy equals to energy level difference of the orbits.
i.e., E = hv = E_f – E_i

So electron goes to higher orbit. As a result we will see sharp lines in the spectrum of gases corresponding to that energy.

Question 5.
Name a physical quantity whose dimensions are the same as those of angular momentum.
Answer:
Angular momentum (L) = mvr,
Dimensions = ML²T^-1
Planck’s constant h has same dimensions of L.
∵ L = \(\frac{nh}{2 \pi}\), where \(\frac{n}{\pi}\) is dimensionless.

Question 6.
What is the difference between α-particle and helium atom?
Answer:
α – particle is helium nucleus. But not helium atom.

α – particle contains 2 protons and two neutrons where as helium atom contains 2 protons, two neutrons and two electrons.

Question 7.
How is impact parameter related to angle of scattering?
Answer:
When impact parameter is minimum head on collision between α – particle and nucleus takes place and α- particle will be deviated by θ = π radians, when impact parameter is high or large the α – particle goes nearly undeviated.

Question 8.
Among alpha, beta and gamma radiations, which get affected by the electric field?
Answer:
α and β particles are affected by electric fields because both of them carries charge on them.

γ – ray is radiation so it is not affected by electric field.

Question 9.
What do you understand by the phrase ‘ground state atom’?
Answer:
The lowest state of the atom which is the lowest energy, with the electron revolving in the orbit of smallest radius is called the ground state.
For ground state hydrogen atom E = – 13.6 eV,

Question 10.
Why does the mass of the nucleus not have any significance in scattering in Rutherford’s experiment?
Answer:
In Rutherford experiment gold foil is used. For gold Z = 79 and its atomic weight is nearly 50 times more than α – particle. So mass of nucleus used to bombard gold atom has no significant effect.

Question 11.
The Lyman series of hydrogen spectrum line in the ultraviolet region Why?
Answer:
For Lyman series \(\frac{1}{\lambda}\) = R(\(\frac{1}{l^2}-\frac{1}{n^2}\))
n = 2, 3, 4 ….
Wavelength of 1st member of Lyman series is 1216A°. It is in ultraviolet region.

Question 12.
Write down a table giving longest and shortest wavelengths of different spectral series.
Answer:

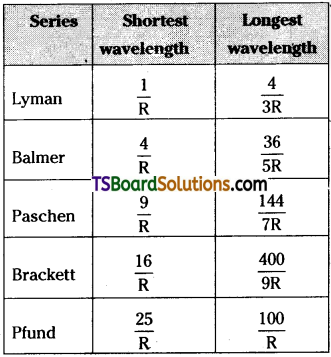
Question 13.
The wavelengths of some of the spectral lines obtained in hydrogen spectrum are 1216Å, 6463 Å and 9546Å. Which one of these wavelengths belongs to the Paschen series?
Answer:
λ = 9546Å belongs to Paschen series. Paschen series is in infrared region. So wavelength λ = 9546Å is in infrared region. So it belongs to Paschen series.

Question 14.
Give two drawbacks of Rutherford’s atomic model. [TS Mar. ’19]
Answer:

1. Electron revolving around the nucleus must be continuously accelerated. An accelerating electron must lose energy continuously due to radiation and finally, atom must destroy.
2. When electron radiates energy it will spiral around nucleus. As a result its angular velocity and frequency of spectral lines must change continuously. But these two things are not taking place.

Short Answer Questions

Question 1.
What is impact parameter and angle of scattering? How are they related to each other?
Answer:
Impact parameter :
It is the perpendicular distance of the initial velocity vector of α-particle from centre of nucleus.

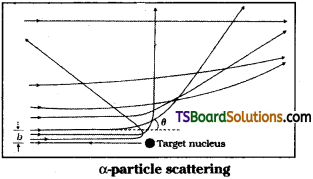
In case of head-on collision, impact parameter is minimum and α – particle rebounds back (θ = π). For a large impact parameter α – particle goes undeviated. The chance of head on collision is very small. It, in turn, suggested that mass of atom is much concentrated in a small volume.

Angle of scattering ‘0’ :
It is the angle between the direction of incident α – particle and scattered α – particle.

Relation between impact parameter and angle of scattering, when Impact parameter is less ⇒ angle of scattering is high and vice-versa.

Question 2.
Derive an expression for potential and kinetic energy of an electron in any orbit of a hydrogen atom according to Bohr’s atomic model. How does P.E. change with increasing n? [TS June 15; Mar. 15]
Answer:
According to Bohr model, electrons are revolving around the nucleus in certain permitted orbits.

For electron to revolve in orbit, the electrostatic force and centrifugal force must be equal i.e., F_c = F_e

∴ Kinetic energy of electron (K) = \(\frac{1}{2}\) mv²

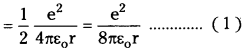
Potential energy between electron and nucleus is

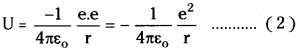
– ve sign indicates force of attraction. Relation between potential energy and radius of orbit.
From the equation (2) U ∝ \(\frac{1}{r}\) …………… (3)

From eq. (3) & (4) potential energy
U ∝ \(\frac{1}{n^2}\)
∴ Potential energy of orbit is inversely proportional to square of number of orbit (n²).

Question 3.
What are the limitations of Bohr’s theory of hydrogen atom? [TS May 18, 17; AP Mar. 17, 14, May 17]
Answer:
Limitations of Bohr model:
i) Bohr model is applicable to hydrogen atom only. It ean not be extended even to a two electron system such as helium.

Because it involves force between + vely charged nucleus and electron.

Electrical forces between electrons are not taken into account.

ii) It is not able to explain the intensity variation in spectral lines of different frequencies and why some transitions are more preferred than others.

Question 4.
Explain the distance of closest approach and impact parameter.
Answer:
Distance of closest approach :
In α- particle scattering experiment the α – particle will move near to gold nucleus until it is just stopped.

Kinetic energy of α – particle just before stopped is equal to electrostatic potential.

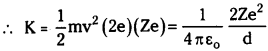
where = Z atomic number of gold, ’2e’ charge on α – particle.
distance of closest approach d = \(\frac{2 \mathrm{Ze}^2}{4 \pi \varepsilon_0 \mathrm{k}}\)

Impact parameter :
It is the perpendicular distance of the initial velocity vector of α – particle from centre of nucleus.

In case of head-on collision impact parameter is minimum and α – particle rebounds back (θ = π). For a large impact parameter α – particle goes undeviated. The chance of head on collision is very small. It in turn suggested that mass of atom is much concentrated in a small volume.

Question 5.
Give a brief account of Thomson model of atom. What are its limitations?
Answer:
J.J. Thomson Model :
J.J.Thomson thought that the positive charge of the atom is uniformly distributed through out the atom and the negatively charged electrons are embedded in it like seeds in a watermelon.

Limitations:

1. Rutherford α – particle scattering experiment showed that in an atom positive charge is concentrated at nucleus of atom.
2. In Thomson model the radiation emitted by solids and gases is due to oscillations of atoms and molecules are governed by the interactions between them.

But experiments on rarefied gases showed that hydrogen always gives rise to a set of lines with fixed wavelength.

Balmer experiments and his formula for Balmer series of wavelength of a group of lines are emitted by atomic hydrogen only. Not by interaction of atoms or molecules.

Question 6.
Describe Rutherford atom model. What are the drawbacks of this model? [AP & TS Mar. ’16]
Answer:
Rutherford’s nuclear model:

1. According to Rutherford the entire positive charge and most of the mass of the atom is concentrated at nucleus. Electrons are revolving around the nucleus as planets revolve around the sun.
2. Rutherford experiments on α – particle suggested that size of atom is about 10^-15 to 10^-14 and size of nucleus is about 10^-10 m.

Drawbacks :

1. When electron is revolving round the nucleus it is in continuously accelerated state. As per classical mechanics, it must emit energy continuously. So electron must follow spiral path instead of circular path. Finally electron must fall on nucleus i.e., + ve charge and atom will destroy which is not happening.
2. This model is not able to explain why electron in an orbit is not radiating any energy.

Question 7.
Distinguish between excitation potential and ionization potential.
Answer:
Excitation potential :

1. Generally gases or vapours are excited at low pressure by passing high current through them. For this we have to apply a very high voltage.
2. When excited atoms or molecules will absorb certain amount of energy from applied potential and gives rise to series of spectral lines called emission spectrum.

Ionisation potential :
It is the amount of minimum energy required to release an electron from the outer most orbit of the nucleus.

From Bohr’s model, energy of the orbit is the ionisation energy of electron in that orbit.
Ex: Energy of 1st orbit in hydrogen is 13.6 eV.

Practically ionisation potential of hydrogen is 13.6 eV.

Difference:
→ Excitation potential will permit electrons to transit between various energy levels whereas ionisation potential will liberate an electron from the influence of nucleus of that atom.

Question 8.
Explain the different types of spectral series of hydrogen atom.
(OR)
Write the different types of Hydrogen Spectral series. The Lyman series of Hydrogen spectrum lies in the ultraviolet region. [TS May 16, Mar. 16, AP Mar. 19, 15, May 18, 16, June 15]
Answer:
Lyman series :
When electrons are jumping from higher energy levels to the first orbit then that series of spectral lines emitted are called “Lyman series”.
In Lyman series, \(\frac{1}{\lambda}\) = R(\(\frac{1}{l^2}-\frac{1}{n^2}\))
where n = 2, 3, …………… etc.

These spectral lines are in ultraviolet region.

Balmer series :
When electrons are jumping the from higher levels to 2nd orbit then that series of spectral lines are called “Balmer series”.

For Balmer series, \(\frac{1}{\lambda}\) = R(\(\frac{1}{2^2}-\frac{1}{n^2}\))
where n = 3, 4, ………… etc.

Spectral lines of Balmer series are in visible region.

Paschen series :
When electrons are jumping on to the 3rd orbit from higher energy levels then that series of spectral lines are called “Paschen series”.
For Paschen series \(\frac{1}{\lambda}\) = R(\(\frac{1}{3^2}-\frac{1}{n^2}\))
where n = 4, 5, …………..

These spectral lines are in near infrared region.

Brackett series :
When electrons are jumping on to the 4th orbit from higher levels then that series of spectral lines are called “Brackett series”.
For Brackett series, \(\frac{1}{\lambda}\) = R(\(\frac{1}{4^2}-\frac{1}{n^2}\))
where n = 5, 6, ………… etc.

Brackett series are in middle infrared region.

Pfund series :
When electrons are jumping on to the 5th orbit from higher energy levels then that series of spectral lines are called “Pfund series”.
For Pfund series \(\frac{1}{\lambda}\) = R(\(\frac{1}{5^2}-\frac{1}{n^2}\))
n = 6, 7, ……….. etc.

These spectral lines are in far infrared region.

Reason for Lyman series in ultraviolet region :
The wavelength of Lyman series is nearly 1200 A° and less. So Lyman series is ultraviolet region.

Question 9.
Write a short note on de Broglie’s explanation of Bohr’s second postulate of quantization. [TS Mar. ’17]
Answer:
De – Broglie’s explanation for Bohr 2nd postulate :
According to de Broglie, the electron in an orbit must be seen as a particle wave. Particle waves can lead to standing waves under resonance condition.

A standing wave can be formed in a wire when length of the wire is equal to wave-length λ or integral multiple of wavelength λ (i.e., nλ). In the same way for an electron moving in a circular orbit, if its circum-ference 2πr_n is equal to nλ then standing waves will be formed in that orbit.
∴ From de-Broglie’s explanation 2πr_n = nλ
But λ = h/p = \(\frac{h}{mv_n}\)
∴ de Broglie wavelength 2πr_n = nh / mv_n
mv_nr_n = \(\frac{nh}{2 \pi}\)
Where mv_nr_n is angular momentum of electron in nth orbit.
In this way de – Broglie hypothesis provided an explanation to Bohr’s 2nd postulate.

Long Answer Questions

Question 1.
Describe Geiger – Marsden Experiment on scattering of α – particles. How is the size of the nucleus estimated in this experiment?
Answer:
Geiger – Marsden experiment :
Description :
In this experiment α – particles from ²¹⁴₈₃Bi are passed through lead blocks containing coaxial holes to emit narrow beam of α – particles. This α – particle beam falls on gold foil and scatters. The scattered α – particles are detected. By rotating the microscope at different angles (θ) number of scattered particles are measured.

Explanation :
In this experiment a beam of α – particles of energy 5.5 MeV are made to fall on gold foil.

α – particle carries 2 units of positive charge and mass is equal to that of helium nucleus.

→ Assume that the gold foil is very thin and α – particle will suffer not more than one scattering during its passage through gold foil.

For gold Z = 79. Its mass is nearly 50 times more than that of α – particle. So during collision it remains almost stationary.

Magnitude of force between α – particle and gold nuclei (F) = \(\frac{1}{4 \pi \varepsilon_{\mathrm{o}}} \frac{(2 \mathrm{e})(\mathrm{Ze})}{\mathrm{r}^2}\), ‘r’ = the distance between them.

The magnitude and direction of force changes continuously as it approaches the nucleus.

Impact parameter :
It is the perpendicular distance of the initial velocity vector of α – particle from centre of nucleus.

In case of head – on collision impact parameter is minimum and α – particle rebounds back (θ = π). For a large impact parameter α – particle goes undeviated. The chance of head on collision is very small.

Estimation of size of nucleus :
In this experiment a graph is plotted between number of scattered particles (n) and angle of scattering ‘θ’. It suggested that size of nucleus is about 10^-15 to 10^-14 m. From kinetic theory size of atom is about 10^-10 m. So size of atom is 10,000 to 100,000 times more than nucleus.

Question 2.
Discuss Bohr’s theory of the spectrum of hydrogen atom. [AP Mar. ’16]
Answer:
From Bohr’s model of hydrogen atom

1. Electrons are revolving in certain per-mitted non-radiating orbits around nucleus.
2. For non-radiating orbits, orbital angular momentum L = mvr = nh / 2π.
3. Electrons may jump from one orbit to another orbit. While doing so they will emit or absorb the energy equal to diffe-rence of those orbital energies.
   E = hν = E_i – E_f

When electrons are revolving in the orbit centrifugal force and centripetal force on it are equal F_c = F_e.

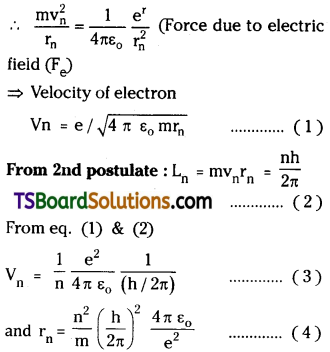
For hydrogen atom n = 1
∴ r₁ = h²ε₀/π me² ……………. (5)
This is also called Bohr radius
a₀ = 5.29 × 10^-11 m
Energy of the orbit E = K + U = Kinetic energy + Potential Energy of electron.
∴ E_n = –\(\frac{e^2}{8\pi \epsilon_0 r}\)
By using value of r1 from eq. 4.

From 3rd postulate :
when electrons are jumping from higher to lower orbit.

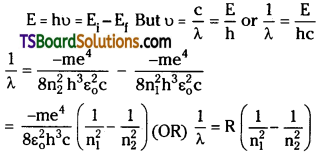
Where R = Rydberg’s constant. (∵ Electron is initially at orbit n₂ and finally at n₁)

In this way Bohr atom model successfully explained energy of the orbits and origin of spectral lines.

Question 3.
State the basic postulates of Bohr’s theory of atomic spectra. [AP Mar. ’16]
Hence obtain an expression for the radius of orbit and the energy of orbital electron in a hydrogen atom.
Answer:
Bohr’s postulates :
Bohr model of hydrogen atom consists of three main postulates.
i) Electrons in an atom could revolve in certain permitted stable orbits. Electrons revolving in these stable orbits do not emit or radiate any energy.

ii) The stable orbits are those whose orbital angular momentum is an integral multiple of h/2π.
i.e., L = nh / 2π where n = 1, 2, 3 etc. (an integer.)
These stable orbits are also called as non – radiating orbits.

iii) An electron may take a transition between non-radiating orbit.

when electron transition takes place a photon of energy equals to the energy difference between initial and final states will be radiated.
E = hν = E_i – E_f.

Bohr Model of Hydrogen atom :
When electrons are revolving in the orbit electrostatic force and centripetal force on it are equal F_c = F_e.

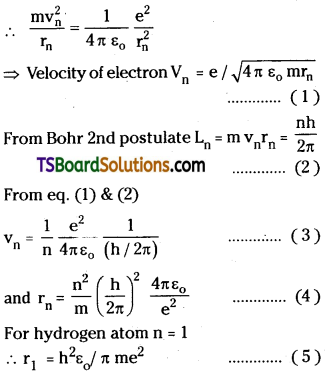
This is also called Bohr radius
a₀ = 5.29 × 10^-11 m
Energy of the orbit E = K + U = Kinetic energy + Potential Energy of electron.

Intext Question and Answer

Question 1.
The radius of the first electron orbit of a hydrogen atom is 5.3 × 10^-11m. What is the radius of the second orbit?
Answer:
Radius of first orbit r₁ = 5.3 × 10^-11m.
Radius of Bohr orbit r =
For 2nd orbit n = 2,
∴ r = n₂ a₀ =4 × 5.3 10^-11 = 2.12 × 10^-11 m.

Question 2.
Determine the radius of the first orbit of the hydrogen atom. What would be the velocity and frequency of the electron in the first orbit ? Given: h = 6.62 × 10^-34J s, m = 9.1 × 10^-31 kg, e = 1.6 × 10^-19 C, k = 9 × 10⁹ m²C.
Answer:
Given h = 6.62 × 10^-34 J,
Mass m = 9.1 × 10^-31 kg
Charge e = 1.6 × 10^-19 C;

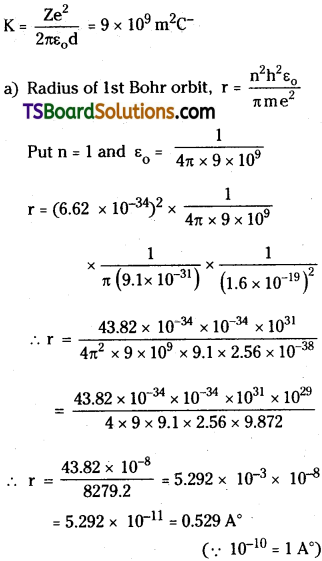
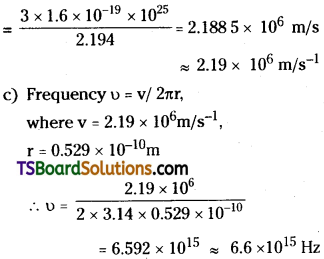
Question 3.
The total energy of an electron in the first excited state of the hydrogen atom is – 3.4 eV. What is the potential energy of the electron in this state?
Answer:
Total energy, T.E. = – 3.4 eV.
Potential energy U = 2 x T.E. = – 3.4 × 2
= – 6.8 eV in same orbit.

Question 4.
The total energy of an electron in the first excited state of the hydrogen atom is – 3.4 eV. What is the kinetic energy of the electron in this state?
Answer:
In 1st excited state
Total energy T.E. = – 3.4 eV.
Kinetic energy in 1st excited state = T.E. – U
Where U = 2 × T.E.
∴ K = U – 2U = – U = – (-3.4) = 3.4 eV.

Question 5.
Find the radius of the hydrogen atom in its ground state. Also calculate the velocity of the electron in n = 1 orbit. Given h = 6.63 × 10^-34 Js, m = 9.1 × 10^-31kg, e = 1.6 × 10^-19 C, k = 9 × 10⁹ N m²C^-2.
Answer:
Planck’s constant h = 6.63 × 10^-34 Js.;
Mass of electron m = 9.1 × 10^-31 Kg.
Charge on electron e = 1.6 × 10^-19 C;
K = 9 × 10⁹ m²C²
a) Radius of orbit, r \(\frac{n^2h^2\epsilon_0}{\pi me^2}\) where \(\frac{h^2\epsilon_0}{\pi me^2}\) = a₀ = 5.3 × 10^-11
For 1st orbit n = 1 ⇒ r = 1 × 5.3 × 10^-11
= 5.3 × 10^-11m

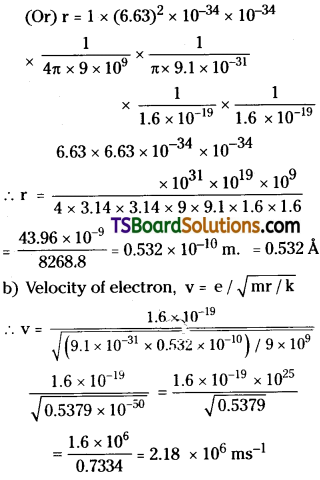
Question 6.
Prove that the ionization energy of hydrogen atom is 13.6 eV.
Answer:
From Bohr atom model energy radiated when electrons are jumping from one orbit
to another orbit is E = hυ = \(\frac{m e^4}{8 \varepsilon_0^2 h^2 n^2}\) = E₁ – E₂

By definition, ionization potential is the minimum amount of energy required to remove the electron from the orbit. Now for an electron of nearest orbit n₁ = 1 and n₂ = ∞ (because electron is free from influence of nucleus).

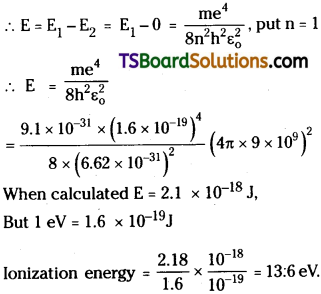
Question 7.
Calculate the Ionization energy fora lithium atom.
Answer:

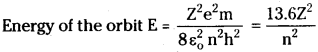
For lithium Z = 3 (But outer most orbit n = 2 in lithium)
∴ lonization energy = Energy of the orbit = \(\frac{13.6\times9}{4}\) = 30.6 eV.

Question 8.
The wavelength of the first member of Lyman series is 1216 Å. Calculate the wavelength of second member of Balmer series.
Answer:
Wavelength of 1st member of Lyman series = 1216 A°.

2nd member of Balmer series ⇒ \(\frac{1}{\lambda_2}\) = R(\(\frac{1}{2^2}-\frac{1}{4^2}\))
∵ For 2nd member of Balmer series n = 4.

Question 9.
The wavelength of first member of Balmer series is 6563 A. Calculate the wavelength of second member of Lyman series.
Answer:
Wavelength of 1 st member of Balmer series = 6563.

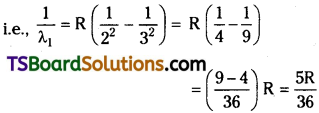
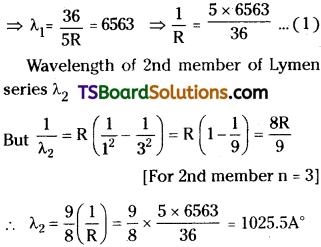
Question 10.
The second member of Lyman series in hydrogen spectrum has wavelength 5400 Å. Find the wavelength of first member.
Answer:
Wavelength of 2nd member of Lyman series λ = 5400

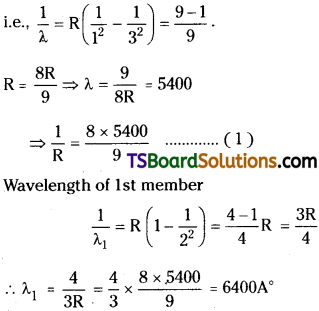
Question 11.
Calculate the shortest wavelength of Balmer series. Or Calculate the wavelength of the Balmer series limit.
Given : R = 10970000m^-1.
Answer:
Given Rydberg constant R = 10970000 m^-1
= 1.097 × 10⁷ m^-1
For Balmer series \(\frac{1}{\lambda_2}\) = R(\(\frac{1}{2^2}-\frac{1}{n^2}\))
Short wavelength ⇒ n₂ = ∞

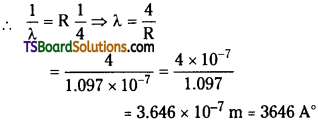
Question 12.
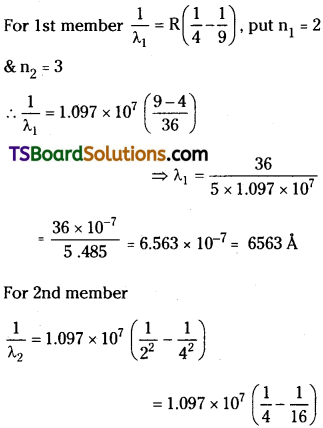
Using the Rydberg formula, calculate the wavelength of the first four spectral lines in the Balmer series of the hydrogen spectrum.
Answer:
For Balmer series \(\frac{1}{\lambda_2}\) = R(\(\frac{1}{2^2}-\frac{1}{n^2}\)) where
n = 2, 3, 4, 5 etc.
Rydberg constant R = 1.097 × 10⁷ m^-1


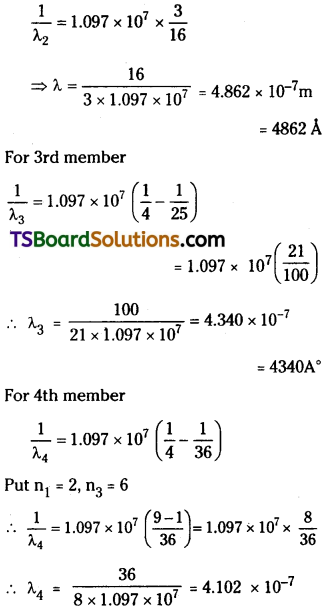
= 4102 A°