TS Inter Second Year Maths 2A Quadratic Expressions Important Questions Very Short Answer Type

Students must practice these Maths 2A Important Questions TS Inter Second Year Maths 2A Quadratic Expressions Important Questions Very Short Answer Type to help strengthen their preparations for exams.

TS Inter Second Year Maths 2A Quadratic Expressions Important Questions Very Short Answer Type

Question 1.
Find the roots of the quadratic equation ax² + bx + c = 0. [March ’02]
Solution:
Given quadratic equation is ax² + bx + c = 0
Now, multiplying with ‘4a’ on both sides
4a (ax² + bx – c) = 0
= 4ax²x² + 4abx + 4ac = 0
(2ax)² + 2 . 2ax . b + b² + b² – b² + 4ac = 0
(2ax + b)² = b² – 4ac
2ax + b = ± \(\sqrt{b^2-4 a c}\)
2ax = – b ± \(\sqrt{b^2-4 a c}\)
x = \(\frac{-b \pm \sqrt{b^2-4 a c}}{2 a}\)
∴ The roots of the quadratic equation, ax² + bx + c = 0 are \(\frac{-b \pm \sqrt{b^2-4 a c}}{2 a}\).

Question 2.
Fmd the roots of the following equation √3x² + 10x – 8√3 = 0.
Solution:
Given quadratic equation is √3x² + 10x – 8√3 = 0
Comparing this with ax² + bx + c = 0,
we have a = √3, b = 10 c = – 8√3
The roots of quadratic equation are \(\frac{-b \pm \sqrt{b^2-4 a c}}{2 a}\)
= \(\frac{-10 \pm \sqrt{100+96}}{2 \sqrt{3}}=\frac{-10 \pm \sqrt{196}}{2 \sqrt{3}}\)
= \(\frac{-10 \pm 14}{2 \sqrt{3}}=\frac{-10+14}{2 \sqrt{3}} \text { (or) } \frac{-10-14}{2 \sqrt{3}}\)
= \(\frac{4}{2 \sqrt{3}} \text { (or) } \frac{-24}{2 \sqrt{3}}=\frac{2}{\sqrt{3}} \text { (or) } \frac{-12}{\sqrt{3}}\)
= \(\frac{2}{\sqrt{3}}\) (or) – 4√3
∴ The roots of the quadratic equation are \(\frac{2}{\sqrt{3}}\) (or) – 4√3

Question 3.
Form a quadratic equation whose roots are 7 ± 2√5. [TS – May ’16; March ’11, ’05, AP – March 2018]
Solution:
Let α = 7 + 2√5, β = 7 – 2√5
Now, α + β = 7 + 2√5 + 7 – 2√5 = 14
αβ = (7 + 2√5) (7 – 2√5)
= 49 – 20 = 29
The quadratic equation whose roots are α, β is
x² – (α + β)x + αβ = 0
x² – 14x + 29 = 0.

Question 4.
Form a quadratic equation whose roots are – 3 ± 5i.
Solution:
Let α = – 3 + 5i, β = – 3 – 5i
Now, α + β = – 3 + 5i – 3 – 5i = – 6
αβ = (- 3 + 5i) (- 3 – 5i)
= 9 – 25i² = 34
The quadratic equation whose roots are α, β is x² – (α + β)x + αβ = 0
⇒ x² + 6x + 34 = 0

Question 5.
Form a quadratic equation whose roots are \(\frac{p-q}{p+q}\), – \(\frac{p+q}{p-q}\) (p ≠ q).
Solution:

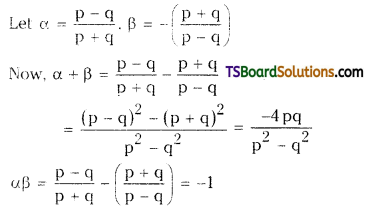
The quadratic equation whose roots are α, β is x² – (α + β)x + αβ = 0
x² + \(\frac{4 p q}{p^2-q^2}\) . x – 1 = 0
⇒ (p² – q²)x² + 4pqx – (p² – q²) = 0

Question 6.
Find the nature of the roots of 4x² – 20x + 25 = 0.
Solution:
Given quadratic equation is 4x² – 20x + 25 = 0.
Comparing this with ax² + bx + c = 0, we get
a = 4; b = – 20; c = 25
b² – 4ac = 400 – 4 . 4 . 25 = 400 – 400 = 0
Since ² – 4ac = 0 then the roots of the given equation are real and equal.

Question 7.
Find the nature of the roots of 2x² – 8x + 3 = 0.
Solution:
Given quadratic equation is 2x² – 8x + 3 = 0
Comparing this with ax² + bx + c = 0, we get
a = 2, b = – 8, c = 3
Now, b² – 4ac = 64 – 4 . 2 . 3
Since, b² – 4ac > 0 then the roots of the given equation are real and distinct.

Question 8.
Find the nature of the roots of 2x² – 7x + 10 = 0.
Solution:
Given quadratic equation is 2x² – 7x + 10 = 0
Comparing this with ax² + bx + c = 0, we get a = 2, b = – 7, c = 10
Now, b² – 4ac = 49 – 80 = – 31 < 0
Since, b² – 4ac < 0 then the roots of the given equation are conjugate complex numbers.

Question 9.
Find the nature of the roots of 3x² + 7x + 2 = 0.
Solution:
Given quadratic equation is 3x² + 7x + 2 = 0
Comparing this with ax² + bx + c = 0, we get a = 3, b = 7, c = 2
Now, b² – 4ac = 49 – 4 . 3 . 2
= 49 – 24 = 25 = 52 > 0
Since, b² – 4ac > 0 then the roots of the given equation are rational and unequal.

Question 10.
If α, β are the roots of ax² + bx + c = 0 then find \(\frac{1}{\alpha^2}+\frac{1}{\beta^2}\).
Solution:
If α, β are the roots of ax² + bx + c = 0 then
α + β = \(\frac{-b}{a}\)
αβ = \(\frac{c}{a}\)
Now, \(\frac{1}{\alpha^2}+\frac{1}{\beta^2}=\frac{\beta^2+\alpha^2}{\alpha^2 \beta^2}=\frac{(\alpha+\beta)^2-2 \alpha \beta}{(\alpha \beta)^2}\)
= \(\frac{\left(\frac{-b}{a}\right)^2-2 \cdot \frac{c}{a}}{\left(\frac{c}{a}\right)^2}=\frac{b^2-2 c a}{c^2}\)

Question 11.
If α, β are the roots of ax² + bx + c = 0 then find \(\frac{1}{\alpha}+\frac{1}{\beta}\). [March ’10]
Solution:
If α, β are the roots of ax² + bx + c = 0
then α + β = \(\frac{-b}{a}\)
αβ = \(\frac{c}{a}\)
Now \(\frac{1}{\alpha}+\frac{1}{\beta}=\frac{\beta+\alpha}{\alpha \beta}=\frac{\frac{-b}{a}}{\frac{c}{a}}=\frac{-b}{c}\)

Question 12.
If α, β are the roots of ax² + bx + c = 0 then find α² + β². [May ’13]
Solution:
If α, β are the roots of ax² + bx + c = 0
then α + β = \(\frac{-b}{a}\), αβ = \(\frac{c}{a}\)
Now α² + β² = (α + β)² – 2αβ
= \(\left(\frac{-b}{a}\right)^2-\frac{2 c}{a}=\frac{b^2}{a^2}-\frac{2 c}{a}=\frac{b^2-2 a c}{a^2}\).

Question 13.
For what values of m, the equation x² – 15 – m (2x – 8) = 0 will have equal roots ?
[March ’13 ’04, May ’08, TS – Mar. 2015; AP – Mar. ’19,’17]
Solution:
Given equation is x² – 15 – m (2x – 8) = 0
x² – 15 – 2mx + 8m = 0
⇒ x² – 2mx + 8m- 15 = 0 ……………(1)
Comparing this equation with
ax² + bx + c = 0, we get
a = 1, b = – 2m, c = 8m – 15
Since (1) have equal roots
⇒ b² – 4ac = 0
⇒ 4m² – 4 (1) (8m – 15) = 0
⇒ 4m² – 32m + 60 = 0
⇒ m² – 8m + 15 = 0
⇒ m² – 5m – 3m+ 15 = 0
⇒ m (m – 5) – 3 (m – 5) = 0
⇒ (m – 3) (m – 5) = 0
⇒ m = 3 (or) m = 5
∴ The values of m are 3, 5.

Question 14.
For what values of in, (m + 1) x² + 2(m + 3) x + (m + 8) = 0 has equal roots? [March ‘03]
Solution:
Given quadratic equation is (m + 1) x² + 2 (m + 3) x +(m + 8) = 0 …………..(1)
Comparing this with ax² + bx + c = 0, we get
a = m + 1, b = 2 (m + 3), c = m + 8
Since (1) have equal roots then b² – 4ac =0
⇒ [2 (m + 3)]² – 4 (m + 1) (m + 8) = 0
⇒ 4 (m + 3)² – 4(m + 1) (m + 8) = 0
⇒ m² + 6m + 9 – m² – 8m – m – 8 = 0
⇒ – 3m – 1 = 0 m = \(\frac{1}{3}\)

Question 15.
For what values of m, (3m + 1) x² + 2(m + 1) x + m = 0 will have equal roots?
Solution:
Given quadratic equation is (3m + 1)x² + 2(m + 1)x +m = 0 ………….(1)
Comparing this with ax² + bx + c = 0, we get
a = 3m + 1, b = 2(m + 1), c = m
since (1) have equal roots then b² – 4ac = 0
4 (m + 1)² – 4m (3m + 1) = 0
m² + 2m + 1 – 3m² – m = 0
– 2m² + m + 1 = 0
2m² – m – 1 = 0
2m² – 2m + m – 1 = 0
2m (m – 1) + 1 (m – 1) = 0
(m – 1) (2m + 1) = 0
m = 1, m = – \(\frac{1}{2}\)

Question 16.
Prove that the roots of (x – a) (x – b) = h² are always real. [AP – May 2013, May ’09]
Solution:
Given quadratic equation is
(x – a) (x – b) = h²
⇒ x² – ax – bx + ab – h² = 0
⇒ x² + (- a – b)x + ab – h² = 0
Comparing this with ax² + bx + c =0, we get
⇒ a = 1, b = – a – b, c = ab – h²
Now,
b² – 4ac = (- a – b)² – 4 (1) (ab – h²)
= a² + b² + 2ab – 4ab + 4h²
=(a² + b² – 2ab) + 4h²
= (a – b)² + (2h)² > 0
Since b² – 4ac > 0 then the roots of the given equation are always real.

Question 17.
Find the quadratic equation, the sum of whose roots is 1 and sum of the sqares of the roots is 13. [May ’07]
Solution:
Let α, β be the roots of required equation.
Given that, the sum of roots, α + β = 1
Sum of squares of the roots, α² + β² = 13
We know that, (α + β)² = α² + β² + 2αβ
⇒ 1 = 13 + 2αβ
⇒ 2αβ = – 12
⇒ αβ = – 6
∴ The quadratic equation whose roots are α, β is x² – (α + β)x + αβ = 0
⇒ x² – x – 6 = 0

Question 18.
Solve the equation 4^{x – 1} – 3 . 2^{x – 1} + 2 = 0. [March ’04]
Solution:
Given equation is 4^{x – 1} – 3 . 2^{x – 1} + 2 = 0
\(\frac{4^x}{4}-3 \cdot \frac{2^x}{2}+2\) = 0
\(\frac{\left(2^2\right)^x}{4}-3 \cdot \frac{2^x}{2}+2\) = 0
\(\frac{\left(2^x\right)^2}{4}-3 \cdot \frac{2^x}{2}+2\) = 0
Let 2^x = a
⇒ \(\frac{\mathrm{a}^2}{4}-\frac{3 \mathrm{a}}{2}\) + 2 = 0
⇒ a² – 6a + 8 = 0
⇒ a² – 4a – 2a + 8 = 0
⇒ (a – 4) (a – 2) = 0
⇒ a = 4 (or) 2.

Case – I:
lf a = 4
⇒ 2^x = 4
⇒ 2^x = 2²
⇒ x = 2.

Case – 2:
If a = 2
⇒ 2^x = 2
⇒ x = 1
∴ The solution set of the given equation is {2, 1}.

Question 19.
Find the maximum or minimum of the expression 12x – x² – 32 x varies over R.
Solution:
Given quadratic expression is – x + 12x – 32
Comparing this expression with ax + bx + c, we have a = – 1, b = 12, c = – 32
Here a = – 1 < 0.
Then – x² + 12x – 32 has absolute maximum at x = \(\frac{-b}{2 a}=\frac{-12}{2(-1)}\) = 6
∴ The maximum value = \(\frac{4 a c-b^2}{4 a}\)
= \(\frac{4(-1)(-32)-144}{4(-1)}\)
= \(\frac{128-144}{-4}=\frac{-16}{-4}\) = 4.

Question 20.
If the quadratic equations ax² + 2bx + c = 0 and ax² + 2cx + b = 0, (b ≠ c) have a common root then show that a + 4b + 4c = 0.
Solution:
Given quadratic equations are ax² + 2bx + c = 0
Comparing this equation with a₁x² + b₁x + c₁ = 0, we get
a₁ = a, b₁ = 2b, c₁ = c
Now, a₂x + 2cx + b = 0
Comparing this with a₂x² + b₂x + c₂ = 0
we get a₂ = a, b₂ = 2c, c₂ = b
The condition for two quadratic equations
a₁x² + b₁x + c₁ = 0 and a₂x² + b₂x + c₂ = 0 to have a common root is
(c₁a₂ – c₂a₁)² = (a₁b₂ – a₂b₁) (b₁c₂ – b₂c₁)
⇒ (ca – ba)² = (a₂c – a₂b) (2bb – 2cc)
⇒ a² (c – b)² = 2a (c – b) 2 (b² – c²)
⇒ a² (c – b)² = 4a (c – b) (b – c) (c – b)
⇒ a = – 4 (c + b) a = – 4c – 4b
a + 4b + 4c = 0.

Question 21.
If x² – 6x + 5 = 0 and x² – 12x + p = 0 have a common root, then find p. [TS – May 2015, Mar. 2017]
Solution:
Given quadratic equations are x² – 6x + 5 = 0
Comparing this equation with
a₁x² + b₁x + c₁ = 0, we get
a₁ = 1, b₁ = – 6, c₁ = 5
Now, x² – 12x + p = 0,
Corn paring with a₂x² + b₂x + c₂ = 0
we get a₂ = 1, b₂ = – 12, c₂ = p
The condition for two quadratic equations
a₁x² + b₁x + c₁ = 0 and a₂x2 + b₂x + c₂ = 0 to have a common root is
(c₁a₂ – c₂a₁)² = (a₁b₂ – a₂b₁) (b₁c₂ – b₂c₁)
(5 (1) – p(1))² = (1 (- 12) – 1 (- 6)) ((- 6)p – (- 12) 5)
⇒ (5 – p)² = – 6 (- 6p + 60)
⇒ p² + 25 – 10p = 36p – 360
⇒ p² – 16p + 385 = 0
⇒ p² – 35p – 11p + 385 = 0
⇒ p (p – 35) – 11 (p – 35) = 0
⇒ (p – 11) (p – 35) = 0
⇒ p = 11 (or) p = 35
∴ p = 11 (or) 35.

Question 22.
For what values of x, the expression x² – 5x + 6 is positive?
Solution:
The given quadratic expression is x² – 5x + 6
⇒ x² – 3x – 2x + 6 = x (x – 3) – 2 (x – 3) = (x – 2)(x – 3)
i) If 2 < x < 3 then the expression x² – 5x + 6 is ‘- ve’.
ii) If x < 2 (or) x> 3 then the expression x² – 5x + 6 is ‘+ ve’.
iii) If x = 2 (or) x = 3 then the expression x² – 5x + 6 = 0

Question 23.
For what values of x, the expression x² – 5x – 6 is negative? [March ‘95]
Solution:
Given quadratic expression is x² – 5x – 6
= x² – 6x + x – 6
= x (x – 6) + 1 (x – 6)
= (x + 1)(x – 6)
i) If – 1 < x < 6 then the expression x² – 5x – 6 is ‘-ve’.
ii) If x < – 1 or x > 6 then the expression x² – 5x – 6 is ‘+ ve’.
iii) If x = – 1 (or) x = 6 then the expression x² – 5x – 6 = 0.

Question 24.
Discuss the signs of the expression x² – 5x + 4 for x ∈ R. [May ’95].
Solution:
Given quadratic expression is x² – 5x + 4
= x² – 4x – x + 4
= x (x – 4) + 1 (x – 4)
= (x – 1) (x – 4)
i) If 1 < x < 4 then the expression x² – 5x + 4 is ’-ve’.
ii) If x < 1 (or) x > 4 then the expression x² – 5x + 4 is ’+ve’.
iii) If x = 1 (or) x = 4 then the expression x² – 5x + 4 = 0.

Question 25.
Find the maximum or minimum value of the expression 12x – x² – 32. [May ’06]
Solution:
Given quadratic expression is – x² + 12x – 32 = 0
Comparing this expression with ax² + bx + c, we have a = – 1, b = 12, c = – 32
Here, a = – 1 < 0.
Then – x² + 12x – 32 has absolute maximum at x = \(\frac{-b}{2 a}=\frac{-12}{2(-1)}\) = 6
The maximum value = \(\frac{4 a c-b^2}{4 a}\)
= \(\frac{4(-1)(-32)-144}{4(-1)}=\frac{128-144}{-4}\)
= \(\frac{-16}{-4}\) = 4.

Question 26.
Find the maximum or minimum value of the expression 2x – 7 – 5x². [May ’10. March ’14, ’12].
Solution:
Given quadratic expression is – 5x² + 2x – 7
Comparing this expression with ax² + bx + c,
we have a = – 5, b = 2, c = – 7
Here, a = – 5 < 0, – 5x² + 2x – 7 has absolute maximum at
x = \(\frac{-b}{2 a}=\frac{-2}{2(-5)}=\frac{1}{5}\)
∴ Maximum value is \(\frac{4 a c-b^2}{4 a}=\frac{4(-5)(-7)-4}{4(-5)}\)
= \(\frac{140-4}{-20}=\frac{136}{-20}=\frac{-34}{5}\)

Question 27.
Find the maximum or minimum value of the expression 3x² + 2x + 11. [May ’92]
Solution:
Given quadratic expression is 3x² + 2x + 11
Comparing this expression with ax² + bx + c, we have a = 3, b = 2, c = 11
Here, a = 3 >0, 3x² + 2x + 11 has absolute minimum at
x = \(\frac{-b}{2 a}=\frac{-2}{2(3)}=\frac{-1}{3}\)
∴ The minimum value = \(\frac{4 a c-b^2}{4 a}=\frac{4(3)(11)-4}{4(3)}\)
= \(\frac{132-4}{12}=\frac{128}{12}=\frac{32}{3}\).

Question 28.
Find the maximum or minimum value of the expression x² – x + 7 as x varies over R. [May ’14]
Solution:
Given quadratic expression is x² – x + 7
Comparing this expression with ax² + bx + c, we have a = 1, b = – 1, c = 7
Here, a = 1 > 0, x² – x + 7 has absolute minimum at x = \(\frac{-b}{2 a}=\frac{-(-1)}{2 \cdot 1}=\frac{1}{2}\)
∴ The minimum value = \(\frac{4 a c-b^2}{4 a}=\frac{4(1)(7)-(-1)^2}{4(1)}\)
= \(\frac{28-1}{4}=\frac{27}{4}\)

Question 29.
Find the roots of the equation 6√5 x² – 9x – 3√5 = 0.
Solution:
\(\frac{\sqrt{5}}{2}, \frac{-1}{\sqrt{5}}\)

Question 30.
Form a quadratic equation whose roots are 2√3 – 5 and – 2√3 – 5.
Solution:
x² + 10x + 13 = 0.

Question 31.
Form a quadratic equation whose roots are \(\frac{\mathbf{m}}{\mathbf{n}}, \frac{-\mathbf{n}}{\mathbf{m}}\), (m ≠ 0, n ≠ 0)
Solution:
mnx² – (m² – n²)x – mn = 0

Question 32.
If α, β are the roots of ax² + bx + c = 0 then find α³ + β³.
Solution:
\(\frac{3 a b c-b^3}{a^3}\)

Question 33.
For what values of m, x² + (m + 3) x + (m + 6) = 0 will have equal roots?
Solution:
m = 3, – 5.

Question 34.
For what values of m, z² – 2 (1 + 3m) x + 7 (3 + 2m) = 0 will have equal roots?
Solution:
m = 2, \(\frac{-10}{9}\).

Question 35.
For what vaIueof m, (2m+ 1)x² + 2(m + 3)x + (m + 5) = 0 will have equal rooti?
Solution:
m = \(\frac{-5 \pm \sqrt{41}}{2}\)

Question 36.
Find the quadratic equation, the sum of whose roots is 7 and sum of the squares of the roots is 25.
Solution:
x² – 7x + 12 = 0

Question 37.
So1ve the equation 3^{1 + x} + 3^{1 – x} = 10.
Solution:
{- 1, 1}

Question 38.
Solve 7^{1 + x} + 7^{1 – x} = 50 for real x.
Solution:
{- 1, 1}

Question 39.
If x² – 6x + 5 = 0 and x² – 3ax + 35 = 0 have a common root, then find a.
Solution:
4 (or) 2.

Question 40.
For what values of x, the expression x² – 5x + 14 is positive?
Solution:
∀ x ∈ R

Question 41.
For what values of x, the expression 3x² + 4x + 4 is positive?
Solution:
∀ x ∈ R

Question 42.
For what values of x, the expression x² – 7x + 10 is negative?
Solution:
2 < x < 5

Question 43.
For what values of x, the expression 15 + 4x – 3x² is negative? [AP – Mar. 2015]
Solution:
x < – \(\frac{5}{3}\) (or) x > 3.

Question 44.
Find the changes in the sign of 4x – 5x² + 2 for x ∈ R.
Solution:
Positive for \(\frac{2-\sqrt{14}}{5}<x<\frac{2+\sqrt{14}}{5}\)
negative for x < \(\frac{2-\sqrt{14}}{5}\) (or) x > \(\frac{2+\sqrt{14}}{5}\)