TS Inter 2nd Year Physics Study Material Chapter 2 Ray Optics and Optical Instruments

Telangana TSBIE TS Inter 2nd Year Physics Study Material 2nd Lesson Ray Optics and Optical Instruments Textbook Questions and Answers.

TS Inter 2nd Year Physics Study Material 2nd Lesson Ray Optics and Optical Instruments

Very Short Answer Type Questions

Question 1.
Define focal length and radius of curvature of a concave lens.
Answer:
Focal length (f) :
The distance between centre of lens and principle focus is called “focal length (f)”

Radius of curvature :
The distance between centre of lens to centre of curvature is called “Radius of curvature” (R).

Question 2.
What do you understand by the terms ‘focus’ and ‘principal focus’ in the context of lenses?
Answer:
Principal focus :
When a paraxial beam of light making small angle with principal axis falls on a lens, after refraction the refracted rays will converge at a point or they appear to diverge from a point on principal axis. This point is called “principal focus”.

Focus :
When parallel rays making some angle with principal axis passes through a lens then after refraction they will converge at a point in a plane passing through F and perpendicular to principal axis called focal plane.

This point of convergence or divergence in a focal plane is called focus. If the focus is on principal axis it is called principal focus.

Question 3.
What is optical density and how is it different from mass density?
Answer:
An optically denser medium is one in which velocity of light is less.

An optically rarer medium may have less mass density.
Ex: Mass density of turpentine is less than water but optical density of turpentine is more than water.

Question 4.
What are the laws of reflection through curved mirrors?
Answer:
Laws of reflection :

1. Angle of reflection is equais to angle of incidence (∠r = ∠i).
2. The incident ray, the reflected ray and normal to the reflecting surface lie in the same plane.

Question 5.
Define ‘power’ of a convex lens. What is [TS Mar., May 16; AP Mar. ’17, May 16]
Answer:
Power of a lens (P) :
Power of a lens is defined as the tangent of the angle by which it converges or diverges a beam of light falling at unit distance from the optical centre, i.e.
tan δ = \(\frac{h}{f}\), h = 1, δ = \(\frac{h}{f}\)
Power P = \(\frac{1}{f}\) unit: dioptre. Here f is in metre.

Question 6.
A concave mirror of focal length 10 cm is placed at a distance 35 cm from a wall. How far from the wall should an object be placed so that its real image is formed on the wall? [TS May ’19]
Answer:
Focal length (f) = 10 cm.
Image distance (v) = 35 cm
Position of object (u) = ?

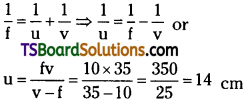
Object distance is from pole of mirror.
From wall the distance of the object
d = 35 – 14 = 21 cm.

Question 7.
A concave mirror produces an image of a long vertical pin, placed 40 cm from the mirror at the position of the object. Find the focal length of the mirror.
Answer:
Given the object and image are at a distance of 40 cm i.e., v = u = 40 cm
In concave mirror when object is at 2f then image is also at 2f ⇒ 2f = 40 cm
∴ Focal length of concave mirror = 20 cm.

Question 8.
A small angled prism of 4° deviates a ray through 2.48°. Find the refractive index of the prism. [AP Mar. 19, 18, June 15]
Answer:
Angle of prism A = 4°.
Angle of deviation, d = 2.48°
Refractive index, n = 1 for small angled prism d = (n – 1) A
∴ 2.48 = (n – 1) 4
⇒ n – 1 = \(\frac{2.48}{4}\) n = \(\frac{2.48}{4}\) + 1 = 0.62 + 1 = 1.62
∴ n = 1.62.

Question 9.
What is ‘dispersion’? Which colour gets relatively more dispersed? [Mar., May ’14]
Answer:
Dispersion :
The phenomenon of splitting of light into its component colours is known as dispersion.

Dispersion takes place due to change in refractive index of medium for different wave lengths.

Bending of violet component of white light is maximum due to its short wavelength.

Question 10.
The focal length of a concave lens is 30 cm. Where should an object be placed so that its image is 1/10 of its size?
Answer:
Here f = – 30 cm, u = ? (For a concave lens)
m = \(\frac{v}{u}=\frac{1}{10}\)
v is – ve because virtual image is formed

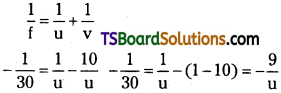
∴ u = 9 × 30 = 270 cm

Question 11.
What is myopia? How can it be corrected? [TS May 17, Mar. 17, 15, June 15; AP Mar., June ’15]
Answer:
Hypermyopia :
It is an eye defect. Where light rays coming from distant object are converged at a point infront of retina. This defect is called “short sightendness or Hyper myopia”, This defect can be compensated by using a concave lens.

Question 12.
What is hypermetropia? How can it be corrected? [AP May 17, Mar.’ 16; TS Mar. 18]
Answer:
Hypermetropia :
It is an eye defect. In this defect, eye lens focuses the incoming light at a point behind the retina. This is called “far sightedness or Hypermetropia”. This defect can be compensated by using a convex lens.

Short Answer Questions

Question 1.
A light ray passes through a prism of angle A in a position of minimum deviation. Obtain an expression for (a) the angle of incidence in terms of the angle of the prism and the angle of minimum devia¬tion (b) the angle of refraction in terms of the refractive index of the prism.
Answer:
a) Let a light ray PQ enters the prism with angle of incidence i and emerges out with an angle ‘e’. When it is at minimum deviation position.
from quadrilateral AQNR
∠A + ∠QNR = 180° …………… (1)
In triangle QNR,
r₁ + r₂ + ∠QNR = 180° …………… (2)
From equation (1) and (2);
r₁ + r₂ = A ……………. (3)

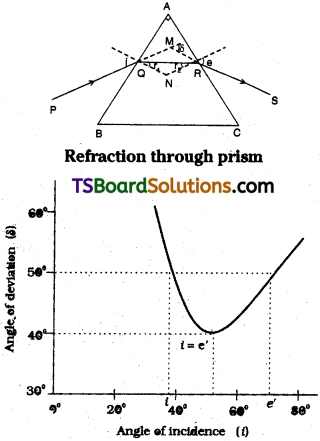
Deviation δ = (i₁ – r₁) + (i₂ – r₂)
= (i₁ + i₂) – r₁ + r₂
At minimum deviation position
i₁ = i₂ = p and r₁ = r₂ = r
∴ δ = i₁ + i₂ – A ⇒ 2i = A + δ
∴ i = \(\frac{A+\delta}{2}\) ……….. (4)

b) From equation (3) r₁ + r₂ = A.
∴ r₁ + r₂ = 2r = A ⇒ r = \(\frac{A}{2}\)

Question 2.
Define focal length of a concave mirror. Prove that the radius of curvature of a concave mirror is double its focal length. [AP Mar. ’19, ’17. May ’18, ’16]
Answer:
Focal length (f) :
The distance between principal focus and pole of mirror is called “focal length” (f).

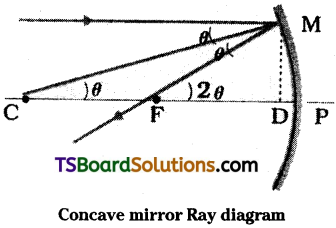
R = 2f proof:
Let ‘C’ be the centre of curvature of a concave mirror, ‘P’ be its pole and F be the principal focus. Line passing through P and F is called principal axis. Consider a ray parallel to principal axis falls on the mirror at ‘M’. After reflection, it passes through principal focus ‘F’. Join CM. It is perpendicular to mirror at M. Let angle of incidence is 0 and MD is the perpendicular at M. From principles of geometry

∠MCP = θ and ∠MFP = 2θ
Now tan θ = \(\frac{MD}{CD}\) = θ ………….. (1)
(∵ when θ is small tan θ = θ)
and tan 2θ = \(\frac{MD}{FD}\) = 2θ ………… (2)
From equation (1) and (2); FD = CD/2 ………….. (3)
But when D is very close to ‘P’
FD = FP & and CD = CP
∴ f = \(\frac{R}{2}\) ⇒ R = 2f
⇒ Radius of curvature R = 2 × focal length.

Question 3.
A mobile phone lies along the principal axis of a concave mirror longitudinally. Explain why the magnification is not uniform.
Answer:
Let a mobile phone is placed along the principal axis of a concave mirror longitudinally then magnification is not uniform.
Explanation :
Let the mobile phone is placed on principal axis as shown.

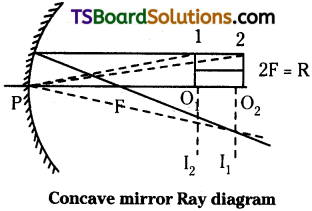
Let the points 1 and 2 will represent the two ends of mobile phone. For the end 1 object distance is PO₁ = u₁. For the end ‘2’ object distance is PO₂ = u₂. Light rays coming from nearer end will form more magnified image I₁ and light rays coming from remote point will form less magnified image I₂.

Because in mirrors \(\frac{1}{f}=\frac{1}{u}+\frac{1}{v}\)
Since f is same. When u increases v will decrease. So v₁ > v₂.

But linear magnification m = \(\frac{v}{u}\)
In first case m₁ = \(\frac{v_1}{u_1}\) and
in second casern m<₂ = \(\frac{v_2}{u_2}\)
Here v₁ > v₂ and u₁ < u₂ as a result m₁ > m₂.
So magnification of mobile phone placed longitudinally is not uniform.

Question 4.
Explain the Cartesian sign convention for mirrors.
Answer:
Cartesian sign convention :

1. All distances must be measured from the pole of the mirror (centre of Jens).
2. The distances measured along the direction of the incident ray are taken as positive and against the direction of incident ray are taken as negative.
3. Distances (heights) above the principal axis of mirror or lens are taken as positive and below are taken as ve’.

Question 5.
Define critical angle. Explain total internal reflection using a neat diagram. [AP Mar. 15. May 14; TS Mar. ’18. ’15, May 17]
Answer:
Critical Angle :
When the light travels from denser to rarer medium, for which angle of incidence, the angle of refraction is 90° is called critical angle of denser medium.

Explanation :
Consider two transparent media say 1 and 2. Where medium 1 is denser medium and medium 2 is a rarer medium.

Let light rays are starting from point A in denser medium. For angle of incidence i₁ they will strike the boundary layer say at ‘O₁‘. Draw normal at ‘O₁‘ when light rays are travelling from denser medium to they will bend away from normal so angle of refraction (r₁) greater than i₁.

When angle of incidence is gradually increased then angle of refraction r₂ will also increase. For a particular angle of incidence ic angle of refraction r = 90° it is called critical angle.

For angle of incidence i > i_c there is no refracted ray in medium two. At this stage light rays are not able to penetrate the boundary layer so they will come back into 1st medium. This is called total internal reflection.

Question 6.
Explain the formation of a mirage. [TS Mar. 19, May 18; AP Mar. 16]
Answer:
Mirage are formed on hot summer days. On a hot summer day earth is heated due to heat radiation from sun. So air near the earth is heated its density will decrease and hot air goes up density. In this process, we will get a special atmosphere where low density air is near earth and its density gradually increases while going up.

Now light rays coming from sun will travel from denser air layers to less dense region (rarer medium). So they will continuously bend away from normal and undergoes total internal reflection.

As a result we will get inverted images of tall objects and an illusion as if there are ponds on high ways even though there is no wet surface. This type of illusion is called mirages.

Question 7.
Explain the formation of a rainbow.
Answer:
Rainbow :
Rainbow is due to dispersion of white light through water drops.

Condition to set Rainbow are 1) Sun should be shining at one side and rain should be at the other side of sky. Formation of rainbow is at least a three step process i.e., refraction – reflection and refraction i.e., light rays entering the water drop should suffer total internal reflection and comes out of the drop through refraction.

Explanation :
Sun lightis first refracted as it enters a rain drop. This causes the different wave lengths of white light to separate. Longer wavelengths (red colour) will bend less and shorter wavelengths (blue and violet colours) will bend more. These component rays will suffer total internal reflection inside the water drop at water – air boundary and again they will travel in water drop and finally they will come out of water drop after refraction.

In this way we will get rainbow on a rainy day when sun is shining at other end.

Question 8.
Why does the setting sun appear red? [AP Mar.’ 14, June ‘ 15; TS Mar.’ 17; May 15]
Answer:
Setting sun appears red due to scattering.

The amount of scattering is inversely proportional to fourth power of the wavelength. i.e, Amount of scattering αλ⁴. This is called Rayleigh’s formula.

Light of shorter wavelengths will scatter much more than light of longer wavelength. Due to this reason, blue light will scatter more than red light.

Rayleigh formula will hold good when size of the particles << λ. For particles of large size i.e., at water droplets, large dust particles etc., visible light of all wavelengths will scatter equally.

At sun set or at sun rise light rays will pass through a longer distances through atmosphere. In this process light of shorter wavelength is removed and light of longer wave length (Red) will suffer less scattering reaches our eye. As a result at sunrise and of sunset sun will appear red.

Question 9.
With a neat labelled diagram explain the formation of image in a simple microscope. [AP Mar. 18, 16, 15. May ’16. June 15; TS Mar., May 16]
Answer:
A single convex lens is used in a simple microscope. Here object distance is adjusted to be less than focal length of the convex lens used i.e., u < f. By proper adjustment of object distance final virtual image is made to form either at near point or at infinity.

Angular magnification (m) = tan θ₀ = \(\frac{h}{d}\)
Where h’ is say height of image and ‘d’ is ‘d’ near point distance.
For maximum clarity eye will form image of parallel rays at near point.

When image is at near point:
Linear magnification m = \(\frac{v}{u}\) = 1 + \(\frac{D}{f}\) because final image is at near point D.

Question 10.
What is the position of the object for a simple microscope? What is the maximum magnification of a simple micro-scope for a realistic focal length?
Answer:
In simple microscope, object is placed in between centre of lens (C) and its principal focus (f). As a result a magnified virtual image is made to form either at near point or at infinity with suitable object distance ‘u’.

In simple microscope near point magnification m = (1 + D/f) when image is at infinity magnification m = D/f.

Magnification – Limits :
Theoretically, we can achieve very high magnification m’ for simple microscope. But due to practical consideration, we can not increase magnification beyond a limit.
Ex : For a magnification of 5 at for point focal length of convex lens
f = \(\frac{D}{m}=\frac{25}{5}\) = 5 cm
with this focal length thickness of lens is high, so dispersion takes place and chromatic aberration will come into account.

Even with a very careful design of convex lens we can not get a magnification of more than ten with simple microscope. Magnification of m = 5 to 10 is the possible limit.

Long Answer Questions

Question 1.
a) What is the cartesian sign convention? Applying this convention and using a neat diagram, derive an expression for finding the image distance using the mirror equation.
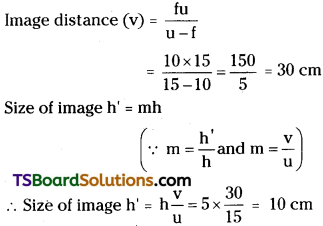
b) An object of 5 cm height is placed at a distance of 15 cm from a concave mirror of radius of curvature 20 cm. Find the size of the image.
Answer:
a) Cartesian sign convention :

1. All distances must be measured from the pole of the mirror (or) the optical centre of lens.
2. The distances measured along the direction of the incident ray are taken as positive and against the direction of incident ray are taken as negative.
3. The distances (heights) measured above the principal axis of mirror or lens are taken as positive and below are taken as ve’.

Mirror equation :
The relation \(\frac{1}{f}=\frac{1}{u}+\frac{1}{v}\) is known as “mirror equation”.

Derivation of equation for image distance :

Let an object is placed infront of a con-cave mirror beyond centre of curvature then a real image is formed between f and c as shown in figure.

As per sign convention all distances must be measured from pole of mirror P.

∴ Focal length f = PF it is against to direction of incident ray so f is – ve.

Image distance from pole of mirror is v = PB’. It is measured against direction of incident ray so image distance v is – ve.

By applying sign convention to mirror equation \(\frac{1}{f}=\frac{1}{u}+\frac{1}{v}\) becomes

b) Height of object (h) = 5 cm;
Object distance (u) = 15 cm
Radius of curvature (R) = 2f = 20 cm ⇒ f = 10 cm

Question 2.
a) Using a neat labelled diagram derive the mirror equation Define linear magnification.
b) An object is placed at 5 cm from a con¬vex is the position and nature of the image?
Answer:
a) Let an object AB is placed in front of a concave mirror beyond radius of curvature C’, The parallel ray comming from object after reflection at mirror (M) will pass through principal focus (F). The ray passing through centre of curvature falls normally on mirror at N. Intersection of these two rays will give position of image A’B’. This is as shown in figure.

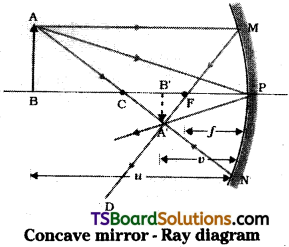
In figure Triangle A’B’ P and MPF are similar triangles by comparing ratio of sides

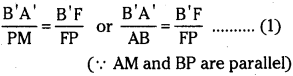
But triangles A ‘ B’ P and BAP are also similar.

In this equation
B’P Image distance B’P = v, FP = Focal length and BP = object distance = u.
∵ These distances measured from pole ‘P’ are against to direction of incident ray v is – ve, f is – ve and u is – ve.
By using these values in equation (3),

This is known as “mirror equation”.

Linear magnification :
The ratio of height of image (h’) to height of object (h) is called “linear magnification”.

Linear magnification, m = \(\frac{h’}{h}=\frac{-v}{u}\).

b) Object distance u = 5 cm; Focal length of convex lens f = 15 cm
Image distance v = ?

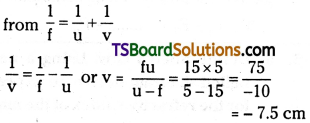
– ve sign indicates image is virtual image.
∴ Position of image is 7.5 cm infront of the lens.

3. a) Derive an expression for a thin double convex lens. Can you apply the same to a double concave lens too?
b) An object is placed at a distance of 20 cm from a thin double convex iens of focal length 15 cm, Find the position and magnification of the image.
Answer:
a) Refraction through double convex lens :
Refraction through double convex lens is studied in two steps.

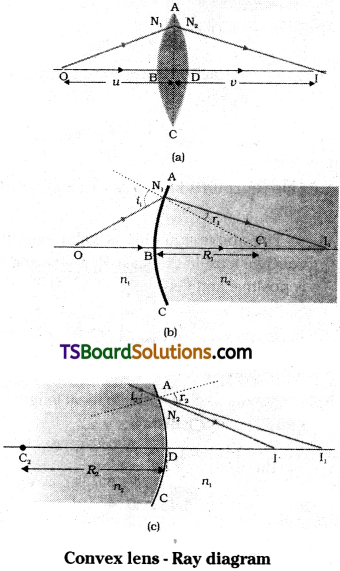
1st step :
Let the first surface will form the image of object ‘O’ at I₁ for this case
\(\frac{\mathrm{n}_1}{\mathrm{OB}}+\frac{\mathrm{n}_2}{\mathrm{BI}_1}=\frac{\mathrm{n}_2-\mathrm{n}_1}{\mathrm{BC}_1}\) ………. (1)
In 2nd step the second surface will form image at I where 1st image Ij will act as virtual object for 2nd surface,
∴ \(-\frac{\mathrm{n}_2}{\mathrm{DI_1}}+\frac{\mathrm{n}_1}{\mathrm{DI}}=\frac{\mathrm{n}_2-\mathrm{n}_1}{\mathrm{DC_2}}\) ………. (2)
For thin lens thickness, BD is small
so BI = D₁
By adding equaion (1) and (2)
\(\frac{n_1}{OB}+\frac{n_1}{DI}\) = (n₂ – n₁ [latex]\frac{1}{BC_1}+\frac{1}{DC_2}[/latex] …………… (3)
If point object is at infinity then OB = ∞ and DI = focal length f.

The distance BC₁ = + R₁ and DC₂ = – R₂ by using these values in equation (3)
\(\frac{n_1}{f}\) = (n₂ – n₁) (\(\frac{1}{R_1}-\frac{1}{R_2}\))

Here refraction is from air to glass so n₁ = 1 and n₂ = n₂₁
∴ \(\frac{1}{f}\) = (n₂₁ – 1) (\(\frac{1}{R_1}-\frac{1}{R_2}\))

This equation is known as lens makers formula.
The above equation is valid even for concave lens. In that case, R₁ is – ve and R₂ is positive and f is – ve.
So \(\frac{1}{f}\) = (n₂₁ – 1) (\(\frac{1}{R_1}-\frac{1}{R_2}\)) is vaild even for concave lens.

b) Object distance u = 20 cm; focal length f = 15 cm. Image distance v = ?

∴ Image distance v = 60 cm.
Magnification m = \(\frac{v}{u}=-\frac{60}{20}\) = 3

Question 4.
Obtain an expression for the combined focal length for two thin convex lenses kept in contact and hence obtain an expression for the combined power of the combination of the lenses.
Answer:
Let two thin lenses A, B with focal lengths f₁ and f₂ are in contact. Let ‘O’ is a point object on the principal axis. The first lens will form the image I₁ of the object

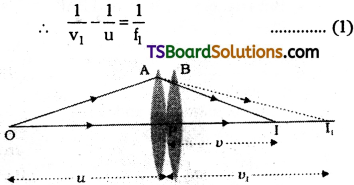
This first image I₁ will act as an object to second lens and final image I is produced.
∴ \(\frac{1}{v}-\frac{1}{v_1}=\frac{1}{f_2}\) ………….. (2)
by adding equation (1) and (2)
\(\frac{1}{v}-\frac{1}{u}=\frac{1}{f_1}+\frac{1}{f_2}\).
But \(\frac{1}{v}-\frac{1}{u}=\frac{1}{f}\)
Here the lens system is regarded as equivalent to a single lens of focal length ‘f’.
∴ \(\frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}\)
If there are n lenses in contact then equivalent focal length is given by

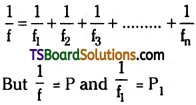
So interms of power,
P = P₁ + P₂ + P₃ + …………. + P_n
Equivalent focal length of lenses in contact is \(\frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}+\frac{1}{f_3}+………….+\frac{1}{f_n}\)

Equivalent power of lens combination is
P = P₁ + P₂ + …………. + P_n.

Question 5.
a) Define Snell’s Law. Using a neat la¬belled diagram derive an expression for the refractive index of the material of an equilateral prism.
b) A ray of light, after passing through a medium, meets the surface separating the medium from air to an angle of 45° and is just not refracted. What is the refractive index of the medium?
Answer:
a) Snell’s Law :
The ratio of sine of angle of incidence to sine of angle of refraction is constant for a given pair of media. i.e., sin i / sin r = n₂₁.
where n₂₁ is refractive index of 2nd medium w.r.to 1st medium.

Derivation of refractive index of prism :
Let ABC is the cross-section of a prism. AB and AC are refracting surfaces. ∠A is angle of prism. Let a light ray PQ falls on AB with angle of incidence i₁. After refraction path of light ray QR is parallel to prism base. RS is emergent ray with angle of emergence ‘e’. When PQ and RS are extended they will meet at M. Angle of deviation is δ. They are as show in figure.

In figure, ∠A + ∠QNR = 180° …………. (1)
In triangle QNR,
r₁ + r₂ + ∠QNR = 180° ………….. (2)
From equation (1) and (2),
r₁ + r₂ = A ……………. I
Angle of deviation
δ = (i₁ – r₁) + (e – r₂) …………. (3)
Or δ = i₁ + e – A …………… (4)
At minimum deviation position i₁ = e and r₁ = r₂
and δ = δ_m by using these values
r₁ + r₂ = A
⇒ 2r = A
⇒ r = A/2 ………….. (5)
δ = i₁ + e – A = 2i₁ – A

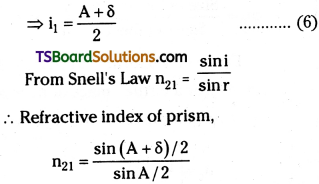
b) Angle of incidence, I = 45°
Angle of refraction, r = 90° ⇒ i = i_c
Refractive index n₂₁ = \(\frac{1}{\sin C}=\frac{1}{\frac{1}{\sqrt{2}}}=\sqrt{2}\) = 1.414

Question 6.
Draw a neat labelled diagram of a compound microscope and explain its working. Derive an expression for its magnification.
Answer:
A compound microscope consists of two convex lenses mounted coaxially. Lens near the object is called objective. Focal length of objective is less.

Object lens forms a real, magnified image of the object. It will work as an object to eyelens.

Lens near the eye is called eye lens its focal length is high. It will form a magnified virtual final image at near point or at infinity. Final image is inverted w.r.t the original.

Ray diagram is as shown in figure. Dis-tance between objective and eye lens is called tube length ‘L’.

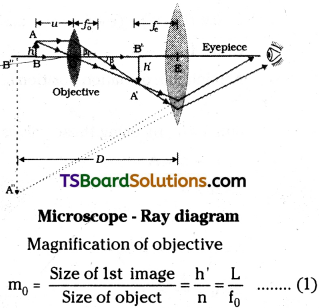
Eye lens will form the final image at infinity or a virtual image at near point.
Near point magnification of eye lens
m_e = (1 + \(\frac{D}{f_e}\)) …………….. (2)
When final image is at infinity Magnifcation of eye lens m_e = \(\frac{D}{f_e}\) …………… (3)
Total magnification of compound microscope m = m₀ . m_e.
∴ Total magnification
i) For final image at near point m = \(\frac{L}{f_0}\)(1+\(\frac{D}{f_e}\))
ii) For final image at infinity m = \(\frac{L}{f_0}.\frac{D}{f_e}\)
Magnification of compound microscope is very high.

Solved Problems

Question 1.
A light wave of frequency 4 × 10¹⁴ Hz and a wavelength of 5 × 10^-7m passes through a medium. Estimate the refractive index of the medium.
Answer:
Frequency (u) = 4 × 10⁴ C/S,
Wavelength (λ) = 5 × 10^-7 m, µ = ?

Question 2.
A ray of light is incident at an angle of 60 on the face of a prism of angle 30°. The emergent ray makes an angle of 30° with the incident ray. Calculate the refractive index of the material of the prism.
Answer:
Angle of incidence (i) = 60°;
Angle of prism (A) = 30°
Angle of deviation (d) = 30°
In prism i₁ + i₂ = A + d
⇒ 60 + i₂ = 30 + 30
⇒ i₂ = 0 ⇒ r₂ = 0
But r₁ + r₂ = A ⇒ r₁ + 0 = 30° or r₁ = 30°

Question 3.
Two lenses of power – 1.75D and + 2.25D respectively are placed in contact. Calculate the focal length of the combination.
Answer:
Power of 1st lens (P₁) = – 1.75 D;
Power of 2nd lens (P₂) = + 2.25 D
When in contact total power
P = P₁ + P₂ = – 1.75 + 2.25 = 0.5
Focal length of combination
f = \(\frac{100}{P}=\frac{100}{0.5}\) = 200 cm

Question 4.
Some rays falling on a converging lens are focussed 20 cm from the lens. When a diverging lens is placed in contact with the converging lens, the rays are focussed 30 cm from the combination. What is the focal length of the diverging lens?
Answer:
f_c = 20 cm
When combined with diverging lens light rays are focused at 30 cm
⇒ f_comb = 30 cm = F (say)

– ve sign indicates that it is a diverging lens.

Question 5.
A small angled prism of 4° deviates a ray through 2.48°. Find the refractive index of the prism. [June ’15]
Answer:
Angle of prism (A) = 4°.
Angle of deviation (d) = 2.48°
Refractive index, n = 1 for small angled prism
d = (n₂₁ – 1) A
∴ 2.48 = (n₂₁ – 1) 4 ⇒ n₂₁ – 1 = \(\frac{2.48}{4}\)
n₂₁ = \(\frac{2.48}{4}\) + 1 = 0.62 + 1 = 1.62

Question 6.
A double convex lens of focal length 15 cm is used as a magnifying glass in order to produce an erect image which is 3 times magnified. What is the distance between the object and the lens?
Answer:
Focal length (f) = 15 cm;
Magnification (m) = 3 = v/u ⇒ v = 34

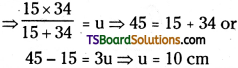
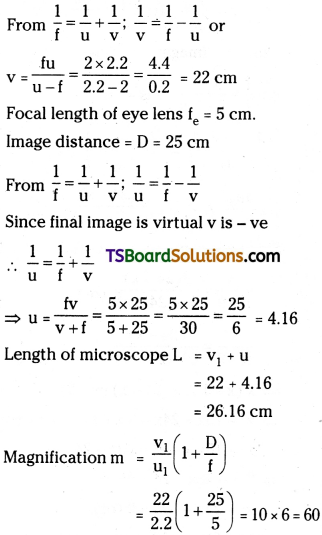
Question 7.
A compound microscope consists of an object lens of focal length 2 cm and an eyepiece of focal length 5 cm. When an object is placed at 2.2 cm from the object lens, the final image is at 25 cm from the eye lens. What is the distance between the lenses? What is the total linear magnification?
Answer:
Focal length of object lens (f₀) = 2 cm;
Object distance (u) = 2.2 cm

Question 8.
The distance between two point sources of light is 24 cm. Where should you place a converging lens, of focal length 9 cm, so that the images of both sources are formed at the same point?
Answer:
Given distance between sources = 24 cm;
Focal length of convex lens (f) = 9 cm
Both images are formed at same point i.e., image distance V is same. This will happen when one image is real and another is virtual.

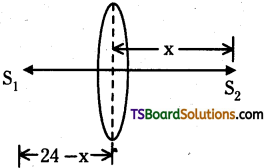
Case – I: Real image let object distance

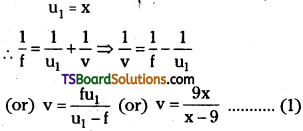
Case – II: Image is virtual then u₂ = 24 – x

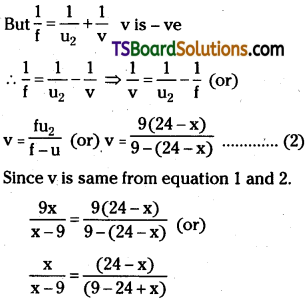
⇒ x (x – 15) = (24 – x) (x – 9)
⇒ x² – 15x = 24x – 9 × 24 – x² + 9x (or) x² – 15x = – x² + 33x – 24 × 9;
⇒ 2x² – 48x + 24 × 9 = 0
∴ x² – 24x – 108 = 0 (or)
(x – 6) (x – 18) = 0
∴ x = 6 cm or x = 18 cm.

Question 9.
Find two positions of an object, placed in front of a concave mirror of focal length 15 cm, so that the image formed is 3 times the size of the object.
Answer:
Focal length of lens (f) = 15 cm;
Magnification (m) = 3 = \(\frac{v}{u}\) ⇒ v = 3u

or 15 × 4 = 60 = 45 ⇒ u = 20 cm.

Case II: Image may be virtual then v is – ve.

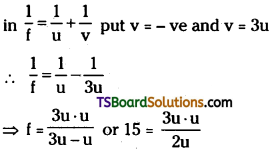
∴ 15 × 2 = 2u ⇒ u = 10 cm
The two positions of object are 10 cm, 20 cm.

Question 10.
When using a concave mirror, the magnification is found to be 4 times as much when the object is 25 cm from the mirror as it is with the object at 40 cm from the mirror, the image being real in each case. What is the focal length of the mirror?
Answer:
From given data, u₁ = 40 cm, u₂ = 25 cm

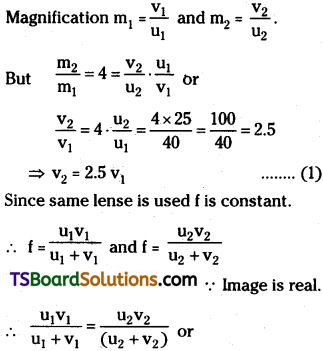
u₁v₁ (u₂ + v₂) = u₂v₂ (u₁ + v₁) ………… (2)
Put v₂ = 2.5 v₁ in equation (2)
∴ 40 v₁ (25 + 2.5 v₁) = 25 × 2.5 v₁ (40 + v₁)
1000 + 100 v₁ = 2500 + 62.5 v₁
⇒ (100 – 62.5) ₁ = 2500 – 1000
⇒ 37.5 ₁ = 1500 (or)

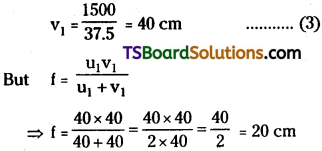
∴ Focal length of concave mirror f = 20 cm.

Question 11.
The focal length of the objective and eyepiece of a compound microscope are 4 cm and 6 cm respectively. If an object is placed at a distance of 6 cm from the objective, what is the magnification produced by the microscope?
Answer:
Focal length of objective (f₀) = 4 cm,
Focal length of eye lens (f_e) = 6 cm.
Object distance (u) = 6 cm.

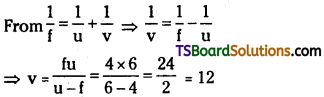
In microscope generally final image is a virtual image at near point. So magnification of eye piece m_e = (l+D/f_e)
Total magnification of microscope

Intext Question And Answer

Question 1.
A small candle, 2.5 cm in size is placed at 27 cm in front of a concave mirror of radius of curvature 36 cm. At what distance from the mirror should a screen be placed in order to obtain a sharp image? Describe the nature and size of the image. If the candle is moved closer to the mirror, how would the screen have to be moved?
Answer:
Size of the candle (h) = 2.5 cm ;
Image size = h’
Object distance (u)= – 27 cm
Radius of curvature of the concave mirror (R) = – 36 cm
Focal length of the concave mirror,
f = \(\frac{R}{2}\) = – 18 cm;
Image distance = v
The image distance can be obtained using the mirror formula : \(\frac{1}{u}+\frac{1}{v}=\frac{1}{f}\)

∴ v = -54 cm

Therefore, the screen should be placed 54 cm away from the mirror to obtain a sharp image.

The magnification of the image is given as:

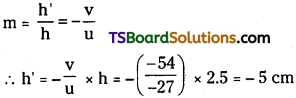
The height of the candle’s image is 5 cm. The negative sign indicates that the image is inverted and real.

If the candle is moved closer to the mirror, then the screen will have to be moved away from the mirror in order to obtain the image.

Question 2.
Double-convex lenses are to be manufactured from a glass of refractive index 1.55, with both faces of the same radius of curvature. What is the radius of curvature required if the focal length is to be 20 cm?
Answer:
Refractive index of glass (µ) = 1.55; Focal length of the double-convex lens, (f) = 20 cm Radius of curvature of one face of the lens = R₁
Radius of curvature of the other face of the lens = R₂
Radius of curvature of the double-convex lens = R
∴ R₁ = R and R₂ = – R
The value of R can be calculated as:

∴ R = 0.55 × 2 × 20 = 22 cm.
Hence, the radius of curvature of the double-convex lens is 22 cm.

Question 3.
A beam of light converges at a point P. Now a lens is placed in the path of the convergent beam 12 cm from P. At what point does the beam converge if the lens is (a) a convex lens of focal length 20 cm, and (b) a concave lens of focal length 16cm?
Answer:
In the given situation, the object is virtual and the image formed is real.
Object distance (u) = +12 cm
(a) Focal length of the convex lens, (f) = 20 cm Image distance = v
According to the lens formula, we have the relation:

Hence the image is formed 7.5 cm away from the lens, toward its right.

(b) Focal length of the concave lens (f) = -16 cm Image distance = v
According to the lens formula, we have the relation:

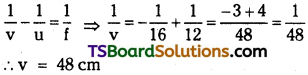
Hence, the image is formed 48 cm away from the lens, toward its right.

Question 4.
An object of size 3,0 cm is placed 14 cm in front of a concave lens of focal length 21 cm. Describe the image produced by the lens. What happens if the object is moved further away from the lens?
Answer:
Size of the object (h₁) = 3 cm;
Object distance (u) = – 14 cm
Focal length of the concave lens, (f) = – 21 cm;
Image distance = v
According to the lens formula, we have the relation :

Hence, the image is formed on the other side of the lens, 8.4 cm away from it. The negative sign shows that the image is erect and virtual.

The magnification of the image is given as:

Hence, the height of the image is 1.8 cm
If the object is moved further away from the lens, then the virtual image will move toward the focus of the lens, but not beyond it. The size of the image will decrease with the increase in the object distance.

Question 5.
What is the focal length of a convex lens of focal length 30 cm in contact with a concave lens of fqcal length 20 cm? Is the system a converging or a diverging lens? Ignore thickness of the lenses.
Answer:
Focal length of the convex lens (f₁) = 30 cm;
Focal length of the concave lens (f₂) = – 20 cm
Focal length of the system of lenses = f
The equivalent focal length of a system of two lenses in contact is given as :

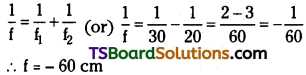
Hence, the focal length of the combination of lenses is 60 cm. The negative sign indicates that the system of lenses acts as a diverging lens.

Question 6.
A small telescope has an objective lens of focal length 144 cm and an eyepiece of focal length 6.0 cm. What is the magnifying power of the telescope? What is the separation between the objective and the eyepiece?
Answer:
Focal length of the objective lens (f₀) = 144 cm
Focal length of the eyepiece (f_e) = 6.0 cm
The magnifying power of the telescope is given as : m = \(\frac{f_0}{f_e}=\frac{144}{6}\) = 24

The separation between the objective lens and the eyepiece is calculated as :
f₀ + f_e = 144 + 6 = 150 cm
Hence, the magnifying power of the telescope is 24 and the separation between the objective lens and the eyepiece is 150 cm.

Question 7.
A small pin fixed on a table top is viewed from above from a distance of 50 cm. By what distance would the pin appear to be raised if it is viewed from the same point through a 15 cm thick glass slab held parallel to the table? Refractive index of glass = 1.5. Does the answer depend on the location of the slab?
Answer:
Actual depth of the pin (d) = 15 cm
Apparent depth of the pin = d’
Refractive index of glass, (µ) = 1.5
Ratio of actual depth to the apparent depth is equal to the refractive index of glass, i.e.,
µ = \(\frac{d}{d’}\) ∴ d’ = \({\frac{d}{\mu}}=\frac{15}{1.5}\) = 10 cm.

The distance at which the pin appears to be raised = d’ – d = 15 – 10 = 5 cm.

For a small angle of incidence, this dis¬tance does not depend upon the location of the slab.

Question 8.
The image of a small electric bulb fixec on the wall of a room is to be obtained on the opposite wall 3 in away by means of a large convex lens. What is the maximum possible focal length of the lens required for the purpose?
Answer:
Distance between the object and the image (d) = 3 m
Maximum focal length of the convex lens = f_max
For real images, the maximum focal length is given as:
m_max = \(\frac{d}{4}=\frac{3}{4}\) = 0.75 m
Hence, for the required purpose, the maximum possible focal length of the convex lens is 0.75 m.

Question 9.
A screen is placed 90 cm from an object. The image of the object on the screen is formed by a convex lens at two different locations separated by 20 cm. Determine the focal length of the lens.
Answer:
Distance between the image (screen) and the object (D) = 90 cm
Distance between two locations of the convex lens (d) = 20 cm
Focal length of the lens = f
Focal length is related to d and D as :

Therefore, the focal length of the convex lens is 21.39 cm.

Question 10.
At what angle should a ray of light be incident on the face of a prism of refracting angle 60° so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.
Answer:
The incident, refracted, and emergent rays associated with a glass prism ABC are shown in the given figure.

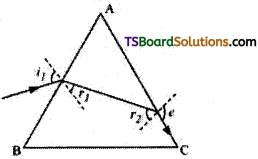
Angle of prism, ∠A = 60°;
Refractive index of the prism, µ = 1.524
i₁ = Incident angle;
r₁ = Refracted angle;
r₂ = Angle of incidence at the face AC
e = Emergent angle = 90°
According to Snell’s law, for face AC, we can have:

It is clear from the figure that angle
A = r₁ + r₂
∴ r₁ A – r₂ = 60 – 41 = 19°
According to Snell’s law, we have the relation:
\(\frac{\sin i_1}{\sin r_1}\)
⇒ sin i₁ = µ sin r₁ = 1.524 × sin 19° = 0.496
∴ i₁ = 29.75°
Hence, the angle of incidence is 29.75°.

Question 11.
You are given prisms made of crown glass and flint glass with a wide variety of angles. Suggest a combination of prisms which will
a) deviate a pencil of white light without much dispersion,
b) disperse (and displace) a pencil of white light without much deviation.
Answer:
a) Place the two prisms beside each other. Make sure that their bases are on the opposite sides of the incident white light, with their faces touching each other. When the white light is incident on the first prism, it will get dispersed. When this dispersed light is incident on the second prism, it will recombine and white light will emerge from the combination of the two prisms.

b) Take the system of the two prisms as suggested in answer (a). Adjust (increase) the angle of the flint-glass-prism so that the deviations due to the combination of the prisms become equal. This combination will disperse the pencil of white light without much deviation.

Question 12.
Does short sightedness (myopia) or long-sightedness (hypermetropia) imply necessarily that the eye has partially lost its ability of accommodation? If not, what might cause these defects of vision?
Answer:
A myopic or hypermetropic person can also possess the normal ability of accommodation of the eye-lens. Myopia occurs when the eye-balls get elongated from front to back. Hypermetropia occurs when the eye-balls get shortened. When the eye-lens loses its ability of accommodation, the defect is called presbyopia.

Question 13.
A myopic person has been using spectacles of power – 1.0 dioptre for distant vision. During old age he also needs to use separate reading glass of power + 2.0 dioptres. Explain what may have happened.
Answer:
The power of the spectacles used by the myopic person (P) = – 1.0 D
Focal length of the spectacles,
f = \(\frac{1}{P}=\frac{1}{-1 \times 10^{-2}}\) = – 100cm

Hence, the far point of the person is 100 cm. He might have a normal near point of 25 cm. When he uses the spectacles, the objects placed at infinity produce virtual images at 100 cm. He uses the ability of accommodation of the eye-lens to see the objects placed between 100 cm and 25 cm. During old age, the person uses reading glasses of power, P’ = + 2D.

The ability of accommodation is lost in old age. This defect is called presbyopia. As a result, he is unable to see clearly the objects placed at 25 cm.

Question 14.
A person looking at a person wearing a shirt with a pattern comprising vertical and horizontal lines is able to see the vertical lines more distinctly than the horizontal ones. What is this defect due to? How is such a defect of vision corrected?
Answer:
In the given case, the person is able to see vertical lines more distincfly than horizontal lines. This means that the refracting system (cornea and eye-lens) of the eye is not working in the same way in different planes. This defect is called astigmatism. The person’s eye has enough curvature in the vertical plane. However, the curvature in the horizontal plane is insufficient. Hence, sharp images of the vertical lines are formed on the retina, but horizontal lines appear blurred. This defect can be corrected by using cylindrical lenses.

Question 15.
a) At what distance should the lens be held from the figure in Exercise 2.29 in order to view the squares distinctly with the maximum possible magnifying power?
b) What is the magnification in this case?
c) Is the magnification equal to the magnifying power in this case? Explain.
Answer:
a) The maximum possible magnification is obtained when the image is formed at the near point (d = 25 cm).
Image distance, υ = – d = – 25 cm; Focal length, f= 10 cm; Object distance = u
According to the lens formula, we have:

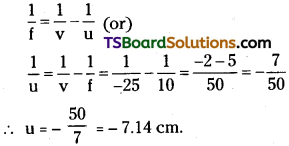
Hence, to view the squares distinctly, the lens should be kept 7.14 cm away from them.

Since the image is formed at the near point (25 cm), the magnifying power is equal to the magnitude of magnification.