TS Inter 1st Year Physics Study Material Chapter 5 Laws of Motion

Telangana TSBIE TS Inter 1st Year Physics Study Material 5th Lesson Laws of Motion Textbook Questions and Answers.

TS Inter 1st Year Physics Study Material 5th Lesson Laws of Motion

Very Short Answer Type Questions

Question 1.
Why are spokes provided in a bicycle wheel? [AP May ’14. ’13]
Answer:
The spokes of cycle wheel increase its moment of inertia. The greater the moment of inertia, the greater is the opposition to any change in uniform rotational motion. As a result the cycle runs smoother and steadier. If the cycle wheel had no spokes, the cycle would be driven with jerks and hence unsafe.

Question 2.
What is inertia? What gives the measure of inertia? [TS ‘Mar. 17; AP Mar. 19, 14]
Answer:
The inability of a body to change its state by itself is known as inertia.

Mass of a body is a measure for its Inertia.
Types of inertia

1. Inertia of rest
2. Inertia of motion
3. Inertia of direction.

Question 3.
According to Newton’s third law, every force is accompanied by an equal and opposite force. How can a movement ever take place? [AP May 17, June 15]
Answer:
From Newton’s third law action = – reaction. But action and reaction are not working on the same system. So they will not cancel each other. Hence, motion is possible.

Question 4.
When a bullet is fired from a gun, the gun gives a kick in the backward direction. Explain. [AP Mar. ’15]
Answer:
Firing of a gun is due to internal forces. Internal forces do not change the momentum of the system. Before firing m₁u₁ + m₂u₂ = 0. Since system is at rest after firing m₁v₁ + m₂v₂ = 0 (or) m₁v₁ = – m₂v₂. So gun and bullet will move in opposite directions to satisfy law of conservation of linear momentum.

Question 5.
Why does a heavy rifle not recoil as strongly as a light rifle using the same cartridges?
Answer:
Velocity (or) recoil v = \(\frac{mv}{M}\) i.e., ratio of momentum of bullet to mass of gun. If mass of gun is high then velocity of recoil is less with same cartridge.

Question 6.
If a bomb at rest explodes into two pieces, the pieces must travel in opposite directions. Explain. [TS Mar. 16, 15, June 15]
Answer:
Explosion is due to internal forces. From law of conservation of linear momentum, internal forces cannot change the momentum of the system. So after explosion m₁v₁ + m₂v₂ = 0 (or) m₁v₁ = – m₂v₂. According to law of conservation of linear momentum they will fly in opposite directions.

Question 7.
Define force. What are the basic forces in nature?
Answer:
Force is that which changes (or) tries to change the state of a body.

The basic forces in nature are :

1. Gravitational forces,
2. Electromagnetic forces,
3. Nuclear forces.

Question 8.
Can the coefficient of friction be greater than one?
Answer:
Yes. Generally coefficient of friction between the surfaces is always less than one. But under some special conditions like on extreme rough surfaces coefficient of friction may be greater than one.

Question 9.
Why does the car with a flattened tyre stop sooner than the one with inflated tyres?
Answer:
Due to flattening of tyres, frictional force increases. Because rolling frictional force between the surfaces is proportional to area of contact. Area of contact increases for flattened tyres. So rolling frictional force increases and the car will be stopped quickly.

Question 10.
A horse has to pull harder during the start of the motion than later. Explain. [AP Mar. 18, May 16, Mar. 13]
Answer:
To start motion in a body we must apply force to overcome static friction (F_s = µ_smg). When once motion is started between the bodies then kinetic frictional force comes into act. Kinetic friction (F_k = µ_kmg) is always less than static friction. So it is tough to start a body from rest than to keep it in motion.

Question 11.
WHat happens to the coefficient of friction if the weight of the body is doubled? [TS Mar. 19; AP Mar. 16, May 14]
Answer:
When weight of the body is doubled still then there is no change in coefficient of friction. Because frictional force cc normal reaction. So when weight of a body is doubled then frictional force and normal reaction will also becomes doubled and coefficient of friction remains constant.

Short Answer Questions

Question 1.
A stone of mass 0.1 kg is thrown vertically upwards. Give the magnitude and direction of the net force on the stone (a) during its upward motion, (b) during its downward motion, (c) at the highest point, where it momentarily comes to rest.
Answer:
Mass of stone, m = 0.1 kg.
a) During upward motion force acts downwards due to acceleration due to gravity.
Magnitude of force F = mg = 0.1 × 9.8
= 0.98 N (↓)
b) During downward motion force acts downward. Magnitude of force F = mg
= 0.1 × 9.8 = 0.98N (↓)

c) At highest point velocity v = 0. But still g will act on it only in downward motion so resultant force F = 0.98 N. downward.
Note : In the entire journey of the body force due to gravitational pull acts only in downward direction.

d) If the body is thrown with an angle of 30° with horizontal then vertical component of gravitational force does not change, hence in this case downward force F = mg = 0.1 × 9.8
= 0. 98 newton.

Question 2.
Define the terms momentum and impulse. State and explain the law of conservation of linear momentum. Give examples. [TS May 18, June 15]
Answer:
Momentum (\(\overline{\mathrm{P}}\)) : It is the product of mass and velocity of a body.

Momentum (\(\overline{\mathrm{P}}\)) = mass (m) × velocity (v)
∴ (\(\overline{\mathrm{P}}\)) = m\(\overline{\mathrm{v}}\)

Impulse (J) :
When a large force (F) acts on a body for small time (t) then the product of force and time is called Impulse.
Impulse (J) = Force (F) × time (t)
∴ Impulse (J) = F × t

Law of conservation of linear momentum:
There is no external force act on the system. The total linear momentum of the isolated system remains constant.

Proof :
Let two bodies of masses say A and B are moving with initial momenta PA and PB collided with each other. During collision they are in contact for a small time say ∆t. During this time of contact they will exchange their momenta. Let final momenta of the bodies are P¹_A and P¹_B. Let force applied by A on B is F_AB and force applied by B on A is F_BA.

From Newton’s 3rd Law F_AB = F_BA or F_AB ∆t = F_BA ∆t

From 2nd Law F_AB∆t = P¹_A – P_A change in momentum of A.
F_BA ∆t = P¹_B – P_B change in momentum of B.
∴ P¹_B – P_A = P¹_B – P_B or P_A + P_B = P¹_B + P¹_B
i. e., sum of momentum before collision is equals to sum of momentum after collision.

Question 3.
Why are shock absorbers used in motor cycles and cars? [AP June ’15]
Answer:
When vehicles are passing over the vertocies and depressions of a rough road they will collides with them for a very short period. This causes impulse effect. Due to large mass and high speed of the vehicles the magnitude of impulse is also high. Impulse may cause damage to the car or even to the passengers in it.

The bad effects of impulse is less if time of contact is more. Impulse J = F.t. For the same magnitude of impulse (change in momentum) if time of contact is high force acting on the vehicle is less. Shock absorbers will absorb the impulse and releases the same force slowly. This is due to large time constant of the springs.

So shock absorbers are used in vehicles to reduce impulse effects.

Question 4.
Explain the terms limiting friction, dynamic friction and rolling friction.
Answer:
Limiting friction :
Frictional forces always oppose relative motion between the bodies. These forces are self adjusting forces. Their magnitude will increase upto some extent with the value of applied force.

The maximum frictional force between the bodies at rest is called “limiting friction”.

Dynamic (or) kinetic friction :
When applied force is equal to or greater than limiting friction then the body will move. When once motion is started then frictional , force will abruptly falls to a minimum value.

Frictional force between moving bodies is called dynamic (or) kinetic friction. Kinetic friction is always less than limiting friction.

Rolling friction :
The resistance encountered by a rolling body on a surface is called rolling friction.

Question 5.
Explain the advantages and disadvantages of friction. [TS Mar. ’17, ’15; AF Mar. ’15]
Answer:
Advantages of friction :

1. We are able to walk because of friction.
2. It is impossible for a car to move on a slippery road.
3. Breaking system of vehicles works with the help of friction.
4. Friction between roads and tyres provides the necessary external force to accelerate the car.
5. Transmission of power to various parts of a machine through belts is possible by friction.

Disadvantages of friction:

1. In many cases we will try to reduce friction because it dissipates energy into heat.
2. It causes wear and tear to machine parts which causes frequent replacement of machine parts.

Question 6.
Explain Friction. Mention the methods used to decrease friction. [TS May, ’17, ’16; Mar. ’19, ’16; AP Mar. ’18. ’14; May ’18, ’14)
Answer:
Friction :
It is a contact force parallel to the surfaces in contact Friction will always oppose relative motion between the bodies.

Friction is a necessary evil. Friction is a must at some places and it must be reduced at some places.

Methods to reduce friction :
1) Polishing :
Friction causes due to surface irregularities. So by polishing friction can be reduced to some extent.

2) Lubricants :
By using lubricants friction can be reduced. Lubricants will spread as an ultra thin layer between the surfaces in contact and in friction decreases.

3) A thin cushion of air maintained between solid surfaces reduces friction.
Ex : Air pressure in tyres.

4) Ball bearings :
Ball bearings are used to reduce friction between machine parts.

Ball bearings will convert sliding motion into rolling motion. As a result friction is reduced.

Question 7.
State the laws of rolling friction.
Answer:
When a body is rolling over the other, then friction between the bodies is known as rolling friction.

Rolling friction coefficient,

Laws of rolling friction :

1. Rolling friction will develop a point contact between the surface and the rolling sphere. For objects like wheels line of contact will develop.
2. Rolling friction(f_r) has least value for given normal reaction when compared with static friction (f_s) or kinetic friction (f_k)
3. Rolling friction is directly proportional
   to normal reaction, f_r ∝ N.
4. In rolling friction the surfaces in contact will get momentarily deformed a little.
5. Rolling friction depends on area of contact. Due to this reason friction increases when air pressure is less in tyres (Flattened tyres).
6. Rolling friction is inversely proportional to radius of rolling body µ_r ∝ \(\frac{1}{r}\)

Question 8.
Why is pulling the lawn roller preferred to pushing it?
Answer:
Let a lawn roller is pulled by means of a force F with some angle θ to the horizontal. By resolving the force into two components.

1. Horizontal component F cos θ is useful to pull the body.
2. The vertical component F sin θ opposes the weight

So N.R. = mg – F sin θ
But frictional force = µ. N.R.
∴ Frictional force [µ(mg – F sin θ)] decreases.

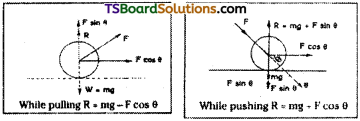
So it is easier to pull the body.
When the lawn roller is pushed by a force, the vertical component F sin θ causes the apparent increase of weight of the object. So the normal reaction N.R. = mg + F sin θ.
∴ Frictional force [µ(mg + F sin θ)] increases and it will be difficult to pull the body.

Long Answer Questions

Question  1.
a) State Newton’s second law of motion. Hence, derive the equation of motion F = ma from it. [AP Mar. ’19, ’17, ’16; AP May ’17. ’16; May ’13]
b) A body is moving along a circular path such that its speed always remains constant. Should there be a force acting on the body?
Answer:
a) Newton’s 2nd law :
The rate of change of momentum of a body is proportional to external force and acts along the direction of force applied.
i.e., \(\frac{dp}{dt}\) ∝ F

Derivation of equation F = ma:
According Newton’s 2nd law.
We know


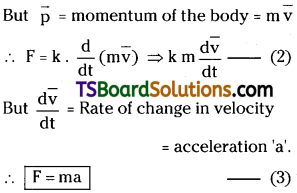
Here, k = constant.
The proportional constant is made equal to one, by properly selecting the unit of force.
∴ F = ma

b) Force on a body moving in a circular path :
Let a body of mass’m’ is moving in a circular path of radius V with constant speed. The velocity of the body is given by the tangent drawn at that point. Since velocity is changing continuously the body will have acceleration.

So the body will experience some acceleration. This is called normal acceleration (or) centripetal acceleration.

Question 2.
Define Angle of friction and Angle of repose. Show that angle of friction is equal to angle of repose for a rough inclined plane.
A block of mass 4 kg is resting on a rough horizontal plane and is about to move when a horizontal force of 30 N is applied on it. If g = 10 m/s². Find the total contact force exerted by the plane on the block.
Answer:
Angle of friction :
The angle made by the resultant of the Normal reaction and the limiting friction with Normal reaction is called angle of friction (Φ).

Angle of reppse :
Let a body of mass m is placed on a rough inclined plane. Let the angle with the horizontal ‘θ’ is gradually increased then fora particular angle of inclination (say α) the body will just slide down without acceleration. This angle θ = α is called angle of repose. At this stage the forces acting on the body are in equilibrium.

Equation for angle of repose :
Force acting on the body in vertically downward direction = W = mg.
By resolving this force into two components.

1. Force acting along the inclined plane in downward direction = mg sin θ.
   This component is responsible for downward motion.
2. The component mg cos θ . which is balanced by the normal reaction.

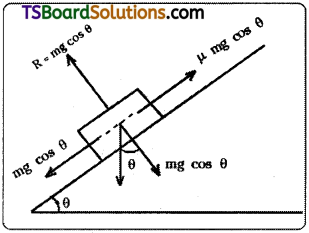
If the body slides down without acceleration resultant force on the body is zero, then
mg sin θ = Frictional force (f_k)
mg cos θ = Normal reaction (N.R.)
But coefficient friction

Hence θ = α is called angle of repose.
∴ µ_k = tan α

Hence tangent of angle of repose (tan θ) is equal to coefficient of friction (f_k) between the bodies.

b) When the block rests on the horizontal surface, it is in equilibrium under the action of four forces. They are
i) Normal reaction (N)
ii) Weight of the block (mg)
iii) Horizontal force (30 N)
iv) Limiting frictional force (f_L)

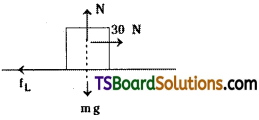
If the applied horizontal force is equal to the limiting frictional force, then only the block will be ready to move on the rough horizontal surface, i.e., f_L = horizontal force applied.
∴ Total contact force = 30 N.

Problems

Question 1.
The linear momentum of a particle as a function of time ‘t’ is given by, p = a + bt, where a and b are positive constants. What is the force acting on the particle?
Solution:
Linear momentum of a particle, p = a + bt
We know that force acting on a particle is equal to rate of change of linear momentum.

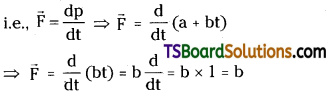
Question 2.
Calculate the time needed for a net force of 5 N to change the velocity of a 10 kg mass by 2 m/s. [TS May ’16]
Solution:
Force, F = 5N
Change in velocity, v – u = 2ms ^-1
Mass, m= 10 kg
From Newton’s second law of motion,

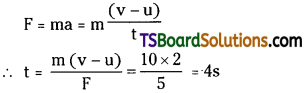
Question 3.
A ball of mass ‘m’ is thrown vertically upward from the ground and reaches a height ‘h’ before momentarily coming to rest, If ‘g’ is acceleration due to gravity. What is the impulse received by the ball due to gravity force during its flight?
Solution:
Impulse, J = force × time

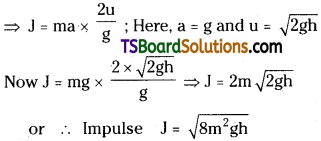
Question 4.
A constant force acting on a body of mass 3.0 kg changes its speed from 2.0 m s^-1 to 3.5 m s^-1 in 25 s. The direction of motion of the body remains unchanged. What is the magnitude and direction of the force?
Solution:
Mass of the body, m = 3.0 kg ;
Initial velocity of the body, u = 2.0 ms^-1
Final velocity of the body, v = 3.5 ms^-1
Time, t = 25 s
From Newton s second law of motion,

∴ Magnitude of force acting on the body, F = 0.18 N. The direction of force acting on the body is along the direction of motion of the body because force is positive.

Question 5.
A man in a lift feels an apparent weight ‘W’ when the lift is moving up with a uniform acceleration of 1/3^rd of the acceleration due to gravity. If the same man was in the same lift now moving down with a uniform acceleration that is 1/2 of the acceleration due to gravity, then what is his apparent weight?
Solution:
Case (i) :
When lift is moving upwards :
Apparent weight of the man = W
Acceleration, a = g/3
Apparent weight of the man when the lift is moving upwards is,

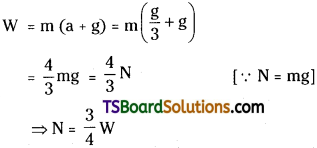
Case (ii) : When lift is moving downwards:
Let W’ be the apparent weight of the man Acceleration, a = g/2
Apparent weight of the man when the lift is moving downwards is,
W’ = m(g – a) = m (g – g/2)

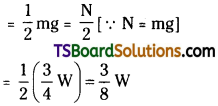
Question 6.
A container of mass 200 kg rests on the back of an open truck. If the truck accelerates at 1.5 m/s², what is the minimum coefficient of static friction between the container and the bed of the truck required to prevent the container from sliding off the back of the truck?
Solution:
Mass of the container, m = 200 kg
Acceleration of truck, a = 1.5 ms^-2
Coefficient of static friction, µ_s = \(\frac{a}{g}\)
\(\frac{1.5}{9.8}\) = 0.153

Question 7.
A bomb initially at rest at a height of 40 m above the ground suddenly explodes into two identical fragments. One of them starts moving vertically downwards with an initial speed of 10m/s. If acceleration due to gravity is 10m/s², What is the separation between the fragments 2s after the explosion?
Solution:
Case (i): (for downward moving fragment)
Initial velocity, u = 10 ms^-1
Acceleration, a = +g = 10 ms^-2
Time, t = 2s
From the equation of motion, s = ut + \(\frac{1}{2}\) at²
the distance moved in downward direction is,
s₁ = 10 × 2 + \(\frac{1}{2}\) × 10 × (2)² = 40 m

Case (ii) (for upward moving fragment)
Given that two fragments are identical hence, after explosion the fragments move in opposite directions. Here the first fragment moves in downward direction, hence, second fragment moves upward direction.
Again from s = ut = \(\frac{1}{2}\)at² we can write,
s₂ = – 10 × 2 + \(\frac{1}{2}\) × 10 × 4 = -20 + 20 = 0m
∴ Separation between the fragments 2s after the explosion = S₁ ~ S₂ = 40 – 0 = 40m

Question 8.
A fixed pulley with a smooth grove has a light string passing over it with a 4 kg attached on one side and a 3 kg on the other side. Another 3 kg is hung from the other 3 kg as shown with another light string. If the system is released from rest, find the common acceleration? (g = 10 m/s²)

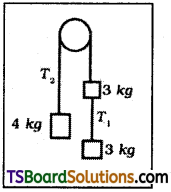
Solution:
Here, m₁ = 3 + 3 = 6 kg; m₂ = 4 kg ;
g = 10 ms^-2

Acceleration of the system,

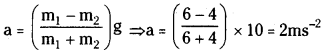
Question 9.
A block of mass of 2 kg slides on an inclined plane that makes an angle of 30° with the horizontal. The coefficient of friction between the block and the surface is √3/2.
a) What force should be applied to the block so that it moves down without any acceleration?
b) What force should be applied to the block so that it moves up without any acceleration?
Solution:
Mass of the block, m = 2kg
Angle of inclination, θ = 30°
Coefficient of friction between the block and the surface, µ = \(\frac{\sqrt{3}}{2}\)

a) The required force to move the block down without acceleration is,

b) The required force to move the block up without any acceleration is,

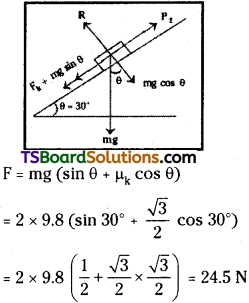
Question 10.
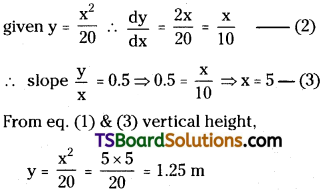
A block is placed on a ramp of parabolic shape given by the equation y = x²/20, sec Figure.

If µ_s = 0.5, what is the maximum height above the ground at which the block can be placed without slipping?
(tan θ = µ_s = \(\frac{dy}{dx}\))
Solution:
For the body not to drop
mg cos θ = µ mg sin θ
⇒ tan θ = µ given µ = 0.5 dy
But tan θ = \(\frac{dy}{dx}\) slope of parabolic region
⇒ \(\frac{dy}{dx}\) = µ = 0.5 …………… (1)

Question 11.
A block of metal of mass 2 kg on a horizontal table is attached to a mass of 0.45 kg by a light string passing over a frictionless pulley at the edge of the table. The block is subjected to a horizontal force by allowing the 0.45 kg mass to fall. The coefficient of sliding friction between the block and table is 0.2.
Calculate (a) the initial acceleration, (b) the tension in the string, (c) the distance the block would continue to move if, after 2 s of motion, the string should break.

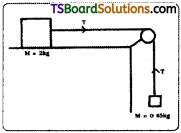
Solution:
Mass of first block, m₁ = 0.45kg
Mass of second block, m₂ = 2 kg
coefficient of slidding friction between the block and table, µ = 0.2

b) Tension in the string

c) Velocity of string after 2 sec = u in this case; u’ = 0
∴ u = u’ + at = 0 + 0.2 × 2 = 0.4 m/s

Question 12.
On a smooth horizontal surface, a block A of mass 10 kg is kept. On this block, a second block B of mass 5 kg is kept. The coefficient of friction between the two blocks is 0.4. A horizontal force of 30 N is applied on the lower block as shown. The force of friction between the blocks is (take g = 10 m/s²)

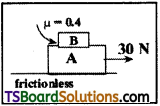
Solution:
Mass of block ‘A’ is, m_A = 10 kg
Mass of block ‘B’ is, m_B = 5 kg
Applied horizontal force, F = 30 N
Coefficient of friction between two blocks, µ = 0.4

Frictional force of block ‘B’ is f = µmg
⇒ f = 0.4 × 5 × 10 = 20N
∴ The frictional force acting between the two blocks, = F – f = 30 – 20 = 10 N

Question 13.
A batsman hits back a ball straight in the direction of the bowler without changing its initial speed of 12 ms^-1. If the mass of the ball is 0.15 kg., determine the impulse imparted to the ball. (Assume linear motion of the ball). [AP Mar. ’17]
Solution:
Impulse = change in momentum
= (0.15 × 12) – (- 0.15 × 12) = 3.6 NS
in the direction from the batsman to the bowler.

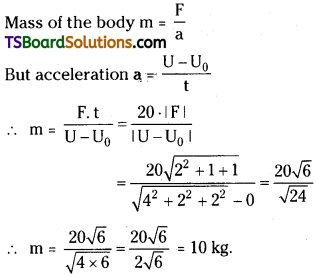
Question 14.
A force \(2\overline{\mathrm{i}}+\overline{\mathrm{j}}-\overline{\mathrm{k}}\) Newton acts on a body which is initially at rest. At the end of 20 seconds the velocity of the body is \(4\overline{\mathrm{i}}+2\overline{\mathrm{j}}-2\overline{\mathrm{k}}\) m/s. What is the mass of the body? [AP May ’16]
Answer:
Force F = \(2\overline{\mathrm{i}}+\overline{\mathrm{j}}-\overline{\mathrm{k}}\), time t = 20 sec.
Initial velocity u₀ = 0.
Final velocity U = \(4\overline{\mathrm{i}}+2\overline{\mathrm{j}}-2\overline{\mathrm{k}}\)

Additional Problems

Question 1.
A constant retarding force of 50 N is applied to a body of mass 20 kg moving initially with a speed of 15 ms-1. How long does the body take to stop?
Solution:
Here, F = – 50N, m = 20 kg
u = 15 ms^-1, v = 0, t = ?

Question 2.
A body of mass 5 kg is acted upon by two perpendicular forces 8 N and 6 N. Give the magnitude and direction of the acceleration of the body.
Solution:

This is the direction of resultant force and hence the direction of acceleration of the body as shown in figure.

Question 3.
The driver of a three-wheeler moving with a speed of 36 km / h sees a child standing in the middle of the road and brings his vehicle to rest in 4.0 s just in time to save the child. What is the average retarding force on the vehicle ? The mass of the three wheeler is 400 kg and the mass of the driver is 65 kg.
Solution:
Here, u = 36 km/h = 10 m/s, v = 0, t = 4s
m = 400 + 65 = 465 kg

Question 4.
A rocket with a lift-off mass 20,000 kg is blasted upwards with an initial acceleration of 5.0 ms^-2. Calculate the initial thrust (force) of the blast.
Solution:
Here, m = 20000 kg = 2 × 10⁴ kg
Initial acceleration, a = 5 ms^-2;
Thrust, F = ?

Clearly, the thrust should be such that it overcomes the force of gravity besides giving it an upward acceleration of 5 ms^-2.

Thus the force should produce a net acceleration of 9.8 + 5.0 = 14.8 ms^-2.
As thrust = force = mass × acceleration
∴ F = 2 × 10⁴ × 14.8 = 2.96 × 10⁵N

Question 5.
A man of mass 70 kg stands on a weighing scale in a lift which is moving
a) upwards with a uniform speed of 10 ms^-1,
b) downwards with a uniform acceleration of 5 ms^-2,
c) upwards with a uniform acceleration of 5 ms^-2,
What would be the readings on the scale in each case?
d) What would be the reading if the lift mechanism failed and it hurtled down freely under gravity?
Solution:
Here, m = 70 kg, g = 10 m/s²
The weighing machine in each case measures the reaction R i.e., the apparent weight.
a) When the lift moves upwards with a uniform speed, its acceleration is zero.
R = mg = 70 × 10 = 700 N

b) When the lift moves downwards with a = 5 ms^-2
R = m(g – a) = 70 (10 – 5) = 350 N

c) When the lift moves upwards with a = 5 ms^-2
R = m (g + a) = 70 (10 + 5) = 1050 N

d) If the lift were to come down freely under gravity, downward acceleration. a = g
∴ R = m (g – a) = m (g – g) = Zero.

Question 6.
Two bodies of masses 10 kg and 20 kg respectively kept on a smooth, horizontal surface are tied to the ends of a light string, a horizontal force F = 600 N is applied to (i) A, (ii) B along the direction of string. What is the tension in the string in each case?
Solution:
Here, F = 600 N m₁= 10 kg, m2 = 20 kg

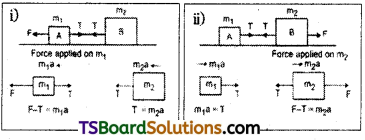
Let T be the tension in the string and a be the acceleration of the system, in the direction of force applied.
∴ a = \(\frac{F}{m_1+m_2}=frac{600}{10+20}\) = 20 m/s²

i) When force is applied on lighter block A, Fig (i).
T = m₂ a = 20 × 20 N’= 400 N

ii) When force is applied on heavier block B, Fig (ii).
T = m₁a = 10 × 20 NT = 200 N
Which is different from value of T in case (i). Hence our answer depends on which mass end, the force is applied.

Question 7.
Two masses 8 kg and 12 kg are connected at the two ends of a light in extensible string that goes over a frictionless pulley. Find the acceleration of the masses, and the tension in the string when the masses are released.

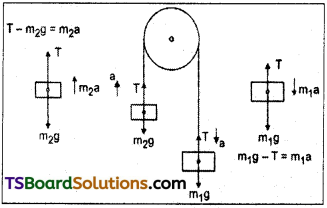
Solution:
Here, m₂ = 8kg, ; m₁ = 12 kg

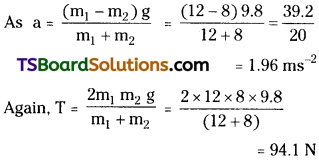
Question 8.
A nucleus is at rest in the laboratory frame of reference. Show that if it disintegrates into two smaller nuclei the products must move in opposite directions.
Solution:
Let m₁, m₂ be the masses of products and \(\overrightarrow{\mathrm{v_1}},\overrightarrow{\mathrm{v_2}}\) be their respective velocities. Therfore, total linear momentum after disintegration = \(m_1\overrightarrow{\mathrm{v_1}}+m_2\overrightarrow{\mathrm{v_2}}\). Before disintegra-tion, the nucleus is at rest. Therefore, its linear momentum before disintegration is zero.

According to the principle of conservation of linear momentum,

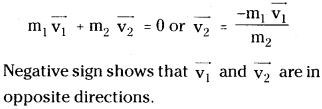
Question 9.
Two billiard balls each of mass 0.05 kg moving in opposite directions with speed 6 ms^-1 collide and rebound with the same speed. What is the impulse imparted to each ball due to the other?
Solution:
Here, initial momentum of the ball
A = 0.05 (6) = 0.3 kg ms^-1

As the speed is reversed on collision, final momentum of the ball A = 0.05 (-6)
= – 0.3 kg ms^-1

Impulse imparted to ball A = change in momentum of ball A = final momentum – initial momentum = – 0.3 – 0.3 = – 0.6 kg ms^-1.

Question 10.
A shell of mass 0.02 kg is fired by a gun of mass 100 kg. If the muzzle speed of the shell is 80 ms^-1, what is the recoil speed of the gun?
Solution:
Here, mass of shell, m = 0.02 kg
mass of gun, M = 100 kg
muzzle speed of shell, V = 80 ms^-1
recoil speed of gun, v = ?
According to the principle of conservation of linear momentum, mV + Mυ = 0

Question 11.
A stone of mass 0.25 kg tied to the end of a string is whirled round in a circle of radius 1.5 m with a speed of 40 rev./min in a horizontal plane. What is the tension in the string? What is the maximum speed with which the stone can be whirled around if the string can withstand a maximum tension of 200 N?
Solution:
Here, m = 0.25 kg, r = 1.5 m ;

Question 12.
Explain why
a) a horse cannot pull a cart and run in empty space,
b) passengers are thrown forward from their seats when a speeding bus stops suddenly,
c) it is easier to pull a lawn mover than to push it,
d) a cricketer moves his hands backwards while holding a catch.
Solution:
a) While trying to pull a cart, a horse pushes the ground backwards with a certain force at an angle. The ground offers an equal reaction in the opposite direction, on the feet of the horse. The forward component of this reaction is responsible for motion of the cart. In empty space, there is no reaction and hence, a horse cannot pull the cart and run.

b) This is due to “inertia of motion”.
When the speeding bus stops suddenly, lower part of the bodies in contact with the seats stop. The upper part of the bodies of the passengers tend to maintain the uniform motion. Hence, the passengers are thrown forward.

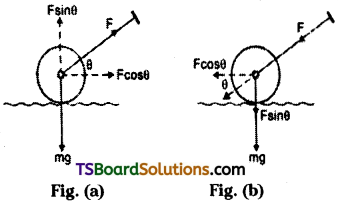
c) While pulling a lawn mover, force is applied upwards along the handle. The vertical component of this force is upwards and reduces the effective weight of the mover, Fig (a). While pushing a lawn mover, force is applied downwards along the handle. The vertical component of this force is downwards and increases the effective weight of the mover, Fig (b). As the effective weight is lesser in case of pulling than in case of pushing, therefore, “pulling is easier than pushing”.

d) While holding a catch, the impulse received by the hands, F × t = change in linear momentum of the ball is constant. By moving his hands backwards, the cricketer increases the time of impact (t) to complete the catch. As t increases, F decreases and as a reaction, his hands are not hurt severely.

Question 13.
A stream of water flowing horizontally with a speed of 15 ms^-1 pushes out of a tube of cross-sectional area 10^-2 m², and hits a vertical wall nearby. What is the force exerted on the wall by the impact of water, assuming it does not rebound?
Solution:
Here, v = 15 ms^-1
Area of cross section, a = 10^-2 m²
Volume of water pushing out/sec = a × v
= 10^-2 × 15 m³ s^-1

As density of water is 10³ kg/m³, therefore, mass of water striking the wall per sec.
m = (15 × 10^-2) × 10³ = 150 kg/s.

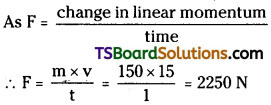
Question 14.
Ten one-rupee coins are put on top of each other on a table. Each coin has a mass m.
Give the magnitude and direction of
a) the force on the 7^th coin (counted from the bottom) due to all the coins on its top,
b) the force on the 7^th coin by the eighth coin,
c) the reaction of the 6^th coin on the 7^th coin.
Solution:
a) The force on 7^th coin is due to weight of the three coins lying above it. Therefore,
F = (3 m) kgf = (3 mg) N
where g is acceleration due to gravity. This force acts vertically downwards.

b) The eighth coin is already under the weight of two coins above it and it has its own weight too. Hence force on 7^th coin due to 8^th coin is sum of the two forces i.e.
F = 2m + m = (3m) kgf = (3 mg) N
The force acts vertically downwards.

c) The sixth coin is under the weight of four coins above it.
Reaction, r = -F = -4m (kgf) = – (4 mg) N

Minus sign indicates that the reaction acts vertically upwards, opposite to the weight.

Question 15.
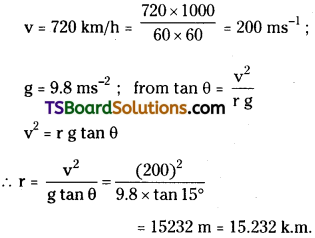
An aircraft executes a horizontal loop at a speed of 720 km/h with its wings banked at 15°. What is the radius of the loop?
Solution:
Here θ = 15°

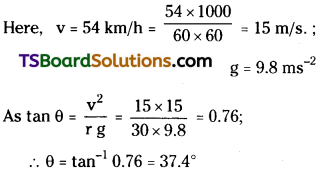
Question 16.
A train runs along an unbanked circular track of radius 30 m at a speed of 54 km/h. The mass of the train is 10⁶ kg. What provides the centripetal force required for this purpose – The engine or the rails? What is the angle of banking required to prevent wearing out of the rail?
Solution:
The centripetal force is provided by the lateral thrust exerted by the rails on the wheels. By Newton’s 3rd law, the train exerts an equal and opposite thrust on the rails causing its wear and tear.

Obviously, the outer rail will wear out faster due to the larger force exerted by the train on it.

Question 17.
A block of mass 25 kg is raised by a 50 kg man in two different ways as shown in Fig. What is the action on the floor by the man in the two cases? If the floor yields to a normal force of 700 N, which mode should the man adopt to lift the block without the floor yielding?

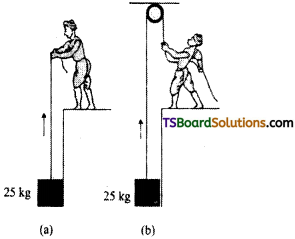
Solution:
Here, mass of block, m = 25 kg
Mass of man, M = 50 kg
Force applied to lift the block
F = mg = 25 × 9.8 = 245 N
Weight of man W = Mg = 50 × 9.8 = 490 N.

a) When block is raised by man as shown in Fig. (a), force is applied by the man in the upward direction. This increases the apparent weight of the man. Hence action on the floor.
W’ = W + F = 490 + 245 = 735 N

b) When block is raised by man as shown in Fig. (b), force is applied by the man in the downward direction. This decreases the apparent weight of the man. Hence, action on the floor in this case would be W’ = W – F = 490 – 245 = 245 N.

As the floor yields to a normal force 700 N, the mode (b) has to be adopted by the man to lift the block.

Question 18.
A monkey of mass 40 kg climbs on a rope (Fig) which can stand a maximum tension of 600 N. In which of the following cases will the rope break : the monkey {LAWS OF MOTION )
a) climbs up with an acceleration of 6 ms^-2
b) climbs down with an acceleration of 4 ms^-2
c) climbs up with a uniform speed of 5 ms^-1
d) falls down the rope nearly freely under gravity?
(Ignore the mass of the rope).
Solution:
Here, mass of monkey, m = 40 kg
Maximum tension the rope can stand, T = 600 N.
In each case, actual tension in the rope will be equal to apparent weight of monkey (R), The rope will break when R exceeds T.
a) When monkey climbs up with a = 6 ms^-2,
R = m (g + a) = 40 (10 + 6) = 640 N (which is greater than T).
Hence the rope will break.

b) When monkey climbs down with a = 4 ms^-2
R = m (g – a) = 40 (10 – 4) = 240 N, which is less than T
∴ The rope will not break.

c) When monkey climbs up with a uniform speed v = 5 ms^-1,
its acceleration a = 0 ∴ R = mg = 40 × 10 = 400 N, which is less than T
∴ The rope will not break.

d) When monkey falls down the rope nearly freely under gravity, a = g
∴ R = m (g – a) = m (g – g) = 0 (Zero.)
Hence the rope will not break.

Question 19.
A 70 kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3 in rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15. What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed?
Solution:
Here, m = 70 kg, r = 3 m
n = 200, rpm = \(\frac{200}{60}\) rps, p = 0.15, ω = ?

The horizontal force N by the wall on the man provides the necessary centripetal force = m r ω². The frictional force (f) in this case is vertically upwards opposing the weight (mg) of the man.

After the floor is removed, the man will remain stuck to the wall, when mg = f < µ N, i.e. mg < µ m r ω² or g < µ r ω²
∴ Minimum angular speed of rotation of

Question 20.
A thin circular loop of radius R rotates about its vertical diameter with an angular frequency ω. Show that a small bead on the wire loop remains at its lowermost point for to ω ≤ √g/R. What is the angle made by the radius vector joining the centre to the bead with the vertical downward direction for to ω = √2g/R? Neglect friction.
Solution:
In Figure we have shown that radius vector joining the bead to the centre of the wire makes an angle 0 with the verticle downward direction. If N is normal reaction, then as is clear from the figure,
mg = N cos θ —- (i)
m r ω² = N sin θ —- (ii)
or m (R sin θ) ω² = N sin θ or m R ω² = N
from (i), mg = m R ω² cos θ or