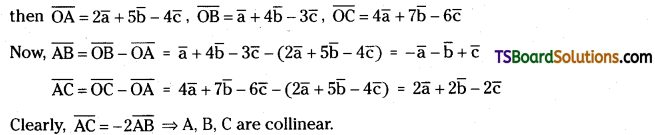TS Inter 1st Year Maths 1A Addition of Vectors Important Questions Short Answer Type

Students must practice these Maths 1A Important Questions TS Inter 1st Year Maths 1A Addition of Vectors Important Questions Short Answer Type to help strengthen their preparations for exams.

TS Inter 1st Year Maths 1A Addition of Vectors Important Questions Short Answer Type

Question 1.
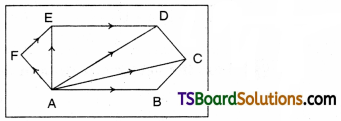
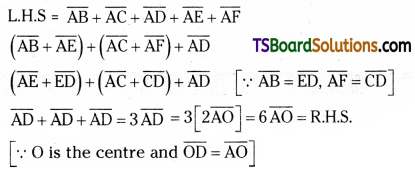
Let ABCDEF be a regular hexagon with centre ’O’. Show that [Mar. 16 (AP), 15 (AP), 03]
\(\overline{\mathrm{AB}}+\overline{\mathrm{AC}}+\overline{\mathrm{AD}}+\overline{\mathrm{AE}}+\overline{\mathrm{AF}}=3 \overline{\mathrm{AD}}=6 \overline{\mathrm{AO}}\)
Answer:
ABCDEF is a regular hexagon with centre ‘O’.

Question 2.
In the two dimensional plane, prove by using vector methods, the equation of the line whose intercepts on the axes are ‘a’ and ‘b’ is \(\frac{x}{a}+\frac{y}{b}\) = 1. [May 05, 01]
Answer:

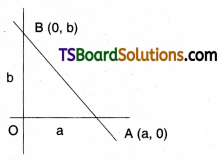
Let A = (a, 0) and B = (0, b)
∴ A = aī, B = bj̄
The equation of the straight line AB is r̄ = (1 – t) ā + tb̄, t ∈ R
r̄ = (1 – t)aī + tbj̄
Let r̄ = xī + yj̄
⇒ xī + yj̄ = (1 – t)aī + tbj̄
Equating the corresponding coefficients of ī, j̄ we have
(1 -1) a = x
\(\frac{x}{a}\) = 1 – t ⇒ \(\frac{x}{a}\) + t = 1 …………… (1)
tb = y ⇒ t = \(\frac{y}{b}\)
From (a) ⇒ \(\frac{x}{a}+\frac{y}{b}\) = 1
∴ The equation of the lines is \(\frac{x}{a}+\frac{y}{b}\) = 1.

Question 3.
Find the point of intersection of the line r̄ = 2ā + b̄ + t(b̄ – c̄) and the plane r̄ = ā + x(b̄ + c̄) + y(ā + 2b̄ – c̄), where ā, b̄ and c̄ are non-coplanar vectors. [May 13]
Answer:
Given r̄ = 2ā + b̄ + t(b̄ – c̄), r̄ = ā + x(b̄ + c̄) + y(ā + 2b̄ – c̄)
Let r̄ be the position vector of the point P the intersection of the line and the plane.
∴ 2ā + b̄ + t(b̄ – c̄) = ā + x(b̄ + c̄) + y(ā + 2b̄ – c̄) (∵ ā, b̄, c̄ are non-coplanar)
Equating the coefficients of ā, b̄, c̄
2 = y + 1 ⇒ y = 1 ⇒ 1 + t = x + 2y ⇒ 1 + t = x + 2 ⇒ t – x = 1 ……………… (1)
– t = x – y ⇒ x + t = y ⇒ x + t = 1 …………….. (2)
Solving (1) and (2) equations t = 1, x = 0.
∴ Point of intersection is r̄ = 2ā + b̄ + b̄ – c̄ = 2ā + 2b̄ – c̄
Hence the position vector of the point of intersection is 2ā + 2b̄ – c̄.

Question 4.
If ā, b̄, c̄ are non coplanar, find the point of intersection of the line passing through the points 2ā + 3b̄ – c̄, 3ā + 4b̄ – 2c̄ with the line joining the points ā – 2b̄ + 3c̄, ā – 6b̄ + 6c̄.
Answer:
Let P = 2ā + 3b̄ – c̄, Q = 3ā + 4b̄ – 2c̄, R = ā – 2b̄ + 3c̄, S = ā – 6b̄ + 6c̄
The equation of the straight line passing through P(2ā + 3b̄ – c̄), Q(3ā + 4b̄ – 2c̄) is
r̄ = (1 – t) (ā + tb̄), t ∈ R
r̄ = (1 – t) (2ā + 3b̄ – c̄) + t(3ā + 4b̄ – 2c̄)
= 2ā + 3b̄ – c̄ – 2tā – 3tab̄ + tc̄ + 3tā + 4tb̄ – 2tc̄
= 2ā + 3b̄ – c̄ + tā + tb̄ – tc̄
r̄ = (2 + t) ā + (3 + t)b̄ + (- 1 – t)c̄ ………………… (1)
The equation of the straight line passing through
R(ā – 2b̄ + 3c̄) and S(ā – 6b̄ + 6c̄) is r̄ = (1 – s)ā + sb̄, s ∈ R
r̄ =(1 – s) (ā – 2b̄ + 3c̄) + s(ā – 6b̄ + 6c̄)
= ā – 2b̄ + 3c̄ – sā + 2sb̄ – 3sc̄ + sā – 6sb̄ + 6sc̄ = ā – 2b̄ + 3c̄ – 4sb̄ + 3sc̄
r̄ = ā + (- 2 – 4s)b̄ + (3 + 3s)c̄ …………….. (2)
From (1) and (2), (2 + t)ā + (3 + t)b̄ + (-1 – t)c̄ = a + (- 2 – 4s)b̄ + (3 + 3s)c̄
Equating the corresponding coefficients of ā, b̄ and c̄ we have

2 + t = 1
t = – 1

3 + t = – 2 – 4s
3 – 1 = – 2 – 4s
2 = – 2 – 4s
4 = – 4s
s = – 1

– 1 – t = 3 + 3s
– 4 = 3s + t
– 4 = 3 (- 1) – 1
– 4 = – 3 – 1
– 4 = – 4

∴ The lines (1) and (2) are intersect each other.
∴ Substituting the value of t = – 1 in (1) (or) s = – 1 in (2)
The point of intersection of the lines is r̄ = (2 – 1)ā + (3 – 1)b̄ + (- 1 + 1)c̄ ⇒ r̄ = ā + 2b̄

DTP. Show that the line joining the pair of points 6a – 4b + 4c, – 4c and the line joining the
pair of points – a – 2b – 3c, a + 2b – 5c intersect at the point -4c when a, b, c are non- coplanar vectors.

Question 5.
Find the vector equation of the plane which passes through the points 2ī + 4j̄ + 2k̄, 2ī + 3j̄ + 5k̄ and parallel to the vector 3ī – 2j̄ + k̄. Also find the point where this plane meets the line joining the points 2ī + j̄̄ + 3k̄ and 4ī – 2j̄+ 3k̄. [Mar. 12]
Answer:
The vector equation of the plane passing through 2ī + 4j̄ + 2k̄, 2ī + 3j̄ + 5k̄ and parallel to the vector 3ī – 2j̄ + k̄ is
r̄ = (1 – t)ā + tb̄ + sc̄; t, s ∈ R
= (1 – t) [2ī + 4j̄ + 2k̄] + t [2ī + 3j̄ + 5k̄] + s(3ī – 2j̄ + k̄)
= 2ī + 4j̄ + 2k̄ – 2tī – 4tj̄ – 2tk̄ + 2tī + 3tj̄ + 5tk̄ + 3sī – 2sj̄ + sk̄
= (2 – 2t + 2t + 3s)ī + (4 – 4t + 3t – 2s)j̄ + (2 – 2t + 5t + s)k̄
r̄ = (2 + 3s)ī + (4 – t – 2s)j̄ + (2 + 3t + s)k̄; s, t ∈ R ……………… (1)
The vector equation of a line passing through the points 2ī + j̄ + 3k̄ and 4ī – 2j̄ +3k̄ is
r̄ = (1 – x)ā + xb̄, x ∈ R
r̄ = (1 – x)(2ī + j̄ + 3k̄) + x(4ī – 2j̄ + 3k̄)
= 2ī + j̄ + 3k̄ – 2xī + xj̄ + 3xk̄ + 4xī – 2xj̄ + 3xk̄
= 2ī + j̄ + 3k̄ – 2xī – 3xj̄
r̄ = (2 + 2x)ī + (1 – 3x)j̄ + 3k̄, x ∈ R …………… (2)
The point of Intersect ion of (1) & (2) Is P. Let the position vector of P Is ÕP = y from (1) & (2)
(2 + 3s)ī + (4 – t – 2s)j̄ + (2 + 3t + s)k̄ = (2 + 2x)ī + (1 – 3x)j̄ + 3k̄
Equation the corresponding coefficients if ī, j̄ and k̄
2 + 3s = 2 + 2x
2x – 3s = 0 ………… (3)
4 – t – 2s = 1 – 3x
t + 2s – 3x = 3 …………… (4)
2 + 3t + s = 3
3t + s = 1 …………… (5)
3 × (4) – (5)
3t + 6s – 9x = 9
3t + s = 1
5s – 9x = 8
9x – 5s = – 8 ……………… (6)
Solve (3) and (6)

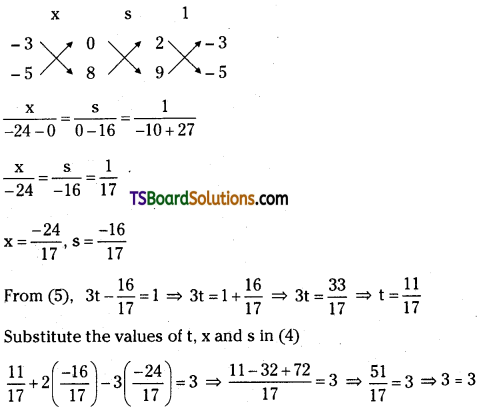
∴ The plane and line Intersect each other.
∴ Substituting the value of s, t in (1) (or) x in (2)
∴ The point of intersection is

Question 6.
Find the vector equation of the plane passing through the points 4ī – 3j̄ – k̄ , 3ī + 7j̄ – 10k̄ and 2ī + 5j̄ – 7k̄ and show that the point ī + 2j̄ – 3k̄ lies in the plane.
Answer:
Vector equation of the plane passing through A(4ī – 3j̄ – k̄); B(3ī + 7j̄ – 10k̄) and C(2ī + 5j̄ – 7k̄) is
r̄ = (1 – s – t) (4ī – 3j̄ – k̄) + s(3ī + 7j̄ – 10k̄) + t(2ī + 5j̄ – 7k̄)
Let D(ī + 2j̄ – 3k̄) lies on the plane, then
(ī + 2j̄ – 3k̄) = (1 – s – t) (4ī – 3j̄ – k̄) + s(3ī + 7j̄ – 10k̄) + t(2ī + 5j̄ – 7k̄)
Since i, j, k are non coplanar, equating co-efficients of ī, j̄, k̄ on both sides
4(1 – s – t) + 3s + 2t = 1 ⇒ 4 – 4s – 4t + 3s + 2t = 1 ⇒ s + 2t = 3 ……………. (1)
– 3(1 – s -1) + 7s + 5t = 2 ⇒ – 3 + 3s + 3t + 7s + 5t = 2 ⇒ 10s + 8t = 5 …………… (2)
Also – (1 – s -1) – 10s – 7t = – 3 ⇒ – 1 + s + t – 10s – 7t = – 3 ⇒ 9s + 6t = 2 …………… (3)
From (1), 3s + 6t = 9
Solving (1) and (3) equations 6s = – 7 ⇒ s = – \(\frac{7}{6}\)

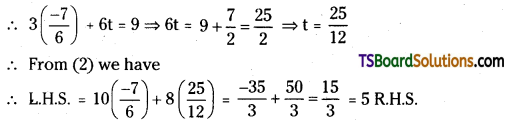
∴ s = \(\frac{-7}{6}\), t = \(\frac{25}{12}\) satisfy (1), (2), (3) and D lies on the plane passing through A, B, C.

Question 7.
Let ā = ī + 2j̄ + 3k̄ and b̄ = 3ī + j̄. Find the unit vector in the direction of a + b.
Answer:
Given ā = ī + 2j̄ + 3k̄, b̄ = 3ī + j̄
Now ā + b̄ = ī + 2j̄ + 3k̄ + 3ī + j̄ = 4ī + 3j̄ + 3k̄
|ā + b̄| = \(\sqrt{4^2+3^2+3^2}\) = \(\sqrt{16+9+9}\) = √34
∴ The unit vector in the direction of
ā + b̄ = \(\frac{\bar{a}+\bar{b}}{|\bar{a}+\bar{b}|}\) = \(\frac{4 \bar{i}+3 \bar{j}+3 \bar{k}}{\sqrt{34}}\) = \(\frac{1}{\sqrt{34}}\) (4ī + 3j̄ + 3k̄)

Question 8.
ā = 2ī + 5j̄ + k̄ and b̄ = 4ī + mj̄ + nk̄ are collinear vectors, then find m and n.
Answer:
Given vectors are ā = 2ī + 5j̄ + k̄, b̄ = 4ī + mj̄ + nk̄
If a₁ī + b₁j̄ + c₁k̄ and a₂ī + b₂j̄ + c₂k̄ vectors are collinear, then \(\frac{a_1}{a_2}=\frac{b_1}{b_2}=\frac{c_1}{c_2}\)
∴ Since ā, b̄ are collinear, then \(\frac{2}{4}=\frac{5}{m}=\frac{1}{n}\)
⇒ \(\frac{1}{2}=\frac{5}{m}=\frac{1}{n}\) ⇒ \(\frac{1}{2}=\frac{5}{m}, \frac{1}{2}=\frac{1}{n}\)
∴ m = 10 and n = 2

Question 9.
Show that the points whose position vectors are ā – 2b̄ + 3c̄, 2ā + 3b̄ – 4c̄, – 7b̄ + 10c̄ are collinear.
Answer:
Let A, B, C be the given points respectively. Then

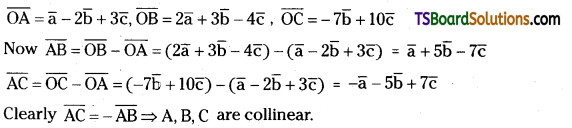
Question 10.
Find the vector equation of the plane passing through the points (0, 0, 0), (0, 5, 0) and (2, 0, 1).
Answer:
Let ā = 0̄; b̄ = 5j̄; c̄ = 2ī + k̄
The vector equation of the plane passing through the points 0̄, 5j̄ and 2ī + k̄ is
r̄ = (1 – s – t)ā + tb̄; t, s ∈ R = (1 – t – s)(0̄) + t(5j̄) + s(2ī + k̄); t, s ∈ R
r̄ = (5t)j̄ + 5(2ī + k̄); t, s ∈ R

Question 11.
Show that the line joining the pair of points 6ā – 4b̄ + 4c̄, – 4c̄ and the line joining the pair of points – ā – 2b̄ – 3c̄, ā + 2b̄ – 5c̄ intersect at the point – 4c̄ when ā, b̄, c̄ are non- coplanar vectors.
Answer:
Let P = 6ā – 4b̄ + 4c̄; Q = – 4c̄; R = – ā – 2b̄ – 3c̄; S = ā + 2b̄ – 5c̄
The equation of the straight line passing through P(6ā – 4b̄ + 4c̄) and Q(- 4c̄) is
r̄ = (1 – t)ā + tb̄, t ∈ R
r̄ = (1 – t) (6ā – 4b̄ + 4c̄) + t(- 4c̄) =6ā – 4b̄ + 4c̄ – 6tā – 4tb̄ + 4tc̄ – 4tc̄
= 6ā – 4b̄ + 4c̄ – 6tā + 4tb̄ – 8tc̄
r̄ = (6 – 6t)ā + (- 4 + 4t)b̄ + (4 – 8t)c̄ ……………….. (1)

The equation of the straight line passing through R(- ā – 2b̄ – 3c̄) and s(ā + 2b̄ – 5c̄) is
r̄ = (1 – s)ā + sb̄, s ∈ R
= (1 – s)(- ā – 2b̄ – 3c̄) + s(ā + 2b̄ – 5c̄) = – ā – 2b̄ – 3c̄ + sā + 2sb̄ + 3sc̄ + sā + 2sb̄ – 5sc̄
= – ā – 2b̄ – 3c̄ + 2sā + 4sb̄ – 2sc̄
⇒ r̄ = (- 1 + 2s)ā + (- 2 + 4s)b̄ + (- 3 – 2s)c̄ …………….. (2)
From (1) & (2)
(6 – 6t)ā + (- 4 + 4t)b̄ + (4 – 8t)c̄
= (- 1 + 2s)ā + (- 2 + 4s)b̄ + (-3 – 2s)c̄
Equating the corresponding coefficients of ā, b̄ & c̄ we have
6 – 6t = – 1 + 2s ⇒ 6t + 2s = 7 ………. (3)
– 4 + 4t = – 2 + 4s ⇒ – 4t + 4s = – 2
2t – 2s = 1 ……… (4)
4 – 8t = – 3 – 2s = 8t – 2s = 7 ……………. (5)
Solve (3) & (4)

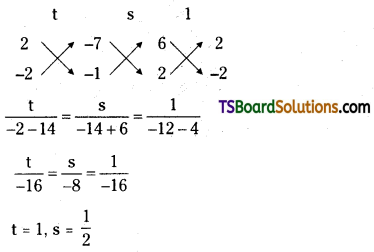
Substitute the values of s, t in equation (5)
8(1) – 2\(\left(\frac{1}{2}\right)\) = 7 ⇒ 8 – 1 = 7 ⇒ 7 = 7
∴ The equations (1) & (2) intersect each other.
Substituting the value of t = 1 in (1)
or s = \(\frac{1}{2}\) in (2)
The point of intersection of the lines is
r̄ = (6 – 6.1)ā + (- 4 + 4.1)b̄ + (4 – 8.1)c̄
r̄ = – 4c̄

Some More Maths 1A Addition of Vectors Important Questions

Question 1.
Find a vector in the direction of vector ā = ī – 2j̄ that has magnitude 7 units.
Answer:
Given ā = ī – 2j̄
|ā| = \(\sqrt{(1)^2+(-2)^2}\) = \(\sqrt{1+4}\) = √5
The unit vector in the direction of the vector a is \(\frac{\Lambda}{\mathbf{a}}\) = \(\frac{\bar{a}}{|\bar{a}|}=\frac{\bar{i}-2 \bar{j}}{\sqrt{5}}\)
∴ The vector having magnitude equal to 7 and in the direction of ā is
\(\frac{\Lambda}{\mathbf{a}}\) = \(7\left(\frac{\overline{\mathrm{i}}-2 \overline{\mathrm{j}}}{\sqrt{5}}\right)=\frac{7}{\sqrt{5}} \overline{\mathrm{i}}-\frac{14}{\sqrt{5}} \overline{\mathrm{j}}\)

Question 2.
Consider the two points P and Q with position vectors \(\overline{\mathrm{OP}}\) = 3ā – 2b̄ and \(\overline{\mathrm{OQ}}\) = ā + b̄. Find the position vector of a point R which divides the line joining P and Q in the ratio 2 : 1 (i) internally and (ii) externally.
Answer:
(i) The position vector of the point R dividing the joining of P and Q internally in the ratio 2: 1 is
\(\overline{\mathrm{OR}}\) = \(\frac{2(\overline{\mathrm{a}}+\overline{\mathrm{b}})+(3 \overline{\mathrm{a}}-2 \overline{\mathrm{b}})}{2+1}=\frac{5 \overline{\mathrm{a}}}{3}\)

(ii) The position vector of the point R dividing the joining of P and Q externally in the ratio 2: 1 is
\(\overline{\mathrm{OR}}\) = \(\frac{2(\overline{\mathrm{a}}+\overline{\mathrm{b}})-(3 \overline{\mathrm{a}}-2 \overline{\mathrm{b}})}{2-1}\) = 4b̄ – ā

Question 3.
Show that the points A(2ī – j̄ + k̄), B(ī – 3j̄ – 5k̄), C(3ī – 4j̄ – 4k̄) are the vertices of a right angled triangle.
Answer:
Given position vectors

Clearly, AB = AC and AB² = BC² + AC²
∴ ∆ABC is right angled.

Question 4.
In ∆ABC, if ‘O’ is the circumcentre and H is the orthocentre, then show that
(i) \(\overline{\mathrm{OA}}+\overline{\mathrm{OB}}+\overline{\mathrm{OC}}=\overline{\mathrm{OH}}\)
(ii) \(\overline{\mathrm{HA}}+\overline{\mathrm{HB}}+\overline{\mathrm{HC}}=2 \overline{\mathrm{HO}}\)
Answer:
Take ‘O’ as origin the position vectors of vertices A, B and C with respect to the origin ‘O’ are
\(\overline{\mathrm{OA}}=\overline{\mathrm{a}}\), \(\overline{\mathrm{OB}}=\overline{\mathrm{b}}\) and \(\overline{\mathrm{OC}}=\overline{\mathrm{c}}\)
Let D be the midpoint of BC then the position vector of D is

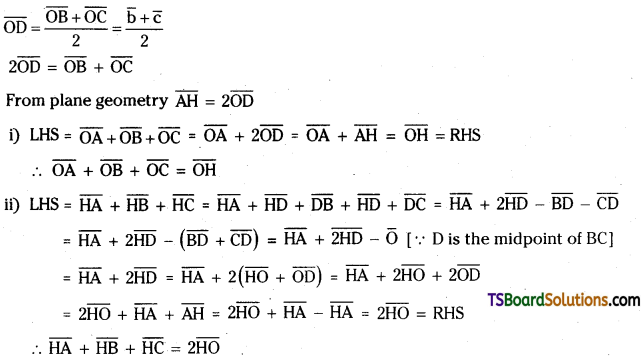
Question 5.
If the points whose position vectors are 3ī – 2j̄ – k̄, 2ī + 3j̄ – 4k̄, – ī + j̄ + 2k̄ and 4ī + 5j̄ + λk̄ are coplanar, then show that λ = \(\frac{-146}{17}\). [May 15 (TS)]
Answer:
Let A, B, C, D be the given points respectively.

Since A, B, C and D are coplanar, then [\(\overline{\mathrm{AB}}\) \(\overline{\mathrm{AC}}\) \(\overline{\mathrm{AD}}\)] = 0
⇒ \(\left|\begin{array}{ccc}
-1 & 5 & -3 \\
-4 & 3 & 3 \\
1 & 7 & \lambda+1
\end{array}\right|\) = 0 ⇒ – 1(3λ + 3 – 21) – 5(- 4λ – 4 – 3) – 3(- 28 – 3) = 0
⇒ – 1 (3λ – 18) – 5(- 4λ – 7) – 3(- 31) = 0
⇒ – 3λ + 18 + 20λ + 35 + 93 = 0 -146
⇒ 17λ + 146 = 0
⇒ λ = \(\frac{-146}{17}\)

Question 6.
ABCDE is a pentagon. If the sum of the vectors \(\overline{\mathbf{A B}}, \overline{\mathbf{A E}}, \overline{\mathbf{B C}}, \overline{\mathbf{D C}}, \overline{\mathbf{E D}}\) and \(\) is λ \(\overline{\mathbf{A C}}\), then find the value of λ.
Answer:
ABCDE is a pentagon. Given that

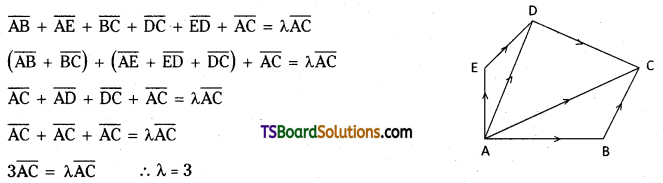
Question 7.
Is the triangle formed by the vectors 3ī + 5j̄ + 2k̄, 2ī – 3j̄ – 5k̄ and – 5ī – 2j̄ + 3k̄ equilateral ?
Answer:
Let ABC be the triangle with

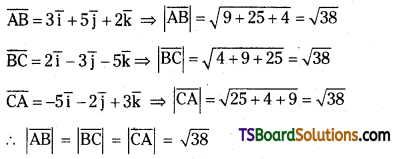
∴ The given vectors formed on equilateral triangle.

Question 8.
ā, b̄, c̄ are non-coplanar vectors. Prove that the four points – ā + 4b̄ – 3c̄, 3ā + 2b̄ – 5c̄, – 3ā + 8b̄ – 5c̄, – 3ā + 2b̄ + c̄ are coplanar.
Answer:

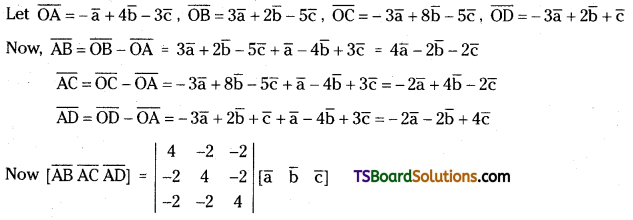
= [4 (16 – 4) + 2 (- 8 – 4) – 2 (4 + 8)] [ā b̄ c̄]
= [4(12) + 2 (-12) – 2 (12)] [ā b̄ c̄]
= [48 – 24 – 24] [ā b̄ c̄] = 0 [ā b̄ c̄] = 0
∴ The given points are coplanar.

Question 9.
a, b, c are non-coplanar vectors. Prove that the four points 6ā + 2b̄ – c̄, 2ā – b̄ + 3c̄, – ā + 2b̄ – 4c̄, – 12ā – b̄ – 3c̄ are coplanar.
Answer:
Given that a, b, c are non-coplanar ⇒ [ā b̄ c̄] ≠ 0

Question 10.
If ī, j̄, k̄ are unit vectors along the positive directions of the coordinate axes, then show that the four points 4ī + 5j̄ + k̄, – j̄ – k̄, 3ī + 9j̄ + 4k̄ and – 4ī + 4j̄ + 4k̄ are coplanar. [Mar. 14]
Answer:

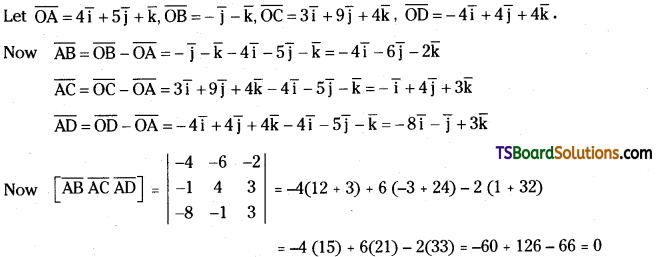
∴ The given vectors are coplanar.

Question 11.
Show that the points whose position vectors are 2ā + 5b̄ – 4c̄ , ā + 4b̄ – 3c̄, 4ā + 7b̄ – 6c̄ are collinear when a, b, c are non-coplanar vectors.
Answer:
Let A, B, C be the given points respectively.