TS Inter Second Year Maths 2B Parabola Important Questions Long Answer Type

Students must practice these Maths 2B Important Questions TS Inter Second Year Maths 2B Parabola Important Questions Long Answer Type to help strengthen their preparations for exams.

TS Inter Second Year Maths 2B Parabola Important Questions Long Answer Type

Question 1.
Show that the equation of a parabola in the standard form is y² = 4ax. [(TS) Mar. ’20, ’18, ’17, ’16; May ’18; (AP) May ’19, ’15; Mar. ’17, ’15]
Solution:

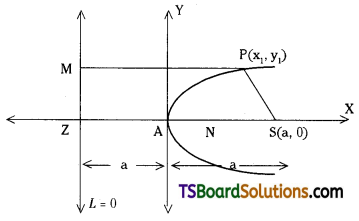
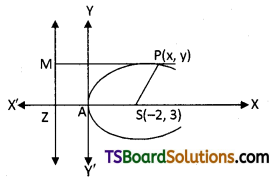
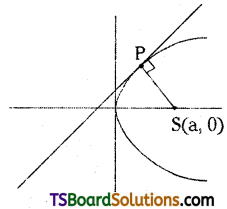
Let S be the focus and l = 0 be the directrix of the parabola.
Let ‘P’ be a point on the parabola.
Let M, Z be the projection (foot of the perpendiculars) of P, S on the directrix L = 0 respectively.
Let ‘N’ be the projection of P on ‘SZ’.
Let ‘A’ be the midpoint of SZ.
Since SA = AZ
‘A’ lies on the parabola let AS = a.
Take AS, the principal axis of the parabola as X-axis and AY ⊥r to SZ as Y-axis.
Then S = (a, 0) and the parabola is in the standard form.
Let P(x₁, y₁)
Now PM = NZ = AN + AZ = x₁ + a
‘P’ lies on the parabola then
\(\frac{\mathrm{SP}}{\mathrm{PM}}\) = 1
SP = PM
\(\sqrt{\left(x_1-a\right)^2+\left(y_1-0\right)^2}=x_1+a\)
Squaring on both sides,
(x₁ – a)² + (y₁ – 0)² = (x₁ + a)²
⇒ \(\mathrm{y}_1^2\) = (x₁ + a)² – (x₁ – a)²
⇒ \(\mathrm{y}_1^2\) = 4ax₁
The locus of ‘P’ is y² = 4ax
∴ The equation to the parabola is y² = 4ax

Question 2.
Find the coordinates of the vertex and focus and the equation of the directrix and axes of the parabola y² – x + 4y + 5 = 0. (Mar. ’05)
Solution:
Given the equation of the parabola is
y² – x + 4y + 5 = 0
⇒ y² + 4y = x – 5
⇒ (y)² + 2 . 2 . y + (2)² – (2)² = x – 5
⇒ (y + 2)² – 4 = x – 5
⇒ (y + 2)² = x – 1
⇒ (y + 2)² = 1(x – 1)
Comparing with (y – k)² = 4a(x – h), we get
h = 1, k = -2, a = \(\frac{1}{4}\)
(i) Vertex = (h, k) = (1, -2)
(ii) Focus = (h + a, k) = (1 + \(\frac{1}{4}\), -2) = (\(\frac{5}{4}\), -2)
(iii) Equation of the directrix is x = h – a
⇒ x = 1 – \(\frac{1}{4}\)
⇒ x = \(\frac{3}{4}\)
⇒ 4x – 3 = 0
(iv) Equation of the axis is y = k
⇒ y = -2
⇒ y + 2 = 0

Question 3.
Find the vertex and focus of 4y² + 12x – 20y + 67 = 0.
Solution:
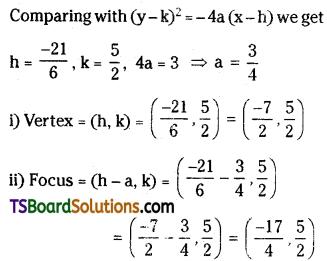
Given equation of the parabola is 4y² + 12x – 20y + 67 = 0
4y² – 20y = -12x – 67
4(y² – 5y) = -12x – 67

Question 4.
Find the coordinates of the vertex and focus, the equation of the directrix, and the axis of the parabola y² + 4x + 4y – 3 = 0.
Solution:
Given the equation of the parabola is
y² + 4x + 4y – 3 = 0
⇒ y² + 4y = -4x + 3
⇒ (y)² + 2 . y(2) + (2)² – (2)² = -4x + 3
⇒ (y + 2)² – 4 = -4x + 3
⇒ (y + 2)² = -4x + 7
⇒ (y + 2)² = -4(x – \(\frac{7}{4}\))
[y-(-2)]² = -4(x – \(\frac{7}{4}\))
Comparing with (y – k)² = -4a(x – h), we get
h = \(\frac{7}{4}\), k = -2, 4a = 4 ⇒ a = 1
(i) Vertex = (h, k) = (\(\frac{7}{4}\), -2)
(ii) Focus = (h – a, k) = (\(\frac{7}{4}\) – 1, -2) = (\(\frac{3}{4}\), -2)
(iii) Equation of the directrix is x = h + a
⇒ x = \(\frac{7}{4}\) + 1
⇒ x = \(\frac{11}{4}\)
⇒ x = 4x – 11 = 0
(iv) Equation of the axis is y = k
⇒ y = -2
⇒ y + 2 = 0

Question 5.
Find the equations of the axis and directrix of the parabola 4x² + 12x – 20y + 67 = 0.
Solution:
Given equation of the parabola is 4x² + 12x – 20y + 67 = 0
⇒ 4x² + 12x = 20y – 67
⇒ 4(x² + 3x) = 20y – 67

Comparing with (x – h)² = 4a(y – k) we get
h = \(-\frac{3}{2}\), k = \(\frac{29}{10}\),
4a = 5 ⇒ a = \(\frac{5}{4}\)
(i) Equation of the axis is x = h
⇒ x = \(-\frac{3}{2}\)
⇒ 2x + 3 = 0
(ii) Equation of the directrix is y = k – a
⇒ y = \(\frac{29}{10}-\frac{5}{4}\)
⇒ y = \(\frac{33}{20}\)
⇒ 20y – 33 = 0

Question 6.
Find the coordinates of the vertex and focus and the equations of the directrix and axes of the parabola 3x² – 9x + 5y – 2 = 0.
Solution:
Given equation of the parabola
3x² – 9x + 5y – 2 = 0
⇒ 3x² – 9x = -5y + 2
⇒ 3(x² – 3x) = -5y + 2

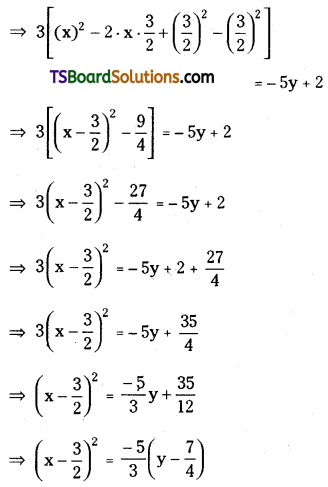
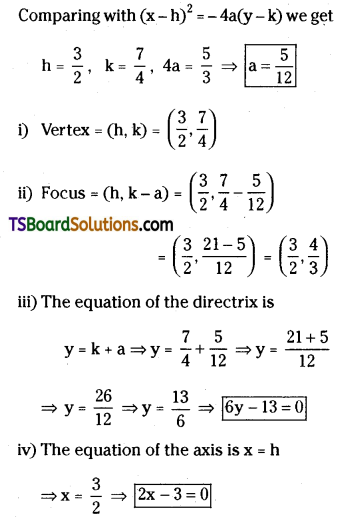
Question 7.
Find the equation of the parabola whose axis is parallel to the X-axis and which passes through die points (-2, 1), (1, 2), and (-1, 3). [(AP) May ’18, ’16, (TS) ’17]
Solution:
Let, the given points are A(-2, 1), B(1, 2), C(-1, 3)
The equation of the parabola whose axis is parallel to the X-axis is
x = ly² + my + n ……….(1)
Since, eq. (1) passes through point A(-2, 1) then
(-2) = l(1)² + m(1) + n
⇒ -2 = l + m + n
⇒ l + m + n = -2
Since, (1) passes through point B(1, 2) then
(1)² = l(2)² + m(2) + n
⇒ 1 = 4l + 2m + n
⇒ 4l + 2m + n = 1 …….(3)
Since, (1) passes through point C(-1, 3), then
-1 = l(3)² + m(3) + n
9l + 3m + n = -1 ………(4)
From (2) and (3)

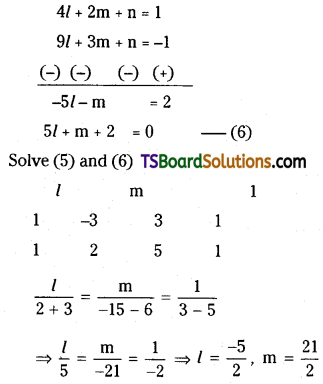
Substitute the values of l, m in (2)
\(\frac{-5}{2}+\frac{21}{2}\) + n = -2
⇒ -5 + 21 + 2n = -4
⇒ 16 + 2n = -4
⇒ 2n = -20
⇒ n = -10
Substitute the values of l, m, n in (1),
The required equation of the parabola is
\(\mathbf{x}=\frac{-5}{2} \mathbf{y}^2+\frac{21}{2} \mathbf{y}-10\)
⇒ -5y² + 21y – 20 = 2x
⇒ 5y² + 2x – 21y + 20 = 0

Question 8.
Find the equation of the parabola passing through the points (-1, 2), (1, -1), and (2, 1) and having its axis parallel to the X-axis.
Solution:
Let, the given points are A(-1, 2), B(1, -1), C(2, 1)
The equation of the parabola whose axis is parallel to the X-axis is
x = ly² + my + n …….(1)
Since, (1) passes through point A(-1, 2) then
(-1) = l(2)² + m(2) + n
⇒ -1 = 4l + 2m + n
⇒ 4l + 2m + n = -1 ……..(2)
Since, (1) passes through point B(1, -1) then
(1) = l(-1)² + m(-1) + n
⇒ l – m + n = 1 ……..(3)
Since (1) passes through point C(2, 1) then
2 = l(1)² + m(1) + n
⇒ l + m + n = 2 ……(4)
From (2) and (3)

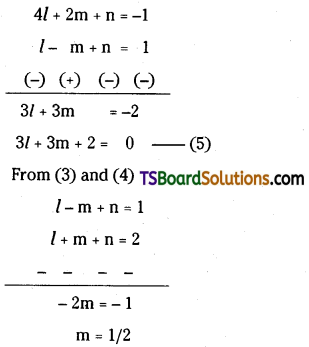
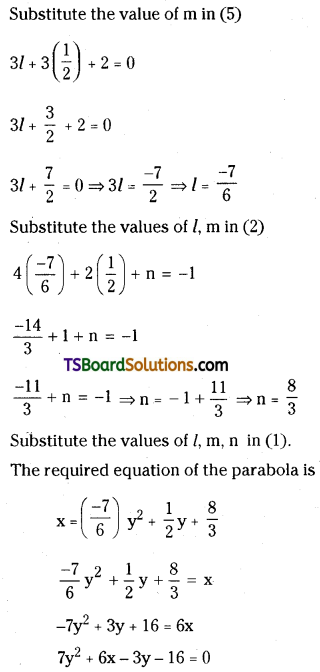
Question 9.
Find the equation of the parabola whose X-axis is parallel to the Y-axis and which passes through the point (4, 5), (-2, 11), (-4, 21). (May ’12)
Solution:
The equation of the parabola whose axis is parallel to the Y-axis then
y = lx² + mx + n ……..(1)
Since eq. (1) passes through the point (4, 5) then
5 = l(4)² + m(4) + n
⇒ 16l + 4m + n = 5 ………(2)
Since eq. (1) passes through the point (-2, 11) then
11 = l(-2)² + m(-2) + n
⇒ 4l – 2m + n = 11 ………(3)
Since eq. (1) passes through the point (-4, 21) then
21 = l(-4)² + m(-4) + n
⇒ 16l + 4m + n = 21 ……….(4)

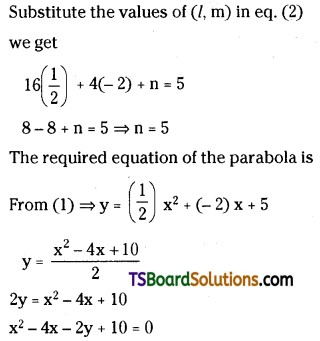
Question 10.
Find the equation of the parabola whose focus is (-2, 3) and whose directrix is the line 2x + 3y – 4 = 0. Also, find the length of the latus rectum and the equation of the axis of the parabola.
Solution:
2x + 3y – 4 = 0
Given that, focus, S = (-2, 3)

The equation of the directrix is 2x + 3y – 4 = 0
Let, P(x, y) be a point on the parabola.
Now, SP = \(\sqrt{(\mathrm{x}+2)^2+(\mathrm{y}-3)^2}\)
PM = the ⊥r distance from P(x, y) to the directrix 2x + 3y – 4 = 0

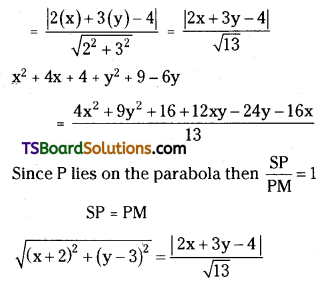
Squaring on both sides
(x + 2)² + (y – 3)² = \(\frac{(2 x+3 y-4)^2}{13}\)
13x² + 52x + 52 + 13y² + 117 – 78y = 4x² + 9y² + 12xy – 24y – 16x + 16
9x² – 12xy + 4y² + 68x – 54y + 153 = 0
∴ The equation to the parabola is 9x² – 12xy + 4y² + 68x – 54y + 153 = 0
Now, the length of the latus rectum = 4a
= 2(2a)
= 2(the ⊥r distance from focus S(-2, 3) to the directrix 2x + 3y – 4 = 0)

The equation of the axis of the parabola is b(x – x₁) – a(y – y₁) = 0
⇒ 3(x + 2) – 2(y – 3) = 0
⇒ 3x + 6 + 2y – 6 = 0
⇒ 3x + 2y = 0

Question 11.
Find the locus of the point of trisection of the double ordinate of a parabola y² = 4ax (a > 0).
Solution:
The given equation of a parabola is y² = 4ax (a > 0)

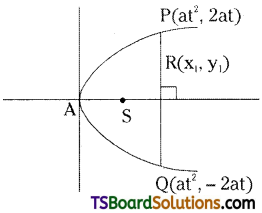
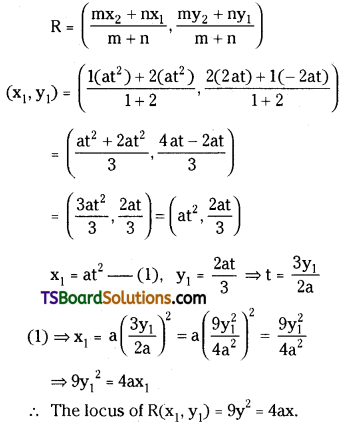
Let, the ends double-ordinate the parabola
y² = 4ax are P(at², 2at), Q(at², -2at)
Let R(x₁, y₁) be any point on the locus trisection ratio = 1 : 2
R(x₁, y₁) is the trisection point, then

Question 12.
Show that the equation of a common tangent to the circle x² + y² = 2a² and the parabola y² = 8ax are y = ±(x + 2a). [(TS) May ’19, ’16 (AP) ’17]
Solution:
Given the equation of the parabola is y² = 8ax
The equation of the tangent to the parabola
y² = 4ax is y = mx + \(\frac{a}{m}\)
The equation of the tangent to the parabola
y² = 8ax is y = mx + \(\frac{2a}{m}\) (∵ a = 2a)
Given the equation of the circle is x² + y² = 2a²
Centre C = (0, 0)
Radius r = √2a
Since eq. (1) is a tangent to the circle x² + y² = 2a² then r = d

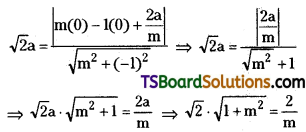
Squaring on both sides we get
2(1 + m²) = \(\frac{4}{\mathrm{~m}^2}\)
⇒ m²(1 + m²) = 2
⇒ m² + m⁴ – 2 = 0
⇒ m⁴ + m² – 2 = 0
⇒ m⁴ + 2m² – m² – 2 = 0
⇒ m²(m² + 2) – 1(m² + 2) = 0
⇒ (m² + 2) (m² – 1) = 0
⇒ m² + 2 = 0 or m² – 1 = 0
⇒ m² = -2 or m² = 1
⇒ m = ±√-2 ∉ R or m = ± 1
Substitute the value of ‘m’ in eq. (1)
∴ The equation of the common tangents is
y = \(\pm x+\frac{2 a}{\pm 1}\)
⇒ y = ±(x + 2a)

Question 13.
Show that the common tangent to the parabola y² = 4ax and x² = 4by is \(x a^{1 / 3}+y b^{1 / 3}+a^{2 / 3} \cdot b^{2 / 3}=0\). [(AP) Mar. ’16]
Solution:
Given equations of the parabola are
y² = 4ax …….(1) and x² = 4by …..(2)

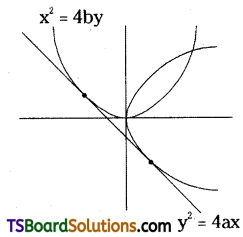
Equation of any tangent to (1) is of the form
y = mx + \(\frac{a}{m}\) ……..(3)
If line (3) is a tangent to (2) also.
The points of intersection of (2) and (3) coincide.
Substituting the value of y from (3) in (2), we get
⇒ x² = \(4 b\left(m x+\frac{a}{m}\right)\)
⇒ x² = 4bmx + \(\frac{4ab}{m}\)
⇒ mx² = 4bm²x + 4ab
⇒ mx² – 4bm²x – 4ab = 0
This equation has equal roots, then it’s discriminant = 0
b² – 4ac = 0
⇒ (-4bm²)² – 4(m) (-4ab) = 0
⇒ 16b²m⁴ + 16abm = 0
⇒ b²m⁴ + abm = 0
⇒ bm⁴ + am = 0
⇒ m(bm³ + a) = 0
⇒ m = 0 (or) bm³ + a = 0

Question 14.
The normal at a point ‘t₁’ on y² = 4ax meets the parabola again in the point ‘t₂’ then prove that t₁t₂ + \(t_1^2\) + 2 = 0. (May ’13)
Solution:

Given the equation of the parabola is y² = 4ax
The equation of the normal at P(\(\mathrm{at}_1{ }^2\), 2at₁) is
y + xt₁ = 2at₁ + \(\mathrm{at}_1{ }^3\) …….(1)
Since eq. (1) meets the parabola again in the Q(\(\mathrm{at}_2{ }^2\), 2at₂) then

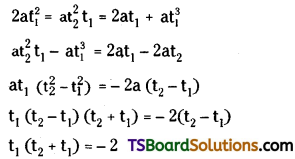
Question 15.
If lx + my + n = 0 is a normal to the parabola y² = 4ax, then show that al³ + 2alm² + nm² = 0.
Solution:

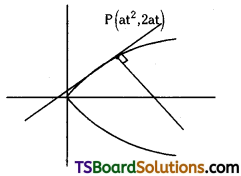
Given the equation of the parabola is y² = 4ax
Given the equation of the normal is
lx + my + n = 0 ……..(1)
Now, the equation of the normal at P(at², 2at) is
y + xt = 2at + at³ ……..(2)
Now, (1) and (2) represent the same line then

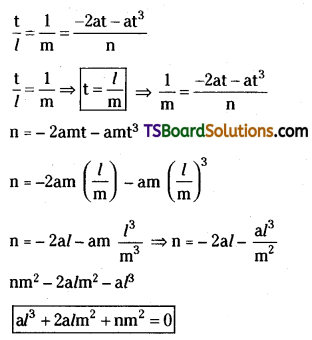
Which is the required condition.

Question 16.
If a normal chord at a point t on the parabola y² = 4ax subtends a right angle at the vertex then show that t = ±√2. (May ’14)
Solution:
Given the equation of the parabola is y² = 4ax

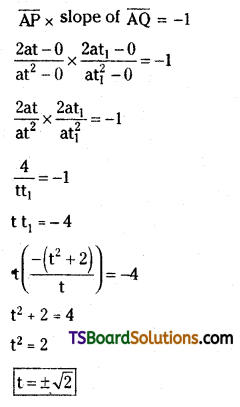
Question 17.
Show that the locus of the point of intersection of perpendicular tangents to the parabola y² = 4ax is the directrix x + a = 0.
Solution:
Given, the equation of the parabola is y² = 4ax.
Let P(x₁, y₁) be the point of intersection of perpendicular tangents of y² = 4ax.
The equation to the pair of tangents drawn from P(x₁, y₁) is \(\mathrm{S}_1{ }^2\) = S.S₁₁
⇒ [yy₁ – 2a(x + x₁)]² = (y² – 4ax) (\(\mathbf{y}_1{ }^2\) – 4ax₁)
Since the tangents are at right angles, then
coefficient of x² + coefficient of y² = 0
4a² + \(\mathbf{y}_1{ }^2\) – (\(\mathbf{y}_1{ }^2\) – 4ax₁) = 0
⇒ 4a² + \(\mathbf{y}_1{ }^2\) – \(\mathbf{y}_1{ }^2\) + 4ax₁ = 0
⇒ 4a² + 4ax₁ = 0
⇒ a + x₁ = 0
⇒ x₁ + a = 0
∴ The equation to the locus of P(x₁, y₁) is x + a = 0.

Question 18.
Show that the feet of the perpendicular from focus to the tangent of the parabola y² = 4ax lie on the tangent at the vertex.
Solution:
Given equation of the parabola is y² = 4ax.

Equation of a tangent to the parabola y² = 4ax is
y = mx + \(\frac{a}{m}\)
⇒ y = \(\frac{m^2 x+a}{m}\)
⇒ m²x – my + a = 0 ……..(1)
Equation of a line passing through the focus S(a, 0) and perpendicular to the line (1) is
y – y₁ = \(\frac{-1}{m}\)(x – x₁)
⇒ y – 0 = \(\frac{-1}{m}\)(x – a)
⇒ y = \(\frac{-1}{m}\)(x – a)
⇒ my = -x + a
⇒ x + my – a = 0 …….(2)
Solve (1) and (2)
(1) + (2) ⇒ m²x – my + a + x + my – a = 0
⇒ x(m² + 1) = 0
⇒ x = 0 (∵ m² ≠ 1)
∴ The point of intersections of lines (1) and (2) lies on x = 0.
Which is the tangent at the vertex.

Question 19.
From an external point, P tangents are drawn to the parabola y² = 4ax and these tangents make angles θ₁, θ₂ with its axis, such that cot θ₁ + cot θ₂ is a constant ‘d’. Then show that all such P lie on a horizontal line. [Mar. ’19 (TS)]
Solution:
Given the equation of the parabola is y² = 4ax

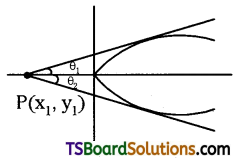
Let P(x₁, y₁) be any point on the required locus.
∴ The equation of any tangent to the parabola y² = 4ax is y = mx + \(\frac{a}{m}\)
If this line passes through P then
y₁ = mx₁ + \(\frac{a}{m}\)
⇒ y₁ = \(\frac{m^2 x_1+a}{m}\)
⇒ my₁ = m²x₁ + a
⇒ x₁m² – y₁m + a = 0 ………(1)
Which is a quadratic equation in m.
If m₁, m₂ are the slopes of the tangents drawn from P to the parabola then m₁, m₂ are the roots of (1)
Sum of the slopes = \(\frac{-b}{a}\)
m₁ + m₂ = \(\frac{-\left(-y_1\right)}{x_1}=\frac{y_1}{x_1}\)
tan θ₁ + tan θ₂ = \(\frac{\mathrm{y}_1}{\mathrm{x}_1}\)
product of the slopes = \(\frac{c}{a}\)
m₁m₂ = \(\frac{\mathrm{a}}{\mathrm{x}_1}\)
tan θ₁ tan θ₂ = \(\frac{\mathrm{a}}{\mathrm{x}_1}\)
(∵ The tangents made angles θ₁, θ₂ with its axis (X -axis) then their slopes m₁ = tan θ₁ and m₂ = tan θ₂)
Given that cot θ₁ + cot θ₂ = d

∴ P lies on a horizontal line y = ad.

Question 20.
From an external point, P tangents are drawn to the parabola y² = 4ax and these tangents make angles θ₁, θ₂ with its axis such that tan θ₁ + tan θ₂ is a constant, b. Then show that P lies on the line y = bx. [(AP) Mar. ’20]
Solution:
Given the equation of the parabola is y² = 4ax

Let, P(x₁, y₁) be any point on the required locus.
∴ The equation of any tangent to the parabola y² = 4ax is y = mx + \(\frac{a}{m}\)
If this line passes through P then
y₁ = mx₁ + \(\frac{a}{m}\)
⇒ y₁ = \(\frac{m^2 x_1+a}{m}\)
⇒ my₁ = m²x₁ + a
⇒ x₁m² – y₁m + a = 0 ……(1)
which is a quadratic equation in m.
If m₁, m₂ are the slopes of the tangents drawn from P to the parabola then m₁, m₂ are the roots of (1).
Sum of the slopes = \(\frac{-b}{a}\)
m₁ + m₂ = \(\frac{-\left(-y_1\right)}{x_1}=\frac{y_1}{x_1}\)
tan θ₁ + tan θ₂ = \(\frac{y_1}{x_1}\)
[∵ The tangents made angles θ₁, θ₂ with its axis (X-axis) then their slopes m₁ = tan θ₁ and m₂ = tan θ₂]
Given that, tan θ₁ + tan θ₂ = b
⇒ \(\frac{y_1}{x_1}\) = b
⇒ y₁ = bx₁
∴ P(x₁, y₁) lies on the line y = bx.

Question 21.
Prove that the two parabolas y² = 4ax and x² = 4by intersect (other than the origin) at an angle of \(\tan ^{-1}\left[\frac{3 a^{\frac{1}{3}} b^{\frac{1}{3}}}{2\left(a^{\frac{2}{3}}+b^{\frac{2}{3}}\right)}\right]\). (Mar. ’14)
Solution:
Without loss of generality, we can assume that a > 0 and b > 0

Let, P(x, y) be the point of intersection of the parabolas other than the origin.
Given equations of the parabolas are y² = 4ax, x² = b4y
⇒ y² = 4ax
Squaring on both sides
y⁴ = 16a²x²
⇒ y⁴ = 16a²(4by)
⇒ y⁴ = 64a²by
⇒ y⁴ – 64a²by = 0
⇒ y(y³ – 64a²b) = 0
⇒ y = 0 or y³ – 64a²b = 0
⇒ y³ = 64a²b
⇒ y = \(4 \mathrm{a}^{2 / 3} \mathrm{~b}^{1 / 3}\)
From y² = 4ax, we get

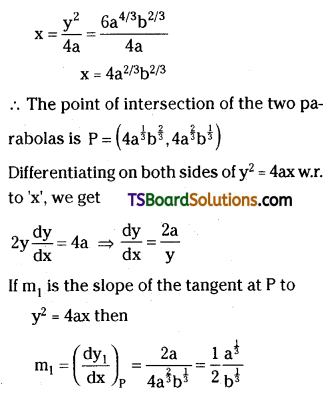
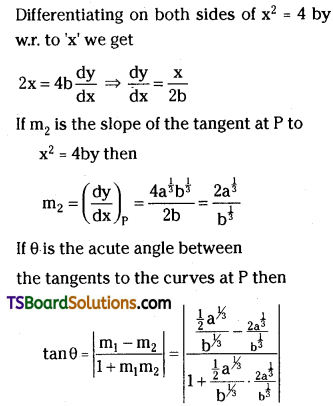
Question 22.
Show that the straight line 7x + 6y = 13 is a tangent to the parabola y² – 7x – 8y + 14 = 0 and find the point of contact.
Solution:
Given the equation of the parabola is
y² – 7x – 8y + 14 = 0 ………(1)
Given the equation of the straight line is
7x + 6y = 13
⇒ 7x = 13 – 6y
⇒ x = \(\frac{13-6 y}{7}\) ………(2)
From (1) and (2) by eliminating x we get the ordinates of the points of intersection of the line and parabola.
y² – 7(\(\frac{13-6 y}{7}\)) – 8y + 14 = 0
⇒ y² – 13 + 6y – 8y + 14 = 0
⇒ y² – 2y + 1 = 0
⇒ (y – 1)² = 0
⇒ y = 1, 1
∴ The given line is tangent to the given parabola substitute the value of y = 1 in (2)
x = \(\frac{13-6}{7}=\frac{7}{7}=1\)
∴ Point of contact = (1, 1)

Question 23.
Show that the common tangents to the circle 2x² + 2y² = a² and the parabola y² = 4ax intersect at the focus of the parabola y² = -4ax.
Solution:
Given the equation of the parabola is y² = 4ax
The equation of the tangent to the parabola y² = 4ax is
y = mx + \(\frac{a}{m}\) ……..(1)
Given the equation of the circle is
2x² + 2y² = a²
⇒ x² + y² = \(\frac{\mathrm{a}^2}{2}\)
∴ Centre, C = (0, 0)
Radius = \(\frac{a}{\sqrt{2}}\)
Since (1) is a tangent to the circle 2x² + 2y² = a² then r = d

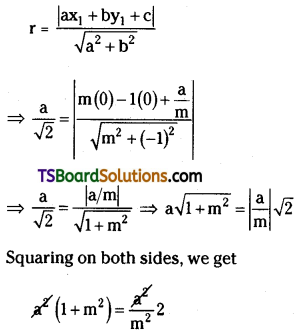
⇒ m² + m⁴ = 2
⇒ m⁴ + m² – 2 = 0
⇒ m⁴ + 2m² – m² – 2 = 0
⇒ m²(m² + 2) – 1(m² + ²) = 0
⇒ (m² + 2)(m² – 1) = 0
⇒ m² + 2 = 0 or m² – 1 = 0
⇒ m² = -2 or m² = 1
⇒ m = ±√-2 ∉ R or m = ±1
Substitute the values of m in (1)
∴ The equations of the common tangents are
y = \(\pm 1 \cdot x+\frac{a}{\pm 1}\)
⇒ y = ±x ± a
⇒ y = ±(x ± a) …….(2)
The focus of the parabola y² = -4ax is S = (-a, 0)
Now, (2) intersects at the focus of the parabola y² = -4ax then
(2) passes through focus, S(-a, 0)
0 = ±(-a + a)
∴ 0 = 0
∴ The common tangents to the circle 2x² + 2y² = a² and the parabola y² = 4ax intersect at the focus of the parabola y² = -4ax.

Question 24.
Show that the condition that the line y = mx + c may be a tangent to the parabola y² = 4ax is c = \(\frac{a}{m}\). (Mar. ’99, ’94; May ’98)
Solution:
Suppose y = mx + c ………(1)
is a tangent to the parabola y² = 4ax
Let P(x₁, y₁) be the point of contact.
The equation of the tangent at ‘P’ is S₁ = 0
⇒ yy₁ – 2a(x + x₁) = 0
⇒ yy₁ – 2ax – 2ax₁ = 0
⇒ 2ax – yy₁ + 2ax₁ = 0 ………(2)
Now, (1) & (2) represent the same line

Since ‘P’ lies on the line

Question 25.
Find the condition for the line y = mx + c to be a tangent to the parabola x² = 4ay. (Mar. ’12; May ’03)
Solution:
Given the equation of the parabola is x² = 4ay
Let the line y = mx + c ………(1)
be a tangent to the parabola x² = 4ay.

The equation of the tangent at P(x₁, y₁) is S₁ = 0
xx₁ – 2a(y + y₁) = 0
⇒ xx₁ – 2ay – 2ay₁ = 0 ……….(2)
Now equations (1) & (2) represent the same line then

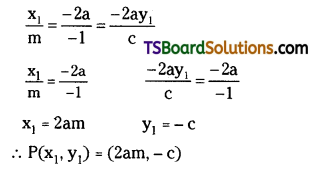
Since P(x₁, y₁) lies on the line y = mx + c then y₁ = mx₁ + c
⇒ -c = m(2am) + c
⇒ 2am² + 2c = 0
⇒ am² + c = 0
Which is the required condition.

Question 26.
Prove that the tangents at the extremities of a focal chord of a parabola intersect at right angles on the directrix.
Solution:
Let the equation of the parabola is y² = 4ax.
Let P(\(\mathrm{at}_1^2\), 2at₁), Q(\(\mathrm{at}_2^2\), 2at₂) are two points on the parabola.


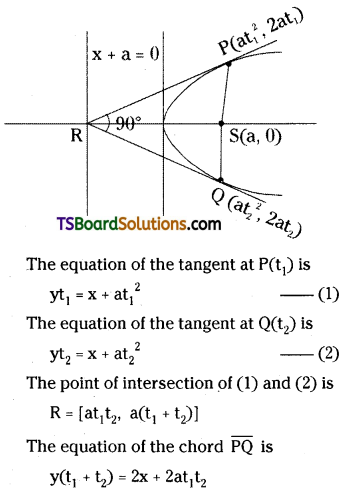
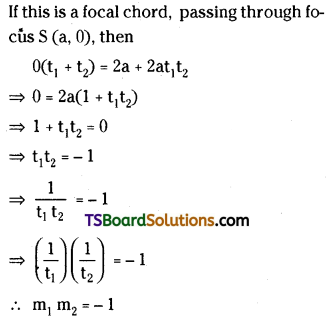
∴ The tangents are drawn at P, Q are perpendicular
The coordinates of R = [-a, a(t₁ + t₂)]
The equation of the directrix of a parabola y = 4ax is x + a = 0
Now, substitute the point R in the directrix x + a = 0
⇒ -a + a = 0
⇒ 0 = 0
∴ The tangents at the extremities of a focal chord of a parabola intersect at right angles on the directrix.

Question 27.
Find the equation of the parabola whose focus is S(3, 5) and the vertex is A(1, 3).
Solution:

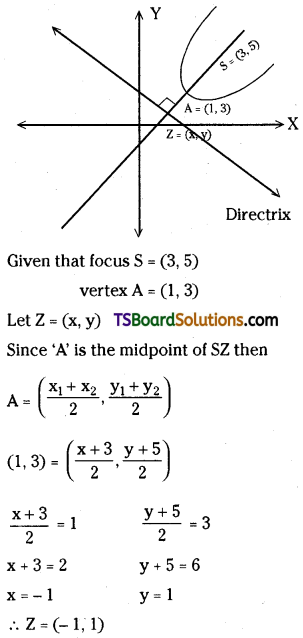
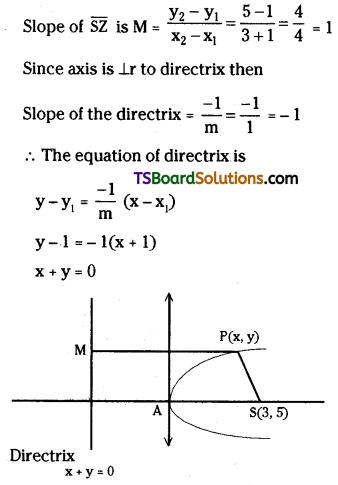
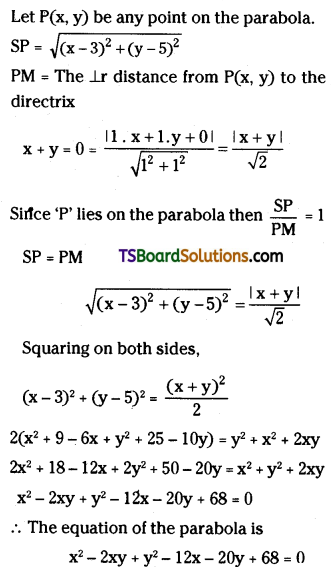
Question 28.
Find the equations of the tangents to the parabola y² = 16x, which are parallel and perpendicular respectively to the line 2x – y + 5 = 0. Find the coordinates of their points of contact also.
Solution:
Given parabola is y² = 16x
Comparing with y² = 4ax
we get 4a = 16 ⇒ a = 4
Given line is 2x – y + 5 = 0
⇒ y = 2x + 5
Comparing with y = mx + c we get
m = 2, c = 5
(i) Equation of the tangent with slope ‘m’ is

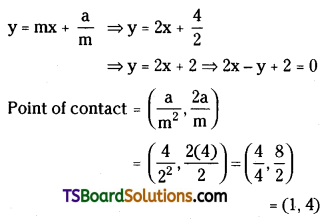
(ii) Slope of the perpendicular given line is m = \(\frac{-1}{2}\)
equation of tangent with slope ‘m’ is

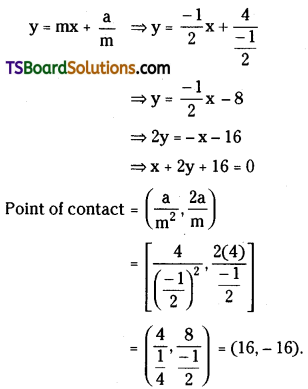
Question 29.
If L and L’ are the ends of the latus rectum of the parabola x² = 6y. Find the equations of OL and OL’ where ‘O’ is the origin. Also, find the angle between them.
Solution:

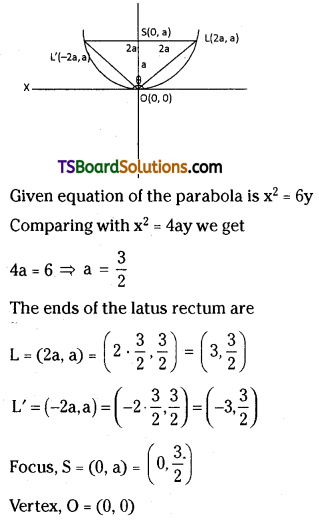
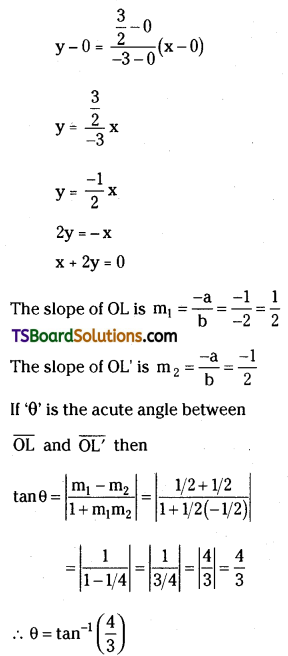
Question 30.
Two parabolas have the same vertex and equal length of latus rectum such that their axes are at right angles. Prove that the common tangent touches each at the end of the latus rectum.
Solution:
Equations of the parabolas can be taken as y² = 4ax and x² = 4ay

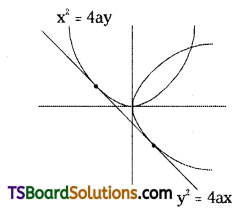
Equation of the tangent at P(2at, at²) to the parabola x² = 4ay is S₁ = 0
⇒ xx₁ – 2a(y + y₁) = 0
⇒ x(2at) – 2a(y + at²) = 0
⇒ xt – y – at² = 0
⇒ tx – y – at² = 0 ………(1)
⇒ y = tx – at²
This is a tangent to y² = 4ax, then
c = \(\frac{a}{m}\)
⇒ -at² = \(\frac{a}{t}\)
⇒ -t³ = 1
⇒ t³ = -1
⇒ t = -1
Substitute the value of t = -1 in (1)
-x – y – a(1) = 0
⇒ x + y + a = 0
Equation of the tangent at L'(a, -2a) to the parabola y² = 4ax is S₁ = 0
⇒ yy₁ – 2a(x + x₁) = 0
⇒ y(-2a) – 2a(x + a) = 0
⇒ y + x + a = 0
⇒ x + y + a = 0
Common tangent to the parabolas touches the parabola y² = 4ax at L'(a, -2a).
Equation of the tangent at L'(-2a, a) to the parabola x² = 4ay is S₁ = 0
⇒ xx₁ – 2a(y + y₁) = 0
⇒ x(-2a) – 2a(y + a) = 0
⇒ x + y + a = 0
∴ Common tangent to the parabolas touches the parabola x² = 4ay at L'(-2a, a).

Question 31.
Show that the tangent at one extremity of a focal chord of a parabola is parallel to the normal at the other extremity.
Solution:
Let, the equation of the parabola is y² = 4ax

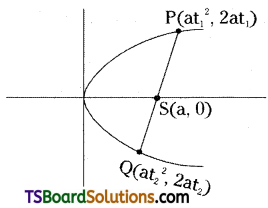
Let P(\(\mathrm{at}_1^2\), 2at₁), Q(\(\mathrm{at}_2^2\), 2at₂) be the two ends of a focal chord of the parabola y² = 4ax, then
t₁t₂ = -1
⇒ -t₂ = \(\frac{1}{t_1}\)
Let \(\frac{1}{t_1}\) = m₁
Slope of the normal at Q(\(\mathrm{at}_2^2\), 2at₂) is
m₂ = -t₂ = \(\frac{1}{t_1}\) = m₁
∴ m₁ = m₂, then the tangent at P and the normal at Q are parallel.

Question 32.
Prove that the normal chord at the point other than the origin whose ordinate is equal to its abscissa subtends a right angle at the focus.
Solution:
Let, the equation of the parabola is y² = 4ax ……..(1)

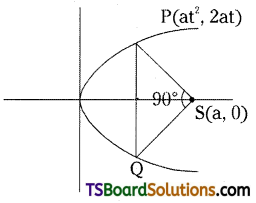
Let P(at², 2at) be any point on the parabola given that, whose ordinate is equal to its abscissa, then
2at = at²
⇒ t² = 2t
⇒ t² – 2t = 0
⇒ t(t – 2) = 0
⇒ t = 0, t = 2
But t ≠ 0, then P(4a, 4a)
The equation of the normal at P(4a, 4a) is
y + xt = 2at + at³
⇒ y + x(2) = 2a(2) + a(2)³
⇒ y + 2x = 4a + 8a
⇒ y + 2x = 12a
⇒ y = 12a – 2x …….(2)
Substituting the value of y = 12a – 2x in (1) we get
(12a – 2x)² = 4ax
⇒ 4(6a – x)² = 4ax
⇒ (6a – x)² = ax
⇒ 36a² + x² – 12ax – ax = 0
⇒ 36a² + x² – 13ax = 0
⇒ x² – 9ax – 4ax + 36a² = 0
⇒ x(x – 9a) – 4a(x – 9a) = 0
⇒ (x – 9a) (x – 4a) = 0
⇒ x – 9a = 0 (or) x – 4a = 0
⇒ x = 9a (or) x = 4a
⇒ x = 4a, 9a
If x = 4a, then y = 12a – 8a = 4a
∴ P = (4a, 4a)
If x = 9a, then y = 12a – 18a = -6a
∴ Q = (9a, -6a)
∴ P = (4a, 4a), Q = (9a, -6a)
Focus S = (a, 0)

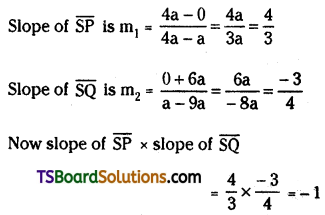
Since m₁m₂ = -1, then
\(\overline{\mathrm{SP}}\) is perpendicular to \(\overline{\mathrm{SQ}}\)
∴ The normal chord subtends a right angle at the focus.

Question 33.
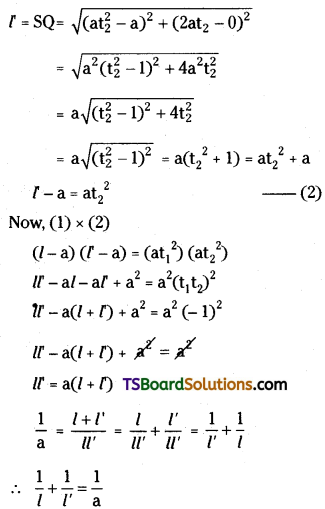
(i) If the coordinates of the ends of a focal chord of the parabola y² = 4ax are (x₁, y₁) and (x₂, y₂) then prove that x₁x₂ = a², y₁y₂ = -4a².
(ii) For a focal chord PQ of the parabola y² = 4ax, if SP = l and SQ = l’ then prove that \(\frac{1}{l}+\frac{1}{l^{\prime}}=\frac{1}{a}\).
Solution:
Given the equation of the parabola is y² = 4ax
Let P(x₁, y₁) = (\(a \mathrm{t}_1^2\), 2at₁) and Q(x₂, y₂) = (\(a \mathrm{t}_2^2\), 2at₂) be two endpoints of a focal chord.

Question 34.
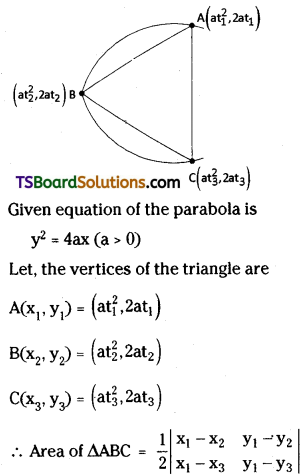
Prove that the area of the triangle inscribed in the parabola y² = 4ax is \(\frac{1}{8a}\) |(y₁ – y₂)(y₂ – y₃)(y₃ – y₁)| sq. units where y₁, y₂, y₃ are the ordinates of its vertices. [(TS) May. ’15]
Solution:

Question 35.
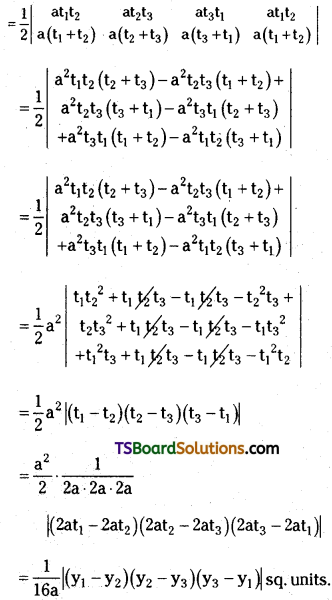
Prove that the area of the triangle formed by the tangents at (x₁, y₁), (x₂, y₂), and (x₃, y₃) to the parabola y² = 4ax (a > 0) is \(\frac{1}{16a}\) |(y₁ – y₂)(y₂ – y₃)(y₃ – y₁)| sq.units. [(AP) Mar. ’18, (TS) ’15]
Solution:
Given parabola y² = 4ax

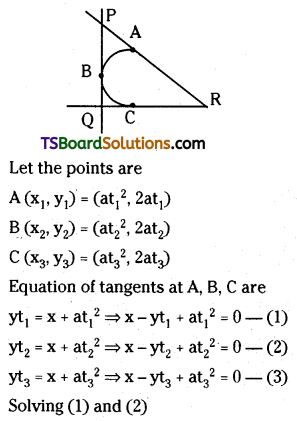
Question 36.
Prove that the orthocentre of the triangle formed by any three tangents to a parabola lies on the directrix of the parabola.
Solution:
Let the equation of the parabola is y² = 4ax
Let A, B, C be the triangle formed by the tangents to the parabola at P(\(\mathrm{at}_1^2\), 2at₁), Q(\(\mathrm{at}_2^2\), 2at₂) and R(\(\mathrm{at}_3^2\), 2at₃) as shown in the figure.

A = Point of intersection of the tangents at P, Q = [at₁t₂, a(t₁ + t₂)]
B = Point of intersection of the tangents at P, R = [at₁t₃, a(t₁ + t₃)]
C = Point of Intersection of the tangents at Q, R = [at₂t₃, a(t₂ + t₃)]
Let, AD and CE be the two altitudes of ∆ABC.
\(\overline{\mathrm{BC}}\) is the tangent at R, then the equation of \(\overline{\mathrm{BC}}\) is yt₃ = x + \(\mathrm{at}_3^2\).


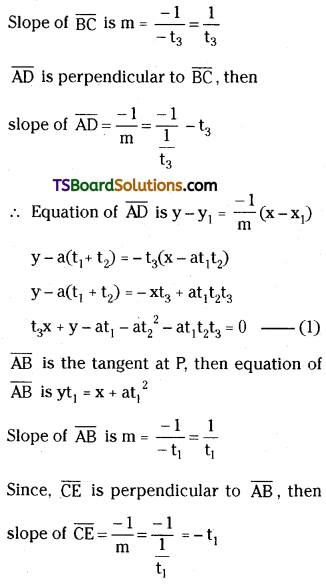
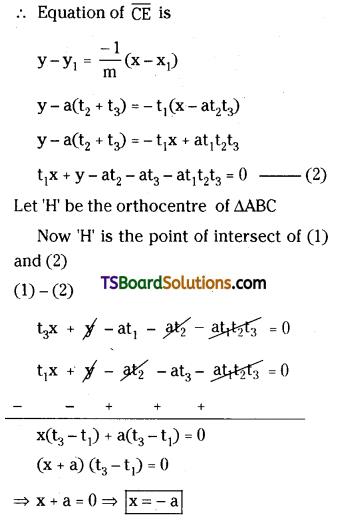
(1) ⇒ t₃(-a) + y – at₁ – at₂ – at₁t₂t₃ = 0
y = a(t₁ + t₂ + t₃ + t₁t₂t₃)
∴ Orthocentre H = [-a, a(t₁ + t₂ + t₃ + t₁t₂t₃)]
The equation of the directrix of the parabola y² = 4ax is x + a = 0
Now substitute H in the directrix
-a + a = 0
⇒ 0 = 0
∴ H lies on the directrix x + a = 0.