Uncategorized

Students must practice these Maths 2A Important Questions TS Inter Second Year Maths 2A Random Variables and Probability Distributions Important Questions Long Answer Type to help strengthen their preparations for exams.

TS Inter Second Year Maths 2A Random Variables and Probability Distributions Important Questions Long Answer Type

Question 1.
The probability distribution of a random variable X is given below:

Find the value of k and the mean and variance of X. [AP – Mar. 2019, AP- Mar. 2017: May ‘14, ’13, May ’14, ’12, ’10].
Solution:
Given that

is the probability distribution of a random variable X.
i) We know that,
\(\) P(X = x_r) = 1
i.e., Sum of probabilities = 1
P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4)+ P(X = 5) = 1
k + 2k + 3k + 4k + 5k = 1
15k = 1
k = \(\frac{1}{15}\)

ii) Mean of X is
μ = \(\sum_{r=1}^5\) P(X = x_r) x_r
= 1 (k) + 2 (2k) + 3 (3k) + (4k) 4 + (5k) 5
= k + 4k + 9k + 16k + 25k = 55 k
= 55(\(\frac{1}{15}\)) = \(\frac{11}{3}\)

iii) Variance of X is
σ² = \(\sum_{r=1}^5\) P(X = x_r) x_r² – μ²
= k(1)² + 2k(2)² + 3k(3)² + 4k (4)² + 5k (5)² – (\(\frac{11}{3}\))²
= k(1) + 2k(4) + 3k(9) + 4k(16) + 5k(25) – \(\frac{121}{9}\)
= k + 8k + 27k + 64k + 125k – \(\frac{121}{9}\)
= 225k – \(\frac{121}{9}\)
= 225 (\(\frac{1}{15}\)) – \(\frac{121}{9}\)
= 15 – \(\frac{121}{9}\)
= \(\frac{135-121}{9}=\frac{14}{9}\).

Question 2.

is the probability distribution of a random variable X. find the value of k and the variance of X.
Solution:

is the probability distribution of a random variable X.
We know that,
\(\sum_{r=-2}^3\) P(X = x_r) = 1
i.e., Sum of probabilities = 1
P(X = – 2) + P(X = – 1) + P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) = 1
0.1 + k + 0.2 + 2k + 0.3 + k = 1
4k + 0.6 = 1
4k = 1 – 0.6
4k = 0.4
k = 0.1
k = \(\frac{1}{10}\)
Mean of X is μ = \(\sum_{r=-2}^3\) (X = x_r) . x_r
= (- 2) (0.1) + (- 1) k + 0(0.2) + 1(2k) + 2(0.3) + 3k
= – 0.2 – k + 0 + 2k + 0.6 + 3k
= 0.4 + 4k
= 0.4 + 4 (\(\frac{1}{10}\))
= 0.4 + 0.4 = 0.8
= \(\frac{8}{10}=\frac{4}{5}\)
Variance of X is σ² = \(\sum_{r=1}^5\) P(X = x_r) x_r² – μ²
= (- 2)² (0.1) + k(- 1)² + (0.2) (0)² + 2k(1)² 0.3(2)² + k(3)² – (\(\frac{4}{5}\))²
= 4 . (0.1) + k . 1 + 0.2 (0) + 2k . 1 + 0.3(4) + k . 9 – \(\frac{16}{25}\)
= 0.4 + k + 0 + 2k + 1.2 + 9k – \(\frac{16}{25}\)
= 1.6 + 12k – \(\frac{16}{25}\)
= \(\frac{16}{10}+\frac{12}{10}-\frac{16}{25}\)
= \(\frac{160+120-64}{100}=\frac{216}{100}\) = 2.16.

Question 3.
A random variable X has the following probability distribution.

Find i) k
ii) the mean and
iii) P(0 < X < 5). [May ’12, ’10, ’08, March ‘09, Board Paper] [AP – May, Mar.16; TS – Mar. ‘18, ‘16]
Solution:

is the probability distribution function.
i) We know that,
\(\sum_{\mathbf{r}=0}^7\) P(X = x) = 1
i.e., Sum of the probabilities = 1
P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4) + P(X = 5) + P(X = 6) + P(X = 7)=
0 + k + 2k + 2k + 3k + k² + 2k² + 7k² + k = 1
10k² + 9k – 1 = 0
10k² + 10k – k – 1 = 0
10k (k + 1) – 1(k + 1) = 0
(k + 1) (10k – 1) = 0
k + 1 = 0 (or) 10k – 1 = 0
k = – 1 (or) k = \(\frac{1}{10}\)
k = – 1 does not satisfy the given probabilities.
∴ k = \(\frac{1}{10}\).

ii) The mean of X is
μ = \(\sum_{r=0}^7\) (X = x_r) . x_r
= 0 . 0 + 1(k) + 2(2k) + 3(2k) + 4(3k)+ 5(k²) + 6(2k²) + 7 (7k² + k)
= 0 + k + 4k + 6k + 12k + 5k² + 12k² + 49k² + 7k
= 66k² + 30k
= \(66\left(\frac{1}{10}\right)^2+30\left(\frac{1}{10}\right)\)
= \(\frac{66}{100}+\frac{30}{10}\)
= 0.66 + 3 = 3.66.

iii) P(0 < X < 5)
= P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4)
= k + 2k + 2k + 3k
= 8k = 8(\(\frac{1}{10}\))
= 0.8 = \(\frac{4}{5}\).

Question 4.
The range of a random variable X is {0, 1, 2}. Given that P(X= 0) = 3c³, P(X = 1) = 4c – 10c², P(X = 2) = 5c – 1. Find
(i) the value of c
(ii) P(X < 1),
(iii) P (1 < X ≤ 2) and P (0 < X ≤ 3). [March ‘13. ‘11, ‘07. ‘05, ‘95; May ‘11. 09. ‘91, TS – Mar. 2015; AP – Mar. 2015]
Solution:
The range of a random variable, X is {O, 1, 2}
Given that,
P(X = 0) = 3c³, P(X = 1) = 4c – 10c²,
P(X = 2) = 5c – 1
ï) We know that,
\(\sum_{\mathrm{r}=0}^2\) P(X = x_r) = 1
i.e., Sum of the probabilities = 1
P(X = 0) + P(X = 1) + P(X = 2) = 1
3c³ + 4c – 10c² + 5c – 1 = 1
3c³ – 10c² + 9c – 2 = 0
Let, f(c) = 3c³ – 10c² + 9c – 2
If c = 1 then,
f(1) = 3(1)³ – 10(1)² + 9(1) – 2
1(1) = 3 – 10 + 9 – 2
= 12 – 12 = 0
∴ c = 1 is a root of 1(c) = 0

3c² – 7c + 2 = 0
3c² – 6c – c + 2 = 0
3c (c – 2) – 1(c – 2) = 0
(c – 2) (3c – 1) = 0
c – 2 = 0 (or) 3c – 1 = 0
c = 2 (or) c = \(\frac{1}{3}\)
∴ c = 1, 2, \(\frac{1}{3}\)
c = 1, 2 does not satisfy the given probabilities.
∴ c = \(\frac{1}{3}\)

ii) P(X < 1) = P(X = 0)
= 3c³
= 3(\(\frac{1}{3}\))³
= \(\frac{3}{27}=\frac{1}{9}\)

iii) P(1 < X ≤ 2)
= P(X = 2)
= 5c – 1
= 5 . \(\frac{1}{3}\) – 1
= \(\frac{5-3}{3}=\frac{2}{3}\)

iv)P(0 < X < 3) = P(X = 1) + P(X = 2)
= 4c – 10c² + 5c – 1
= 9c – 10c² – 1
= 9(\(\frac{1}{3}\)) – 10(\(\frac{1}{9}\)) – 1
= \(\frac{27-10-9}{9}=\frac{8}{9}\).

Question 5.
The range of a random variable X is {1, 2, 3, …………..} and P(X = k) = (k = 1, 2, 3, ………………). Find the value of c and P(0 < X < 3). [TS – May 2015; March 2003, May 2009]
Solution:
The range of a random variable, X is {1, 2, 3, …………..}.
Given that,
P(X = k) = \(\) k = 1, 2, 3 …………….
i) We know that,
\(\) P(X = x_k) = 1
i.e, sum of the probabilities = 1
P(X = 1) + P(X = 2) + P(X = 3) + …………………. = 1
\(\frac{\mathrm{c}}{1 !}+\frac{\mathrm{c}^2}{2 !}+\frac{\mathrm{c}^3}{3 !}+\ldots \ldots\)
\(e^x=1+\frac{x}{1 !}+\frac{x^2}{2 !}+\ldots \ldots\)
\(1+\frac{\mathrm{c}}{1 !}+\frac{\mathrm{c}^2}{2 !}+\frac{\mathrm{c}^3}{3 !}+\ldots \ldots\)
e^c = 2.
c = log_e 2

ii) P(0 < X < 3)
= P(X = 1) + P(X = 2)
= \(\frac{c}{1 !}+\frac{c^2}{2 !}=\frac{c}{1}+\frac{c^2}{2}\)
= log_e 2 + \(\frac{\left(\log _{\mathrm{e}} 2\right)^2}{2}\).

Question 6.
A cubical die is thrown. Find the mean and variance of X, giving the number on the face that shows up. [TS – Mar. 2019] [TS – May 2016; AP – May’ 2015]
Solution:
Let S be the sample space and X be the random variable associated with S, where P(X) is given by the following table.

Mean of X is μ = \(\sum_{r=1}^6\) P(X = x_r) . x_r

Question 7.
If X is a random variable with probability distribution P(X = k) = \(\frac{(k+1) c}{2^k}\), k = 0, 1, 2, …………….. then find c. AP – Mar. 2018;
Solution:
Given that,
P(X = k) = \(\frac{(k+1) c}{2^k}\), k = 0, 1, 2, ………………. is the probability distribution of X.
We know that,
\(\sum_{k=0}^{\infty}\) P(X = x_k) = 1
i.e., sum of the probabilities = 1
P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) + ………………. = 1
\(\frac{1 \cdot c}{2^0}+\frac{2 \cdot c}{2}+\frac{3 \cdot c}{2^2}+\frac{4 \cdot c}{2^3}+\ldots \ldots\) = 1

Question 8.
Let X be a random variable such that P(X = – 2) = P(X = – 1) = P(X = 2) = P(X = 1) = \(\frac{1}{6}\) and P(X = 0) = \(\frac{1}{3}\). Find the mean and variance of X.
Solution:
X is a random variable.
Given that, P(X = – 2) = \(\frac{1}{6}\)
P(X = – 1) = \(\frac{1}{6}\)
P(X = 2) = \(\frac{1}{6}\)
P(X = 1)= \(\frac{1}{6}\)
P (X = 0) = \(\frac{1}{6}\)

Question 9.
Two dice are rolled at random. Find the probability distribution of the sum of the numbers on them. Find the mean of the random variable.
Solution:
When two dice are rolled, the sample space S’ consists of 6 × 6 = 36.
Sample points are
S = {(1, 1), (1, 2), (1, 3), (1,4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6)
(4, 1), (4, 2), 4, 3), (4, 4), (4. 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5,4), (5, 5), (5, 6),
(6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6))
Let X denote the sum of the numbers on the two dice.
Then the range of X is {2, 3, 4, 5, 6, …………………, 12)
The probability distribution for X is given here under:

Question 10.
Find the constant ‘c’, so that F(x) = c \(\left(\frac{2}{3}\right)^x\) x = 1, 2, 3, …….. is the p.d.f. of a discrete random variable X.
Solution:
Given that,
F(x) = c \(\left(\frac{2}{3}\right)^x\), x = 1, 2,3,….. is the probability distribution function.
We know that,
\(\sum_{r=1}^{\infty}\) P(X = x_r) = 1
i.e., sum of the probabilities = 1
P(X = 1) + P(X = 2) + P(X = 3) + …………. = 1
\(c\left(\frac{2}{3}\right)^1+c\left(\frac{2}{3}\right)^2+c\left(\frac{2}{3}\right)^3+\ldots \ldots \ldots\) = 1
\(c\left[\frac{2}{3}+\left(\frac{2}{3}\right)^2+\left(\frac{2}{3}\right)^3+\ldots \ldots\right]\) = 1
Here, a = \(\frac{2}{3}\), r = \(\frac{2}{3}\)
S_∞ = \(\frac{a}{1-r}\)
c\(\left[\frac{\frac{2}{3}}{1-\frac{2}{3}}\right]\) = 1
\(c\left[\frac{\frac{2}{3}}{\frac{1}{3}}\right]\) = 1
c(2) = 1
c = \(\frac{1}{2}\).

Question 11.
In the experiment of tossing a coin n times, if the variable X denotes the number of heads ndP(X=4),P(X=5),P(X=6) are in arithmetic progression then find n. [March 2002]
Solution:
X follows binomial distribution with p = \(\frac{1}{2}\), q = \(\frac{1}{2}\)
Given that,
P(X = 4), P(X = 5), P(X = 6) are in A.P.
Since a, b, c are in A.P. then
∴ 2P(X = 5) = P(X = 4) + P(X = 6)
We have,
P(X = r) = \({ }^n \mathrm{C}_1\) p^rq^1-r

12 (n – 4) = 30 + (n – 5) (n – 4)
12n – 48 = 30 + n² – 9n + 20
n² – 21n + 98 = 0
n² – 14n – 7n + 98 = 0
n (n – 14) – 7(n – 14) = 0
(n – 14) (n – 7) = 0
n – 14 = 0 (or) n – 7 = 0
n = 14 (or) n = 7
∴ n = 7 (or) 14.

Students must practice these Maths 2A Important Questions TS Inter Second Year Maths 2A Permutations and Combinations Important Questions Short Answer Type to help strengthen their preparations for exams.

TS Inter Second Year Maths 2A Permutations and Combinations Important Questions Short Answer Type

Question 1.
If the letters of the word PRlSON are permuted in all possible ways and the words thus formed are arranged in dictionary order, find the rank of the word
i) PRISON.
ii) SIPRON [Mar. ‘14, May’ 13, AP – Mar. 2017]
Solution:
i) The alphabetical order of the letters of the given word is I, N, O, P, R, S.

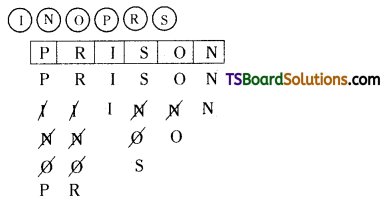
The no. of words begin with I is 5! = 120
The no. of words begin with N is 5! = 120
The no. of words begin with O is 5! = 120
The no. of words begin with Pl is 4! = 24
The no. of words begin with PN is 4! = 24
The no. of words begin with PN is 4! = 24
The no. of words begin with PRlN is 2! = 2
The no, of words begin with PRIO is 2! = 2
The no. of words begin with PRISN is 1! = 1
The next word is PRISON = 1
∴ Rank of the word, PRISON = 120 + 120 + 120 + 24 + 24 + 24 + 2 + 2 + 1 + 1 = 438.

ii) The alphabetical order of the letters of the given word is I, N, O, P, R, S.

The no. of words begin with L is 5! = 120
The no. of words begin with N is 5! = 120
The no. of words begin with Ois 5! = 120
The no. of words begin with P is 5! = 120
The no. of words begin with R is 5! = 120
The no. of words begin with SIN is 3! 6
The no. ol words begin with SlO is 3! = 6
The no. of words begin with SIPN is 2! = 2
The no. of words begin with SIPO is 2! = 2
The no. of wor(ls begin with SIPRN is 1! = 1
The next word is SIPRON = 1
∴ The Rank of SIPRON is 120 + 120 + 120 + 120 + 120 + 6 + 6 + 2 + 2 + 1 + 1 = 618.

Question 2.
If the letters of the word MASTER are permuted In all possible ways and the words thus formed are arranged in the dictionary order, then find the ranks of the word REMAST. [March ‘08, Board Paper].
Solution:

The alphabetical order of the letters of the given word is A, E. M, R, S, T.
The no. of words begin with A is 5! = 120
The no. of words begin with E is 5! = 120
The no. of words begin with M is 5! = 120
The no. of words begin with RA is 4! = 24
The no. of words begin with REA is 3! = 6
The next word is REMAST = 1
∴ The Rank of the word, REMAST = 120 + 120 + 120 + 246 + 1 = 391.

Question 3.
If the letters of the word MASTER are permuted in all possible ways and the words thus fonned are arranged in the dictionary order, then find the ranks f the word MASTER. [AP & TS – Mar. 2019] [May ‘11, ‘10, ‘07,’06; March ‘11,’09, ‘08, TS – Mar. 2016; AP – Mar., May 2015].
Solution:

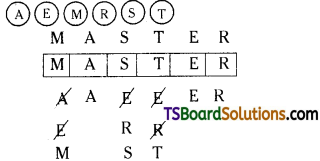
The alphabetical order of the letters of thi given word is A, E, M, R, S, T.
The no. of words begin with A is 5! = 120
The no. of words begin with E is 5! = 120
The no. of words begin with MAE is 3! = 6
The no. of words begin with MAR is 3! = 6
The no. of words begin with MASE is 2! = 2
The no. of words begin with MASR is 2! = 2
The next word is MASTER = 1
The Rank of the word, MASTER = 120 + 120 + 6 + 6 + 2 + 2 + 1 = 257.

Question 4.
Find the sum of all 4-digit numbers that can be formed using the digits 1, 3, 5, 7, 9. [AP & TS – Mar. 18; May ‘14, Mar.13]
Solution:
The no. of four digited number formed by using the digits 1, 3, 5, 7, 9 without repetition = \({ }^n P_r={ }^5 P_4\)
= 5 . 4. 3 . 2 = 120.
Out of these 120 numbers, \({ }^n P_r={ }^5 P_4\) number contain 1 in units place.
\({ }^4 \mathrm{P}_3\) numbers contain 1 in Ten’s place.
\({ }^4 \mathrm{P}_3\) numbers contain 1 in hundred’s place.
\({ }^4 \mathrm{P}_3\) numbers contain 1 in thousand’s place.

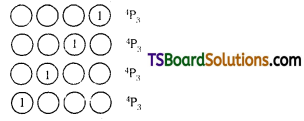
∴ The value obtained by adding (1) in all the numbers = \({ }^4 \mathrm{P}_3\) × 1 × 1 + \({ }^4 \mathrm{P}_3\) × 1 × 10 + \({ }^4 \mathrm{P}_3\) × 1 × 100 + \({ }^4 \mathrm{P}_3\) × 1 × 1000
= \({ }^4 \mathrm{P}_3\) × 1 + \({ }^4 \mathrm{P}_3\) × 10 + \({ }^4 \mathrm{P}_3\) × 100 + \({ }^4 \mathrm{P}_3\) × 1000
= \({ }^4 \mathrm{P}_3\) × 1 [1 + 10 + 100 + 1000]
= \({ }^4 \mathrm{P}_3\) × 3 × (1111)

Similarly the value obtained by adding (3) is \({ }^4 \mathrm{P}_3\) × 3 × 1111
The value obtained by adding (5) is \({ }^4 \mathrm{P}_3\) × 5 × 1111
The value obtained by adding (7) is \({ }^4 \mathrm{P}_3\) × 7 × 1111
The value obtained by adding (9) is \({ }^4 \mathrm{P}_3\) × 9 × 1111
The sum of all the numbers = \({ }^4 \mathrm{P}_3\) × 1 × (1111) + \({ }^4 \mathrm{P}_3\) × 3 × (1111) + \({ }^4 \mathrm{P}_3\) × 5 × (1111) + \({ }^4 \mathrm{P}_3\) × 7 × (1111) + \({ }^4 \mathrm{P}_3\) × 9 × (1111)
= \({ }^4 \mathrm{P}_3\) (1111) [1 + 3 + 5 + 7 + 9]
= 24 (1111) (25) = 6,66,600.

Question 5.
Find the sum of aIl 4 digited numbers that can be formed using the digits 1, 2, 4, 5, 6 without repetition. [March ’10]
Solution:
The number of 4 digited numbers formed by using the digits 1, 2, 4, 5, 6 without repetition = \({ }^5 \mathrm{P}_4\)
= 5 . 4 . 3 . 2 = 120
Out of these 120 numbers,
\({ }^4 \mathrm{P}_3\) numbers contain 1 in units places
\({ }^4 \mathrm{P}_3\) numbers contain 1 in ten’s place
\({ }^4 \mathrm{P}_3\) numbers contain 1 in hundred’s place
\({ }^4 \mathrm{P}_3\) numbers contain 1 in thousand’s place

∴ The value obtained by adding (1) in all the numbers
= \({ }^4 \mathrm{P}_3\) × 1 × 1 + \({ }^4 \mathrm{P}_3\) × 1 × 10 + \({ }^4 \mathrm{P}_3\) × 1 × 100 + \({ }^4 \mathrm{P}_3\) × 1 × 1000
= \({ }^4 \mathrm{P}_3\) × 1 [1 + 10 + 100 + 1000]
= \({ }^4 \mathrm{P}_3\) × 1 × 1111
Similarly the value obtained by adding (2) is \({ }^4 \mathrm{P}_3\) × 2 (1111)
The value obtained by adding (4) is \({ }^4 \mathrm{P}_3\) × 4 (1111)
The value obtained by adding (5) is \({ }^4 \mathrm{P}_3\) × 5 (1111)
The value obtained by adding (6) is \({ }^4 \mathrm{P}_3\) × 6 (1111)
∴ The sum of all the numbers = \({ }^4 \mathrm{P}_3\) x 1×1111 + \({ }^4 \mathrm{P}_3\) x2(1111) + \({ }^4 \mathrm{P}_3\) x4(1111) + \({ }^4 \mathrm{P}_3\) x5(1111)+ \({ }^4 \mathrm{P}_3\)x6(1111)
= \({ }^4 \mathrm{P}_3\) (1111) + (1 + 2 + 4 + 5 + 6)
= \({ }^4 \mathrm{P}_3\) (1111) (18)
= 4 . 3 . 2 (1111) (18)
= 24 (1111) 18
= 479952.

Question 6.
Find the number of numbers that are greater than 4000 which can be formed using the digits 0, 2, 4,6, 8 without repetition. [May ‘09]
Solution:
From the given digits, 0 cannot be placed in the first place. So, 1st place can be filled with the remaining four digits.
All the numbers of five digits are greater than 4000.
4 digit numbers:

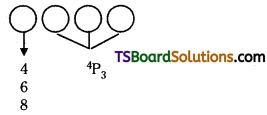
In four digit numbers the number starting 4 with 4 or 6 or 8 are greater than 4000.
The number of 4 digit numbers which begin with 4 or 6 or 8 = 3 . \({ }^4 \mathrm{P}_3\)
= 3 . 4 . 3 . 2 = 72

5 digit numbers:

The number of five digit numbers = 4 . \({ }^4 \mathrm{P}_4\)
= 4 . 4 . 3 . 2 . 1 = 96
∴ The number of numbers greater than 4000 = 72 + 96 = 168.

Question 7.
Find the number of ways of arranging 5 different mathematics hooks, 4 different physics books and 3 different chemistry books such that the books of the same subject are together.
Solution:

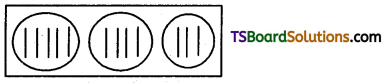
The number of ways of arranging three bundles of Maths, Physics and Chemistry books is \({ }^3 \mathrm{P}_3\) = 3!.
5 different Mathematics books in the bundle are arranged among themselves in 5! ways.
4 different Physics books in the bundle are arranged among themselves in 4! ways.
3 different Chemistry books in the bundle are arranged among themselves in 3! ways.
∴ The required number of arrangements = 3! . 5! . 4! . 3!
= 6 . 120 . 24 . 6 = 103684.

Question 8.
Find the number of ways of arranging 7 gents and 4 ladies around a circular table if no two ladles wish to sit together. [May ‘07]
Solution:
First arrange 7 gents around a circle in (7 – 1)! = 6! ways.

Now arrange 4 ladies in 7 positions in \({ }^7 \mathrm{P}_4\) ways.
∴ The required number of arrangements = 6! . \({ }^7 \mathrm{P}_4\)
= 6 . 5 . 4 . 3 . 2 . 1 . 7 . 6 . 5 . 4 = 604800.

Question 9.
Find the number of ways of arranging the letters of word SINGING so that
i) they begin and end with I
ii) the two G’s come together.
Solution:
The given word has 7 letters ¡n which there are 21’s are alike, 2N’s are alike. 2G’s are alike, 1S is different.
i) They begin and end with I:

First we fill the first and last places with I’s in \(\frac{2 !}{2 !}\) ways.
Now, we fill the remaining 5 places with the remaining 5 letters in \(\frac{5 !}{2 ! 2 !}\) ways.
∴ The number of required arrangments is \(\frac{5 !}{2 ! 2 !} \cdot \frac{2 !}{2 !}=\frac{5 \cdot 4 \cdot 3 \cdot 2 \cdot 1}{2 \cdot 2}\) = 30.

ii) The 2 G’s come together:
Treat the 2Gs as one unit.
Then we have 6 letters in which there are 2I’s and 2N’s.
They can be arranged in \(\frac{6 !}{2 ! 2 !}\) ways.
Now, the 2G’s can be arranged among themselves in \(\frac{2 !}{2 !}\).
∴ The number of required arrangments is \(\frac{6 !}{2 ! 2 !} \cdot \frac{2 !}{2 !}\) = 180.

Question 10.
If the letters of the word EAMCET are permuted in all possible ways and 1f the words they formed are arranged In the dictionary order, find the rank of the word EAMCET. [TS – May 2015; Mar. ‘12] [AP – May, Mar. 2016; TS – Mar. 2017, May ’16]
Solution:
Given word EAMCET,
The alphabetical order of the letters of the, ACEEMT.
The no. of words begin with A = \(\frac{5 !}{2 !}\) = 60
The no. of words begin with C = \(\frac{5 !}{2 !}\) = 60

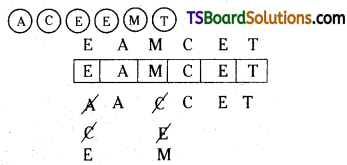
The no. of words begin with EAC = 3! = 6
The no. of words begin with EAE = 3! = 6
The next word is EAMCET = 1
Rank of word EAMCET = 60 + 60 + 6 + 6 + 1 = 133.

Question 11.
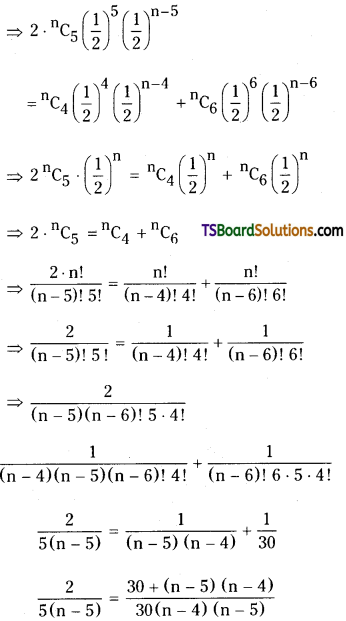
If 1 ≤ r ≤ n,then show that \({ }^n C_{r-1}+{ }^n C_r={ }^{(n+1)} C_r\). [March’11, May ‘9]
Solution:

Question 12.
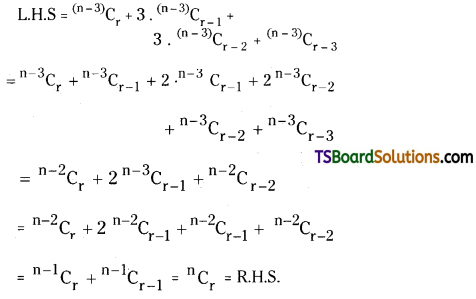
Prove that 3 ≤ r ≤ n, \({ }^{(n-3)} C_r+3 \cdot{ }^{(n-3)} C_{(r-1)}+3 \cdot{ }^{(n-3)} C_{(r-2)}\) + \({ }^{(n-3)} C_{(r-3)}={ }^n C_r\). [May ’12, ’09]
Solution:

Question 13.
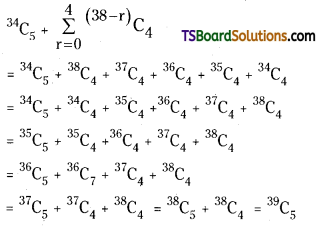
Simplify \({ }^{34} C_5+\sum_{r=0}^4(38-r) C_4\). [AP – Mar. ’19, ’17, ’16; March ’12, May ’11].
Solution:

Question 14.
Find the number of ways of selecting 3 girls and 3 boys out of 7 girls and 6 boys. [May ’09]
Solution:
The no. of ways of selecting 3 girls and 3 boys out of 7 girls and 6 boys = \({ }^7 \mathrm{C}_3 \cdot{ }^6 \mathrm{C}_3\)
= \(\frac{7 \cdot 6 \cdot 5}{1 \cdot 2 \cdot 3} \cdot \frac{6 \cdot 5 \cdot 4}{3 \cdot 2 \cdot 1}\) = 700

Question 15.
Find the number of ways of selecting 3 vowels and 2 consonants from the letters of the word EQUATION. [May ’11, March ’07]
Solution:
The word EQUATION contains 5 vowels and 3 consonants.
The 3 vowels can be selected from 5 vowels in \({ }^5 \mathrm{C}_3\) ways.
The 2 consonants can be selected from 3 consonants in \({ }^3 \mathrm{C}_2\) ways.
∴ The required no. of ways of selecting 3 vowels and 2 consonants = \({ }^5 \mathrm{C}_3 \cdot{ }^3 \mathrm{C}_2\)
= \(\frac{5 \cdot 4 \cdot 3}{3 \cdot 2 \cdot 1} \cdot \frac{3 \cdot 2}{2 \cdot 1}\) = 30.

Question 16.
Prove that \(\frac{{ }^{4 n} C_{2 n}}{{ }^{2 n} C_n}=\frac{1 \cdot 3 \cdot 5 \ldots \ldots(4 n-1)}{\{1 \cdot 3 \cdot 5 \ldots \ldots(2 n-1)\}^2}\). [TS – Mar. ’19, May ’08, 2000, Mart. ’98] [AP – Mar. 2017, 2015, TS – May 2015].
Solution:

Question 17.
Find the number of ways of selecting a cricket team of 11 players from 7 batsmen and 6 bowlers such that there will be atleast 5 bowlers in the team. [May ’14, ’10, March ’05, Board Paper AP – May 2016; TS – Mar. 18, May 2016]
Solution:
Total number of players in the team = 11.

1) 6 Batsmen, 5 bowlers:
The no. of ways of selecting a cricket team = \({ }^7 \mathrm{C}_6 \cdot{ }^6 \mathrm{C}_5\)
= 7 . 6 = 42

2) 5 Batsmen, 6 bowlers:
The no. of ways of selecting cricket team = \({ }^7 \mathrm{C}_5 \cdot{ }^6 \mathrm{C}_6\)
= \(\frac{7 \cdot 6}{2 \cdot 1}\) . 1
= 7 . 3 = 21.

Question 18.
Find the number of ways of forming a committee of 5 members out of 6 Indians and 5 Americans so that always the Indians will be in majority in the committee. [TS – Mar. 2015; March ‘13. ’09, ’08]
Solution:
Since, the committee must contain always the Indians will be in majority, the members of the committee may be the following.

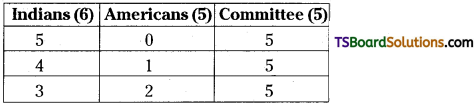
i) 5 Indians, 0 Americans.
The no. of ways of selecting committee = \({ }^6 \mathrm{C}_5 \cdot{ }^5 \mathrm{C}_0\)
=6×1 = 6

ii) 4 Indians, 1 American:
The no. of ways of selecting committee = \({ }^6 \mathrm{C}_4 \cdot{ }^5 \mathrm{C}_1\)
= \(\frac{6 \cdot 5}{2 \cdot 1}\) . 5 = 75

iii) 3 Indians, 2 Americans.
The no. of ways of selecting committee = \({ }^6 \mathrm{C}_3 \cdot{ }^5 \mathrm{C}_2\)
= \(\frac{6 \cdot 5 \cdot 4}{3 \cdot 2 \cdot 1} \cdot \frac{5 \cdot 4}{2 \cdot 1}\) = 200
The required no. of ways of selecting j committee = 6 + 75 + 200 = 281.

Some More Maths 2A Permutations and Combinations Important Questions

Question 19.
If \({ }^n \mathrm{P}_3\) = 1320, find n. [May ’08, March ’10, ’07]
Solution:
Given \({ }^n \mathrm{P}_3\) = 1320 = 12 . 11 . 10
\({ }^n P_3={ }^{12} P_3\)
⇒ n = 12.

Question 20.
If \((n+1) P_5:{ }^n P_6\) = 2 : 7, find n.
Solution:
Given \(\frac{(n+1)}{{ }^n P_5}=\frac{2}{7}\)
\(\frac{(n+1) n(n-1)(n-2)(n-3)}{n(n-1)(n-2)(n-3)(n-4)(n-5)}=\frac{2}{7}\)
\(\frac{(n+1)}{(n-4)(n-5)}=\frac{2}{7}\)
7n + 7 = 2 (n² – 5n – 4n + 20)
7n + 7 = 2n² – 18n + 40
2n² – 18n – 7n + 40 – 7 = 0
2n² – 25n + 33 = 0
2n² – 22n – 3n + 33 = 0
2n (n – 11) – 3 (n – 11) = 0
(n – 11) (2n – 3) = 0
n = 11; n = \(\frac{3}{2}\)
Since, n is a integer then n = 11.

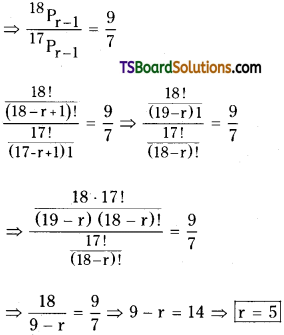
Question 20.
If \({ }^{18} P_{(r-1)}:{ }^{17} P_{(r-1)}\) = 9 : 7, find ‘r’.
Solution:
Given, \({ }^{18} \mathrm{P}_{\mathrm{r}-1}:{ }^{17} \mathrm{P}_{\mathrm{r}-1}\) = 9 : 7

Question 21.
Find the number of different chains that can be prepared using 7 dIfferent coloured beads.
Solution:
Total number of beads n = 7
The no. of different chains that can be prepared using 7 different coloured beads is = \(\frac{(7-1) !}{2}=\frac{6 !}{2}\) = 360.

Question 22.
Prove that \({ }^{25} C_4+\sum_{r=0}^4(29-r) C_3={ }^{30} C_4\). [AP – Mar. 2018]
Solution:

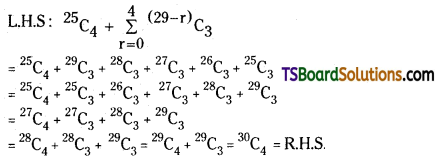
Question 23.
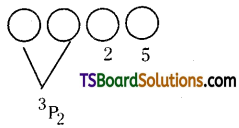
Find the number of ways of arranging 5 boys and 4 girls in a row so that the row begins and ends with boys.
Solution
The total no. of persons is 9. (5 boys + 4 girls)

Let us take 9 blank’s,
First, we fill the first and last places with boys.
This can be done in \({ }^5 \mathrm{P}_2\) ways.
Now, we have to fill up the remaining 7 places with the remaining 7 persons (3 boys + 4 girls) In 7! ways.
The required no. of arrangements is = \({ }^5 \mathrm{P}_2\) . 7!
= 20 × 5040 = 100800.

Question 24.
In how many ways 9 mathematics papers can be arranged so that the best and the worst (i) may come together (ii) may not come together?
Solution:
i) If the best and worst papers are treated as one unit, then we have 9 – 2 + 1 = 7 + 1 = 8 papers.
Now these can be arranged in (7 + 1)! ways and the best and worst papers between themselves can be permuted in 2! ways.
Therefore the number of arrangements in which best and worst papers come twet her is 8! 2!.

ii) Total number of ways of arranging 9 mathematics papers is 9!.
The best and worst papers come together in 8! . 2! ways.
Therefore the number of ways they may not come together is
9! – 8! 2! = 8! (9 – 2) 8! × 7.

Question 25.
Find the number of ways of arrangIng 6 boys and 6 girls ¡n a row. In how many of these arrangements
i) all the girls are together
ii) no two girls are together
iii) boys and girls come alternately?
Solution:
6 boys + 6 girls = 12 persons.
They can be arranged in a row in (12)! ways.

i) Treat the 6 girls as one unit. Then we have 6 boys + 1 unit of girls.
They can be arranged in 7! ways.
Now, the 6 girls among themselves can be permuted in 6! ways.
Hence, by the fundamental principle, the number of arrangements in which all 6 girls are together 7! x 6!.

ii) First we arrange 6 boys in a row in 6! ways.
The girls can be arranged in the 7 gaps between the boys.
These gaps are shown below by the letter X.

Now, the girls can be arranged in these 7 gaps in \({ }^7 \mathrm{P}_6\) ways.
Hence, by the fundamental principle, the number of arrange ments in which no two girls corner together is 6! × 7P6
= 6! × 7!
= 7 × 6! × 6!.

iii) Let us take 12 places. The row may begin with either a boy or a girl. That is, 2 ways.
If it begins with a boy, then all odd places (1, 3, 5, 7, 9, 11) will be occupied by boys and the even places (2, 4, 6, 8, 10, 12) by girls.
The 6 boys can be arranged in the 6 odd places in 6! ways and the 6 girls can be arranged in the 6 even places in 6! ways.
Thus the number of arrangements in which boys and girls come alternately is 2 x 6! x 6!.

Note :
In the above, one may think that questions (ii) and (iii) are same. But they are not (as evident from the answers).
In Question (ii), after arranging 6 boys, we found 7 gaps and 6 girls are arranged in these 7 gaps.
Hence one place remains vacant.
It can be any one of the 7 gaps. But in Question (iii), the vacant place should either be at the beginning or at the ending but not in between.
Thus, only 2 choices for the vacant place.

Question 26.
Find the number of 4 letter words that can be formed using the letters of the word MIRACLE. How many of them
i) begin with an vowel
ii) begin and end with vowels
iii) end with a consonant ?
Solution:
The word MIRACLE has 7 letters. Hence the number of 4 letter words that can be formed using these letters is \({ }^7 \mathrm{P}_4\) = 7 × 6 × 5 × 4 = 840.
Let us take 4 blanks.

i) We can fill the first place with one of the 3 vowels (I, A, E) in \({ }^3 \mathrm{P}_1\) = 3 ways.
Now, the remaining 3 places can be filled using the re¬maining 6 letters in \({ }^6 \mathrm{P}_3\) = 120 ways.
Thus the number of 4 letter words that begin with an vowel is 3 × 120 = 360.

ii) Fill the first and last places with 2 vowels in \({ }^3 \mathrm{P}_2\) = 6 ways.
The remaining 2 places can be filled with the remaining 5 letters in \({ }^5 \mathrm{P}_2\) = 20 ways.
Thus the number of 4 letter words that begin and end with vowels is 6 × 20 = 120.

iii) We can fill the last place with one of the 4 consonants (M, R, C, L) in \({ }^4 \mathrm{P}_1\) = 4 ways.
The remaining 3 places can be filled with the letters in \({ }^6 \mathrm{P}_3\) ways.
Thus the number of 4 letter words that end with an vowel is 4 × \({ }^6 \mathrm{P}_3\) = 4 × 120 = 480.

Question 27.
Find the number of ways of permuting the letters of the word PICTURE so that
i) all vowels come together
ii) no two vowels come together.
iii) the relative positions of vowels and consonants are not disturbed.
Solution:
The word PICTURE has 3 vowels (I, U, E) and 4 consonants (P, C, T, R).
i) Treat the 3 vowels as one unit. Then we can arrange 4 consonants + 1 unit of vowels in 5! ways. Now the 3 vowels among themselves can be permuted in 3! ways. Hence the number of per¬mutations in which the 3 vowels come together is 5! × 3! = 720.

ii) First arrange the 4 consonants in 4! ways. Then in between the vowels, in the beginning and in the ending, there are 5 gaps as shown below by the letter X.

In these 5 places we can arrange the 3 vowels \({ }^5 \mathrm{P}_3\) ways.
Thus the number of words in which no two vowels come together is 4! × \({ }^5 \mathrm{P}_3\)
= 24 × 60 = 1440.

iii) The three vowels can be arranged in their relative positions in 3! ways and the 4 consonants can be arranged in their relative positions in 4! ways.

The required number of arrangements is 3! . 4! = 144.

Note :
In the above problem, from (i) we get that the number of permutations in which the vowels do not come together is
= Total number of permutations – number of permutations in which 3 vowels come together.
= 7! – 5! . 3!
= 5040 – 720 = 4320.
But the number of permutations in which no two vowels come together is only 1440.
In the remaining 2880 permutations two vowels come together and third appears away from these.

Question 28.
Find the number of 4-digit numbers that can be formed using the digits 2, 3, 5, 6, 8 (without repetition). How many of them are divisible by
i) 2
ii) 3
iii) 4
iv) 5
v) 25
Solution:

The number of 4 digit numbers that can be formed using the 5 digits 2, 3, 5, 6, 8 is \({ }^5 \mathrm{P}_4\) = 120.

i) divisible by 2 :

A number is divisible by 2, when its unit place must be filled with an even digits (2 or 6 or 8) from among the given integers. This can be done in three ways.
Now, the remaining three places can be filled with remaining four digits in \({ }^4 P_3\) = 4 . 3 . 2 = 24 ways.
∴ The no. of 4 digited numbers divisible by 2 = 3 . 24 = 72

ii) Divisible by 3:
A number is divisible by 3 only when sum of the digits in that number is a multiple of 3.
Sum of the given 5 digits = 2 + 3 + 5 + 6 + 8 = 24.
The 4 digits such that, their sum is a multiple of 3 from the given digits are 2, 3, 5, 8 (or) 2, 5, 6, 8.
In each case, we can permute (arrange) in 4! ways.
∴ The no. of 4 digited numbers divisible by 3 = 2 . 4!
= 2 . 4 . 3 . 2 . 1 = 48.
∴ The no. of 4 digited numbers divisible by 3 = 48.

iii) Divisible by 4 :

A number is divisible by 4.
Hence, the last two places should be filled with one of the following 28, 32, 36, 52, 56, 68.
Thus, the last two places can be filled in 6 ways.
The remaining two places can be filled by remaining three digits in \({ }^3 \mathrm{P}_2\) = 3 . 2 = 6 ways.
The number of 4 digited number divisible by 4 = 6 × 6 = 36.

iv) Divisible by 5 :

A number is divisible by 5 when its units place must be filled by 5 from the given integers 2, 3, 5, 6, 8.
This can be done in one way.
The remaining three places can be filled with remaining 4 digits in 4P3 ways = 4 . 3 . 2 = 24 ways.
∴ The number of 4 digited number divisible by 5 = 1 × 24 = 24.

v) Divisible by 25 :

A number is divisible by 25, when its last two places are filled with 25.
Thus, the last two places can be filled in one way.
The remaining two places from the remaining 3 digits can be filled in \({ }^3 \mathrm{P}_2\) = 6 ways.
∴ The number of 4 digited number divisible by 25 = 1 × 6 = 6.

Question 29.
If the letters of the word BRING are per-muted in all possible ways and the words thus formed are arranged in the dictionary order, then find the 59th word.
Solution:
Given word is BRING.
∴ The alphabetical order of the letters is B, G, I, N, R.
In the dictionary order, first we write all words beginning with B.
Clearly the number of words beginning with B are 4! = 24.
Similarly the number of words begin with G are 4! = 24.
Since the words begin with B and G sum to 48, the 59th word must start with I.
Number of words given with IB = 3! = 6
Hence the 59th word must start with IG. Number of words begin with IGB = 2! = 2
Number of words begin with IGN = 2! = 2
∴ Next word is 59th = IGRBN.

Question 30.
Find the number of 5-letter words that can be formed using the letters of the word MIXTURE which begin with an vowel when repetitions are allowed.
Solution:
We have to fill up 5 blanks using the letters of the word MIXTURE having 7 letters among which there are 3 vowels. Fill the first place with one of the vowels (I or U or E) in 3 ways as shown below.

Each of the remaining 4 places can be filled in 7 ways (since we can use all 7 letters each time).
Thus the number of 5 letter words is 3 × 7 × 7 × 7 × 7 = 3 × 7⁴.

Question 31.
Find the number of 4 – digit numbers that can be formed using the digits 1, 2, 3, 4, 5, 6, that are divisible by
i) 2
ii) 3 when repetition is allowed.
Solution:
i) Numbers divisible by 2:

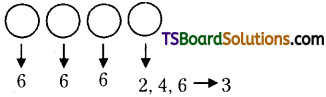
For a number to be divisible by 2.
The units place should be filled with an even digit (2 or 4 or 6).
This can be done in 3 ways.
Now, each of the remaining 3 places can be filled in 6 ways.
∴ The number of 4 digit numbers that are divisible by 2 is 8.

ii) Numbers divisible by 3 :

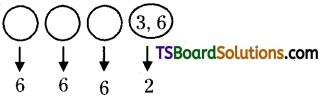
Fill the first three places with the given 6 digits in 6³ ways.
Now, after filling up the first three places with three digits if we fill up the unit’s place in 6 ways we get 6 consecutive positive integers.
Out of any six consecutive integers exactly two are divisible by 3.
Hence, the unit’s place can be filled in two ways.
∴ The number of 4 digit numbers divisible by 3 is
2 . 6³ = 2 . 216 = 432.

Question 32.
Find the number of 5 – letter words that! can be formed using the letters of the word EXPLAIN that begin and end with a vowel when repetitions are allowed.
Solution:

We can fill the first and last places with vowels each in 3 ways (E, A, I).
Now, each of the remaining three places can be filled in 7 ways (when repetitions are allowed).
∴ The number of 5 letter words which begin and end with vowels is 3² . 7³
= 9 . 343 = 3087.

Question 33.
Find the number of ways of arranging 8 persons around a circular table if two particular persons were to sit together.
Solution:
Treat the two particular persons as one unit.
Then we have 6 + 1 = 7 things.
They can be arranged around a circular table in (7 – 1) ! = 6!
Now, the two particular persons can be arranged among themselves in 2! ways.
∴ The number of required arrangements is 6! – 2! = 6 . 5 . 4 . 3 . 2 . 1 . 2 = 1440.

Question 34.
Find the number of ways of arranging 8 men and 4 women around a circular table. In how many of them
i) all the women come together
ii) no two women come together.
Solution:
Total number of persons = 12 [8M + 4W]
∴ The number of ways of arranging these 12 persons around a circular table = (12 – 1)1 = 11!
i) All the women come together :
Treat the four women as one unit then we have 8 men + 1 unit of women then we have 9 entries.
They can be arranged around a circular table in (9 – 1) ! = 8! ways.
Now, the four women among themselves can be arranged in 4! ways.
The required number of arrangements is 8! . 4!.

ii) No two women come together :

First we arrange 8 men around the circular table in (8 – 1) ! = 7 ! ways.
There are 8 places in between them.
Now, we can arrange the four women in these 8 places in \({ }^8 \mathrm{P}_4\) ways.
The required number of arrangements is 7 ! . \({ }^8 \mathrm{P}_4\).

Question 35.
Find the number of ways of seating 5 Indians, 4 Americans and 3 Russians at a round table so that
i) all Indians sit together
ii) no two Russians sit together
iii) persons of same nationality sit together.
Solution:
i) All Indians sit together :
Treat the five Indians as one unit.
Then we have 4 Americans + 3 Russians + 1 unit of Indians = 8 entries.
They can be arranged at a round table in (8 – 1) ! = 7 ! ways.
Now, the five Indians among themselves can be arranged in 5! ways.
∴ The required number of arrangements is 7! – 5!.

ii) Now two Russians sit together :
First we arrange the 5 Indians + 4 Americans around the table in (9 – 1)! = 8! ways.
Now, there are 9 gaps in between 9 persons.
The 3 Russians can be arranged in the 9 gaps in \({ }^9 P_3\).
∴ The required number of arrangements is 8! . \({ }^9 P_3\).

Persons of same nationality sit together:
Treat the 5 Indians as one unit, the four Americans as one unit and the 3 Russians as one unit.
These 3 units can be arranged at round table in (3 – 1)1 = 2! ways.
Now, the 5 Indians among themselves can be arranged in 5! ways.
The four Americans among themselves can be arranged in 4! ways.
The 3 Russians among themselves can be arranged in 3! ways.
The required number of arrangements = 2! . 5! . 3! . 4!.

Question 36.
Find the number of ways of arranging the letters of the word SPECIFIC. In how many of them
i) the two ‘C’, ‘S’ come together,
ii) the two I’s do not come together.
Solution:
The given word has eight letters in which there are 2 I’s are alike and 2 C’s are alike and rest are different.
They can be arranged in \(\frac{8 !}{2 ! 2 !}\) ways.
\(\frac{8 \cdot 7 \cdot 6 \cdot 5 \cdot 4 \cdot 3 \cdot 2 \cdot 1}{2 \cdot 2}\) = 10080.

i) the two C’s come together:
Treat the 2 C’s as one unit.
Then we have 6 + 1 = 7 letters.
In which 2 I’s are alike.
Now, they can be arranged in \(\frac{7 !}{2 !}\) ways.
The 2 C’s can be arranged among themselves in \(\frac{2 !}{2 !}\) ways.
∴ The number of required arrangements = \(\frac{7 !}{2 !} \cdot \frac{2 !}{2 !}\) = 2520.

ii) The 2I’s do not come together:
Since, the 2 I’s do not come togeter, then first arrange the remaining six letters in \(\frac{6 !}{2 !}\) ways.
Among these 6 letters we find 7 gaps.
Now, the 2 I’s can be arranged in these 7 gaps in \(\frac{{ }^7 \mathrm{P}_2}{2 !}\) ways.
∴ The number of required arrangements = \(\frac{6 !}{2 !} \cdot \frac{{ }^7 \mathrm{P}_2}{2 !}\)
= \(\frac{6 \cdot 5 \cdot 4 \cdot 3 \cdot 2 \cdot 1}{2} \cdot \frac{7 \cdot 6}{2}\) = 7560

Question 37.
Find the number of 5- digit numbers that can be formed using the digits 1, 1, 2, 2, 3. How many of them are even?
Solution:
In the given 5 digits there are two 1’s and two 2’s.
They can be arranged in \(\frac{5 !}{2 ! 2 !}\) ways
= \(\frac{5 \cdot 4 \cdot 3 \cdot 2 \cdot 1}{2 \cdot 2}\) = 30
Now, the remaining 4 places can be arranged using the remaining digits 1, 1, 2, 3 in \(\frac{4 !}{2 !}\) ways = 12.
The number of 5 digit even numbers that can be formed using the digits 1, 1, 2, 2, 3 is 12.

Question 38.
Find the number of ways of arranging the letters of the words if
i) SINGING
ii) PERMUTATION
iii) COMBINATION
Solution:
i) SINGING: Given word is ‘SINGING’.
The word SINGING contains 7 letters in which there are 2 I’s are alike, 2 N’s are alike and 2G’s are alike, and rest are different.
∴ The number of required arrangements = \(\frac{7 !}{2 ! 2 ! 2 !}\).

ii) PERMUTATION:
Given word is ‘PERMUTATION’.
The word ‘PERMUTATION’ contains 11 letters in which there are 2 T’s are alike and rest are different.
∴ The number of required arrangements = \(\frac{11 !}{2 !}\)

iii) COMBINATION:
Given word is COMBINATiON.
The word ‘COMBiNATION’ contains 11 letters in which there are 2 O’s are alike, 2 I’s are alike, 2 N’s are alike and rest are different.
∴ The number of required arrangements = \(\frac{11 !}{2 ! 2 ! 2 !}\).

Question 39.
If the letters of the word AJANTA are permuted in all possible ways and the words thus formed are arranged in dictionary order, find the ranks of the words
(i) AJANTA
(ii) JANATA.
Solution:
The alphabetical order of the letters of the given word is AAAJNT.

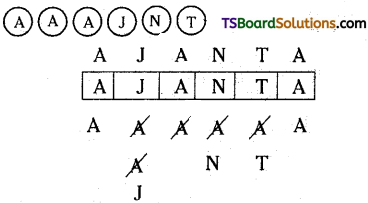
The no. of words begin with AA is 4! = 24
The no. of words begin with AJAA is 2! = 2
The no. of words begin with AJANA is 1! = 1
The next word is AJANTA = 1
Rank of the word, AJANTA = 24 + 2 + 2 + 1 + 1 = 28.

ii) The alphabetical order of the letters of the given word is AAAJNT.

The no. of words begin with A is \(\frac{5 !}{2 !}\) = 60
The no. of words begin with JAA is 3! = 6
The no. of words begin with JANAA is 1! = 1
The next word ¡s JANATA = I
∴ Rank of the word, JANATA = 60 + 6 + 1 + 1 = 68.

Question 40.
Find the number of ways of forming a committee of 4 members out of 6 boys and 4 girls such that there is atleast one girl in the committee.
Solution:

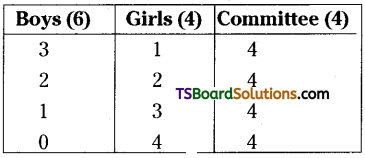
Since, the committee must contain at least one girl, the numbers of committee will be as follows:
i) 3 boys and one girl :
The number of ways of selecting committee = \({ }^6 \mathrm{C}_3 \cdot{ }^4 \mathrm{C}_1=\frac{6 \cdot 5 \cdot 4}{3 \cdot 2 \cdot 1} \cdot 4\) = 80.

ii) 2 boys and 2 girls :
The number of ways of selecting committee = \({ }^6 \mathrm{C}_2 \cdot{ }^4 \mathrm{C}_2=\frac{6 \cdot 5}{2 \cdot 1} \cdot \frac{4 \cdot 3}{2 \cdot 1}\) = 90.

iii) One boy and 3 girls :
The number of ways of selecting committee = \({ }^6 \mathrm{C}_1 \cdot{ }^4 \mathrm{C}_3\) = 6 . 4 = 24

iv) 0 boys and 4 girls: The number of ways of selecting committee = \({ }^6 \mathrm{C}_0 \cdot{ }^4 \mathrm{C}_4\) = 1
∴ The required number of ways of selecting a committee is = 80 + 90 + 24 + 1 = 195.

Question 41.
Find the number of ways of selecting 11 member cricket team from 7 batsmen, 6 bowlers and 2 wicket keepers so that the team contains 2 wicket keepers and atleast 4 bowlers. [March ‘14]
Solution:

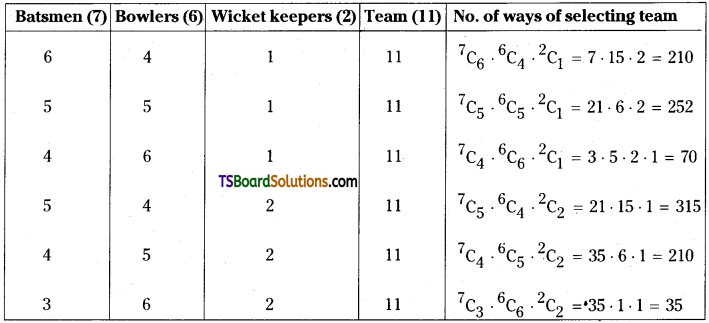
The number of ways of selecting the required cricket team = 210 + 252 + 70 + 315 + 210 + 35 = 1092.

Question 42.
If 2 ≤ r ≤ n, then show that \({ }^n C_{(r-1)}+2 \cdot{ }^n C_{(r-1)}+{ }^n C_r={ }^{(n+2)} C_r\).
Solution:
L.H.S:
\({ }^n C_{(r-1)}+2 \cdot{ }^n C_{(r-1)}+{ }^n C_r\)
= \({ }^n C_{r-2}+{ }^n C_{r-1}+{ }^n C_{r-1}+{ }^n C_r\)
= \({ }^{(n+1)} C_{r-1}+{ }^{(n-1)} C_r={ }^{(n+2)} C_r\) = R.H.S

Question 43.
14 persons are seated at a round table. Find the number of ways of selecting two persons out of them who are not seated
adjacent to each other. [AP – May 2015]
Solution:

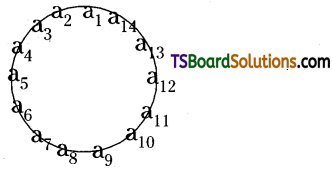
14 persons at the round table.
Selecting 2 persons from 14 persons to be seated on a circular table = \({ }^{14} C_2=\frac{14 \cdot 13}{1 \cdot 2}\) = 91 ways.
The number of ways of selecting 2 adjacent persons 14 (a₁a₂, a₂a₃, a₃a₄ ………………….. a₁₄a₁)
∴ The number of required selections = 91 – 14 = 77.

Question 44.
Find the number of ways of selecting a committee of 6 members out of 10 members always including a specified number.
Solution:
Since, a specified member always includes in a committee.
Remaining 5 members can be selected from 9 members in \({ }^9 \mathrm{C}_5\) ways.
∴ Required number of ways of selecting a committee C = \({ }^9 \mathrm{C}_5=\frac{9 \cdot 8 \cdot 7 \cdot 6 \cdot 5}{5 \cdot 4 \cdot 3 \cdot 2 \cdot 1}\) = 126.

Question 45.
If a set A has 12 elements, find the num¬ber of subsets of A having
i) 4 elements
ii) Atleast 3 elements
iii) Atmost 3 elements.
Solution:
Number of elements in set A = 12
i) Number of subsets of A with exactly four elements = \({ }^{{ }^n} \mathrm{C}_{\mathrm{r}}={ }^{12} \mathrm{C}_4\)
= \(\frac{12 \cdot 11 \cdot 10 \cdot 9}{4 \cdot 3 \cdot 2 \cdot 1}\) = 495.

ii) Number of subsets of A with exactly zero elements is \({ }^{12} \mathrm{C}_0\).
Number of subsets of A with exactly one elements is \({ }^{12} \mathrm{C}_1\).
Number of subsets of A with exactly two elements is \({ }^{12} \mathrm{C}_2\).
Total number of subsets of A formed = 2ⁿ = 2¹².
Number of subsets of A with atleast 3 elements = Total number of subsets – number of subsets contains 0 or 1 or 2 elements.
= 2¹² – [latex]{ }^{12} \mathrm{C}_0+{ }^{12} \mathrm{C}_1+{ }^{12} \mathrm{C}_2[/latex]
= 4096 – 1 – 12 – \(\frac{12 \cdot 11}{2 \cdot 1}\)
= 4096 – 1 – 12 – 66
= 4096 – 79 = 4017.

iii) The required subsets contain atmost 3 elements i.e., it may contain 0 or 1 or 2 or 3 elements.
Number of subsets of A with exactly one element is \({ }^{12} \mathrm{C}_1\).
Number of subsets of A with exactly two elements is \({ }^{12} \mathrm{C}_2\).
Number of subsets of A with exactly three elements is \({ }^{12} \mathrm{C}_3\).
:. Number of subsets of A with atmost 3 elements = \({ }^{12} \mathrm{C}_0+{ }^{12} \mathrm{C}_1+{ }^{12} \mathrm{C}_2+{ }^{12} \mathrm{C}_3\)
= 1 + 12 + 66 + \(\frac{12 \cdot 11 \cdot 10}{3 \cdot 2 \cdot 1}\)
= 79 + 220 = 299

Question 46.
In 5 vowels and 6 consonants are given, then how many 6 letter words can be formed with 3 vowels and 3 consonants.
Solution:
Given 5 vowels and 6 consonants.
6 letter word is formed with 3 vowels and 3 consonants.
Number of ways of selecting 3 vowels from 5 vowels is \({ }^5 \mathrm{C}_3\).
Number of ways of selecting 3 consonants is from 6 consonants is \({ }^6 \mathrm{C}_3\).
∴ Total number of ways of selecting = \({ }^5 \mathrm{C}_3 \times{ }^6 \mathrm{C}_3\)
These letters can be arranged themselves in 6! ways.
∴ Number of 6 letter words formed = \({ }^5 \mathrm{C}_3 \times{ }^6 \mathrm{C}_3\) × 6!.

Question 47.
A question paper is divided into 3 sections A, B, C containing 3, 4, 5 questions respectively. Find the number of ways of attempting 6 questions choosing atleast one from each section.
Solution:
A question paper contains 3 sections A, B, C containing 3, 4, 5 questions respectively.
Number of ways of selecting 6 questions out of these 12 questions = \({ }^{12} \mathrm{C}_6\)
Number of ways of selecting 6 questions from sections B and C (i.e., from 9 questions) = \({ }^9 \mathrm{C}_6\)
Number of ways of selecting 6 questions from sections A and C (i.e., from 8 questions) = \({ }^8 \mathrm{C}_6\)
Number of ways of selecting 6 questions from sections A and B (i.e., 7 questions) = \({ }^7 \mathrm{C}_6\)
∴ Number of ways of selecting 6 questions choosing atleast one from each section = \({ }^{12} \mathrm{C}_6-{ }^7 \mathrm{C}_6-{ }^8 \mathrm{C}_6-{ }^9 \mathrm{C}_6\) = 805.

Question 48.
Find the sum of all 4-digit numbers that can be formed using the digits 0, 2, 4, 7, 8 without repetition.
Solution:
The number of 4 digited numbers formed by the using the digits 0, 2, 4, 7 8 = \(\) (∵ 0 is present).
= 5 × 4 × 3 × 2 – 4 × 3 × 2
= 120 – 24 = 96
The sum of r-digited numbers formed with n distinct non-zero digits (when ’0′ is present).
= \({ }^{(n-1)} P_{(r-1)}\) × (Sum of ’n’ digits) × (111 …………….. 1)_{r times} – \({ }^{(n-2)} \mathrm{P}_{(\mathrm{r}-2)}\) × (Sum of n digits) × (111 …………. 1)_{r – 1 times}
= \((5-1)_{P_{(4-1)}}\) × (0 + 2 + 4 + 7 + 8) × 1111 – \((5-2)_{P_{(4-2)}}\) × (0 + 2 + 4 + 7 + 8) × 111
= \({ }^4 \mathrm{P}_3\) × 21 × 1111 – \({ }^3 \mathrm{P}_2\) × 21 × 111
= 24 × 21 × 1111 – 6 × 21 × 111 = 5,45,958.

Here students can locate TS Inter 1st Year Botany Notes 12th Lesson Histology and Anatomy of Flowering Plants to prepare for their exam.

TS Inter 1st Year Botany Notes 12th Lesson Histology and Anatomy of Flowering Plants

→ Study of different tissues in the plant body is called Histology.

→ Study of internal structures (arrangement of various tissues) of plants is called Anatomy.

→ Different organs in a plant show differences in their internal structure.

→ Internal structures also show adaptations to diverse environments.

→ Katherine Esau

• Katherine Esau was horn in Ukraine in 1898.
• She reported in her early publications that the curly top virus spreads through a plant via the food conducting or phloem tissue.
• Dr Esau’s Plant Anatomy published in 1954 took a dynamic, developmental approach designed to enhance one’s understanding of plant structure and had enormous impact world wide, literally bringing about a revival of the discipline. The Anatomy of seed plants by Katherine Esau was published in 1960. It was referred to as Webster’s of plant biology – it is encyclopediac.

→ Internal Morphology deals with the study of internal structure of different plant organs. It has two branches they are Histology and Anatomy.

→ Histology is the study of different tissues present in the plant body.

→ Anatomy deals with the study of gross internal details of plant organs like root, stem, leaf, flower, etc.

→ Tissues are functional units of an organ. Tissues are groups of cells having similar, function and origin.

→ Meristems are localised growth regions of the plant producing,new tissues and organs throughout its life period. Based on origin – 2 types – primary meristem, secondary meristem. Based on position – 3 types – apical, intercalary and lateral meristems.

→ Permanent tissues have mature cells adapted to specific function.

→ Simple tissues have similar kinds of cells.

→ Parenchyma is a fundamental living tissue with intercellular spaces.

→ Collenchyma is a simple living mechanical tissue.

→ Sclerenchyma is a simple dead mechanical tissue with lignified walls.

→ Tissue systems are three types. They are – epidermal, ground and vascular.

→ The epidermal tissue systems are made up of epidermal cells, stomata and the epidermal appendages.

→ The ground tissue system forms the main bulk of the plant. It is divided into three zones- cortex, pericycle and pith.

→ The vascular tissue system is formed by the xylem and phloem.

→ The general plant internal structure is similar in both dicot and monocot roots.

→ Root TS shows 3 regions – epidermis, cortex and stele.

→ Exodermis contains suberised cells prevents leakage of water.

→ General cortex is made up of parenchyma.

→ Epidermis is outermost layer with unicellular root hairs. Cuticle & stomata are

→ Exodermis shows casparian bands.

→ Stele consists of pericycle, vascular bundles, conjunctive tissues and pith.

→ Pericycle is a single layered parenchymatous cells. Pericycle gives lateral roots.

→ Vascular bundles are separate, radial, alternate and closed. Xylem is exarch.

→ Stele is monarch to octarch, generally tetrarch in dicot root and polyarch in monocot root.

→ Pith is either scanty or absent in dicot root. It is large and well developed in monocot root.

→ Conjunctive tissue is present between xylem and phloem strands.

→ A typical dicot stem consists of epidermis, cortex and stele. A typical monocot stem shows epidermis, hypodermis, ground tissue and vascular bundles.

→ Epidermis is a uniseriate protective layer covered with cuticle. Stomata help in exchange of gases.

→ Hypodermis is collenchymatous in dicot stem and sclerenchymatous in monocot stem.

→ General cortex in dicot stem and ground tissue in monocot stem are parenchymatous.

→ Endodermis is distinct only in dicot stem with casparian strips.

→ Stele is eustele in dicot stem and atactostele in monocot stem.

→ Vascular bundles are few and arranged in a ring inside pericycle in dicot stem. They are numerous and scattered in the ground tissue in monocot stem.

→ Vascular bundles are conjoint, collateral and endarch They are open in dicot stem and closed in monocot stem.

→ Distinct pith and medullary rays are present in dicot stem.

→ Dicot leaf is dorsiventral and monocot leaf is isobilateral.

→ Epidermis covers adaxial and abaxial surfaces of the leaf. Cuticle and stomata are present.

→ Mesophyll is differentiated into upper palisade and lower spongy tissues in dorsiventral leaf. It is undifferentiated and homogeneous in isobilateral leaf.

→ Vascular bundles are collateral and closed. Xylem is on upper side and phloem on lower side. Vascular bundle is covered with bundle sheath.

→ In Monocot leaf upper epidermis shows bulliform or motor cells.

→ Secondary growth results in increase in girth by addition of secondary tissues.

→ Interfascicular and intrafascicular cambia together form vascular cambium.

→ Vascular cambium forms secondary xylem inside and secondary phloem on the exterior.

→ Cork cambium develops from cortical region. It produces cork towards outside and secondary cortex on the inner side.

→ Secondary xylem or wood forms the bulk of tree. Production of secondary xylem is affected by seasonal change.

→ Autumn wood and spring wood formed in one year together constitute annual ring.

→ Wood in the centre of the tree ceases to perform the function of conduction and is blocked by gums, resins, tyloses etc. It is called heartwood. Outer lighter sapwood is functional wood.

→ Cork is a dead tissue containing suberised cells.

→ Phellogen (cork cambium), phellem (cork) and phelloderm (secondary cortex) constitute periderm.

→ Lenticels are lens shaped openings found in the cork for gaseous exchange.

→ All tissues outside the vascular cambium constitute bark.

Here students can locate TS Inter 2nd Year Physics Notes 7th Lesson Moving Charges and Magnetism to prepare for their exam.

TS Inter 2nd Year Physics Notes 7th Lesson Moving Charges and Magnetism

→ Conclusion of Oersted states that moving charges produce a current and magnetic field in the surrounding space.

→ If current carrying wire is perpendicular to the plane of the paper then magnetic field lines produced are concentric circles in the plane of the paper with the conductor at centre.

→ If direction of current in a conductor is reversed then direction of magnetic field is also reversed.

→ A static charge will produce only electric field whereas a moving charge will produce both magnetic field and electric field.

→ Lorentz force: If a charge q is moving with a velocity V in electric (E̅) and magnetic fields (B) then total force on it is the sum of force due to electric field (F_ele) and force due to magnetic field (F_mag) at that given point say V.
F = q [ E(r) + v̅ × B̅ (r)] = F_ele + F_mag
This is known as Lorentz force.

→ Force acting on a current carrying conductor placed in a magnetic field F = BIf sin θ

→ Motion of a charged particle in a magnetic field : if a charged particle q’ is moving perpendicularly in a magnetic field B̅ with a velocity ‘v’ then force due to magnetic field is always perpendicular to velocity V. So it describes a circular path.
Since force and displacement are perpendicular no work is done.

→ For a charged particle moving perpendicularly in a magnetic field mv²/r = qvB. or radius of circular/ helical path r = \(\frac{m v}{q B}\)

→ Velocity selector : If a charged particle is moving in a crossed electric (E̅) and magnetic fields (B̅) such that they will cancel each other then path of charged particle is undeviated, i.e., when qE = qvB
⇒ v = \(\frac{E}{B}\)
The ratio of \(\frac{E}{B}\) for undeviation condition B is called velocity selector.

→ Cyclotron : Cyclotron is a charged particle accelerator.
Cyclotron frequency υ_c = \(\frac{\mathrm{qB}}{2 \pi \mathrm{m}}\) is independent of velocity of charged particle.

→ From Biot – Savart’s law magnetic field due to a current carrying conductor at a given point P’ is given by dB = \(\frac{\mu_0}{4 \pi} \frac{I d l \sin \theta}{\mathbf{r}^2}\)

→ From Ampere’s circuital law the total mag¬netic field coming out of a current carrying conductor is p0 times greater than the cur¬rent flowing through it.
∮B. dI = µ₀I

→ Solenoid : A solenoid consists of a long wire wound on an insulating hollow cylinder in the form of helix.
Net magnetic field is the vector sum of fields due to all turns.
Magnetic field along the axis of solenoid B= n0nI

→ Toroid : Toroid is a coiled coil.
It consists of a large number of turns of wire which are closely wound on a ring. Or Toroid is a solenoid bent into the form of a ring.
In a toroid magnetic field B = \(\frac{\mu_0 \mathrm{NI}}{2 \pi \mathrm{r}}\)
Where N = 2πrn = perimeter of toroid × number of turns per unit length.
Current loop:

→ Torque on a current loop τ = IAB sin θ
For a loop of n turns torque τ = n IAB sin θ
Here sin θ is the angle between Area vector A̅ and direction of magnetic field B̅.

→ Magnetic moment of current loop M̅ = IA̅; OR nIA̅
Torque τ = M̅ x B̅

→ For a revolving electron : Current I = e/T
Magnetic moment μ₁ = \(\frac{\mathrm{e}}{2 \mathrm{~m}_{\mathrm{e}}}\)(m_evr) = \(\frac{\mathrm{e}}{2 \mathrm{~m}_{\mathrm{e}}}\)I ;
Time period T = \(\frac{2 \pi r}{v}\)
Gyromagnetic ratio \(\frac{\mathrm{e}}{2 \mathrm{~m}_{\mathrm{e}}}\)
Angular momentum I = \(\frac{\mathrm{nh}}{2 \pi}\)

→ Moving Coil Galvanometer (M.C.G) :
Torque on the coil τ = nlAB deflection Φ = \(\left(\frac{\mathrm{NAB}}{\mathbf{k}}\right)\)I, k = torsional constant of spring.

→ To convert galvanometer into ammeter shunt to be added R_s = \(\frac{G}{n-1}\).
Where n = \(\left(\frac{i}{i_g}\right)=\frac{\text { new current }}{\text { old current }}\)
= \(\frac{\text { current to be measured }}{\text { current permitted through galvanometer }}\)

→ To convert galvanome fer into voltmeter series resistance to be added R_s = \(\frac{\mathrm{V}}{\mathrm{I}_{\mathrm{g}}}\) – R_G
Where I_g = Current permitted through galvanometer
V = Voltage to be measured.
R_G = Resistance of galvanometer.

Telangana TSBIE TS Inter 1st Year Political Science Study Material 10th Lesson Constitution Textbook Questions and Answers.

TS Inter 1st Year Political Science Study Material 10th Lesson Constitution

Long Answer Questions

Question 1.
Define Constitution and explain the features of Constitution.
Answer:
Introduction :
The age of Democracy led to political civilisation. Now-a-days every civilised state possess a constitution. A Constitution is a condition of modem state. The constitution is a living text of a political system. It represents the political character of the state and its constituents.

The term constitution implies a written document embodying the provisions relating to the powers and functions of the government organs, the rights and duties of the citizens.

Meaning :
The term constitution is an English word. It was derived from a Latin word “Constitution, which means to Establish”.

Definitions:
1) Aristotle :
“Constitution is the arrangement of offices in a state, especially the highest of all”.

2) Lord Bryce :
“Constitution is a set of established rules embodying and enacting the practice of Government”.

3) Stephen Leacock :
“Constitution is the form of Government”.

4) K.C. Wheare :
“Constitution is that body of rules which regulates the ends for which governmental power is exercised”.

Features of the Constitution :
1) Preamble :
Every Constitution will have a preamble. The preamble denotes the aims and aspirations of the Constitution. It is like the soul of the Constitution. Hence, preamble is considered as an important feature of the Constitution.

2) Clarity :
Clarity is another important feature of the Constitution. The Constitution clearly explains about the different policies and methods of governance. It is written in a simple and clear language.

3) Incorporation of Fundamental Rights :
Every Constitution includes some fundamental rights. These fundamental rights are meant for safeguarding the freedoms of the citizens. They enable the citizens to realise their personality in various spheres. They , help the citizens for leading a happy and honorable life in the state.

4) Brevity :
Brevity is another feature of a Constitution. Brevity avoids confusion among the individuals in understanding and interpreting provisions. Unnecessary elements are not included in the Constitution. It should be precise. It must not contain large number of clauses.

5) Flexibility :
The Constitution must be flexible for adapting the wishes are aspirations of the people from time to time. There must be a scope of amending the provisions of the Constitution if necessary. Frequent changes in the Constitution tend to weaken the spirit of the Constitution. But, at the same time, the Constitution of a modem state should be adaptable to the progressive changes.

6) Permanence :
Permanence is one more feature of the Constitution. The Constitution must have everlasting values for the welfare of the whole nation. It represents the actual structure of the state and its political institutions. It obliges the customs of the people.

7) Mode of Amendment :
The Constitution specifies the mode of amendment. It will be relevant to the contemporary conditions of the state. It contains a special chapter on the constitutional amendment procedures. Usually the constitutional amendments are of three types, namely (i) Rigid (ii) Flexible and (iii) Half rigid and Half flexible. On the whole, the constitution of every state comprises both rigid and flexible elements.

8) Explanatory :
The Constitution is explanatory in nature. It denotes and discusses almost all elements relating to the People, Government and State. It contains separate provisions on the structure, powers and limitations of state activity.

Question 2.
Define Constitution and point out the differences between Flexible and Rigid Constitutions.
Answer:
Definition :
The age of Democracy led to political civilisation. Now-a-days every civilised state possess a Constitution. A Constitution is a condition of modern state. The constitution is a living text of a political system. It represents the political character of the state and its constituents.

Flexible Constitution :
Flexible constitution is one whose provisions can be amended easily. It requires no special procedure for changing its provisions. It can be amended by the authorities by adopting the same procedure of ordinary laws. So we do not find differences between ordinary and constitutional laws. Flexible constitutions were prevalent in the ancient period. Ex : British constitution.

Rigid Constitution :
Rigid constitution is one whose provisions cannot be changed easily. In this system the constitutional amendment methods are different from those of ordinary laws. There will be a special procedure for amending the provisions of the rigid constitution. The rigid constitution will have firmness due to its special procedures of amendment. Ex : United States.

Differences between Flexible and Rigid Constitution

Flexible Constitution	Rigid Constitution
1. Constitutional matters are not clearly mentioned.	1. Constitutional matters are clearly written.
2. Not appropriate to a federal state.	2. Appropriate for a federal state.
3. Highly unstable.	3. Highly stable.
4. Constitution can be easily amended.	4. Constitution cannot be easily amended.
5. Provides no scope for judicial review.	5. Provides scope for judicial review.
6. Only one type of law is found.	6. Two types of laws are found, constitutional and ordinary. Constitutional laws precede ordinary laws.
7. Rights, freedoms and liberties of people may not be safeguarded by the Judiciary.	7. Rights, freedoms and liberties of people will be better safeguarded by the Judiciary.
8. No scope for revolutions.	8. Scope for revolutions.
9. Possibility of unlimited legislative power.	9. Possibility of a limited legislative power.
10. More suitable to the politically advanced states.	10. More suitable to the developing nations.
11. It makes no differentiation between constitutional and ordinary laws.	11. It makes differentiation between constitutional and ordinary laws.
12. Appropriate to small states.	12. Appropriate to large states.

 

Short Answer Questions

Question 1.
Define Constitution. Explain its features.
Answer:
Features of the Constitution :
1) Preamble :
Every Constitution will have a preamble. The preamble denotes the aims and aspirations of the Constitution. It is like the soul of the Constitution. Hence, preamble is considered as an important feature of the Constitution.

2) Clarity :
Clarity is another important feature of the Constitution. The Constitution clearly explains about the different policies and methods of governance. It is written in a simple and clear language.

3) Incorporation of Fundamental Rights :
Every Constitution includes some fundamental rights. These fundamental rights are meant for safeguarding the freedoms of the citizens. They enable the citizens to realise their personality in various spheres. They help the citizens for leading a happy and honourable life in the state.

4) Brevity :
Brevity is another feature of a Constitution. Brevity* avoids confusion among the individuals in understanding and interpreting provisions. Unnecessary elements are not included in the Constitution. It should be precise. It must not contain large number of clauses.

5) Flexibility :
The Constitution must be flexible for adapting the wishes are aspirations of the people from time to time. There must be a scope of amending the provisions of the Constitution if necessary. Frequent changes in the Constitution tend to weaken the spirit of the Constitution. But, at the same time, the Constitution of a modem state should be adaptable to the progressive changes.

6) Permanence :
Permanence is one more feature of the Constitution. The Constitution must have everlasting values for the welfare of the whole nation. It represents the actual structure of the state and its political institutions. It obliges the customs of the people.

Question 2.
What are the merits and demerits of a Written Constitution?
Answer:
Written Constitution :
A written constitution is formulated and adopted by a Constituent Assembly or a Convention. It comprises several principles and rules of the Government in a written form or document. The Constitution of India is an example of written constitution. The American Constitution is the first written constitution in the world.

Merits:

1. A written constitution carries more simplicity. It gives no scope for confusion and ambiguity among the people in understanding the structure and organization of various institutions. .
2. It protects the fundamental rights of the people.
3. It puts limitations on the powers of the Government.
4. It renders political stability due to its rigid nature.
5. It embodies the aspirations of the people. It cautions the Government about the importance of the accomplishment of popular needs.
6. It maintains equilibrium between the centre and the states by allocating powers in a judicious manner.
7. It safeguards the sanctity and spirit of a .federation.

Demerits:

1. A written constitution cannot provide a better Government as it impose some stipulated conditions on the party in power.
2. It makes the judiciary a predominant one.
3. Its provisions cannot be changed according to the needs and wishes of the people. So, the progress of the nations lags behind.
4. Its rigid nature is not helpful to the development of the state.
5. It gives scope for conflicts among the governmental organs.
6. It may not be conductive to the formation of a welfare state.

Question 3.
Explain the merits and demerits of Unwritten Constitution.
Answer:
Unwritten Constitution: Unwritten constitution is one whose provisions are not written in a single document. It includes several customs and traditions which are manifested in the form of the laws.

The Constitution of Britain is the best example of unwritten constitution.

Merits:

1. An unwritten constitution paves the way for progressive legislation. It has develop-ment orientation.
2. It always undergoes the process of evolution as it aims at ‘bettering the best’.
3. It gives no scope for revolutions and such other agitations. It concedes to the popular demands.
4. It can be amended according to the popular needs and aspirations.
5. Its provisions are elastic in nature. So, changes in the constitution are easily made.

Demerits :

1. An unwritten constitution may be changed frequently by the party in power for its political gains. This affects the political stability of the nation.
2. It fails to protect the rights and freedom of people.
3. It is more informal in nature.
4. It is also not suitable for federal states.
5. An unwritten constitution is considered as a play tool of judges. This may lead to judicial manipulations.
6. It is prone to frequent amendments.
7. It is not suitable to democratic states.

Question 4.
Distinguish between Written and Unwritten Constitution. [A.P. Mar. 16]
(or)
5. Explain the difference between Written and Unwritten Constitution.
Answer:
Written Constitution :
A written constitution is formulated and adopted by a Constituent Assembly or a Convention. It comprises several principles and rules of the Government in a written form or document. The Constitution of India is an example of written constitution. The American Constitution is the first written constitution in the world.

Unwritten Constitution :
Unwritten constitution is one whose provisions are not written in a single document. It includes several customs and traditions which are manifested in the form of the laws. The Constitution of Britain is the best example of unwritten constitution.

Differences between Written and Unwritten Constitutions

Written Constitution	Unwritten Constitution
1. Written constitution implies a document or few documents in which the rules regulating the main institutions of Government are written down.	1. Unwritten constitution denotes a sum of customs, conventions and usages which have not been systematically documented.
2. All the basic principles of the State are clearly written.	2. All the basic principles of the State exist in the form of customs and traditions.
3. Written constitution is framed by a special assembly convened at a particular point of time.	3. Unwritten constitution contains some written elements also in the form of enactments of fundamental charters made from time to time.
4. It is suitable to the educated and literate people.	4. It is suitable to the uneducated and illiterate people.
5. Courts of law protect the liberties of the citizens.	5. Courts of law cannot provide much protection.
6. It is formulated at a particular time.	6. It is evolutionary in nature.
7. It provides political stability.	7. It could not ensure political stability.
8. It cannot be easily amended.	8. It can easily be amended.
9. It is useful to federal states.	9. It is advantageous to the unitary states.

 

Very Short Answer Questions

Question 1.
What do you mean by Constitution?
Answer:
The term constitution implies a written document embodying the provisions relating to the powers and functions of the Government organs, the rights and duties of the citizens.

Question 2.
What is an Unwritten Constitution?
Answer:
An unwritten constitution is one whose provisions are not written in a single document. It includes several customs and traditions which are manifested in the form of Laws. The constitution of Britain is the best example of an unwritten constitution.

Question 3.
What is a Written Constitution?
Answer:
A written constitution is formulated and adopted by a constituent Assembly. It comprises several principles and rules of the government in a written form or document. The constitution of India is an example of written constitution. The American constitution is the first written constitution in the world.

Question 4.
Write is Flexible Constitution.
Answer:
Flexible constitution is one whose provisions can be amended easily. It requires no special procedure for changing its provisions.

It can be amended by the authorities by adopting the same procedure of ordinary laws. So we do not find differences between ordinary and constitutional laws. Flexible constitutions were prevalent in the ancient period.
Ex.: British Constitution.

Question 5.
What is Rigid Constitution?
Answer:
Rigid Constitution is one whose provisions can be changed easily. In this system, the constitutional amendment methods are different from those of ordinary laws. There will be a special procedure for amending the provisions of the Rigid Constitution. The Rigid Constitution will have firmness due to its special procedures of the amendment.
Ex.: Constitution of United States of America.

Question 6.
What is the preamble?
Answer:
Every Constitution will have a preamble. The preamble denotes the aims and aspirations of the constitution. It is the soul of the constitution. Hence, the preamble is considered one of the important features of the Constitution.